Which of the following are models for perpendicular lines :(a) The adjacent edges of a table top.(b) The lines of a railway track.(c) The line segments forming the letter ‘L’.(d) The letter V.
To determine which of the given options are models for perpendicular lines, we will analyze each option one by one.
1. Analyze Part (a): The adjacent edges of a table top.
- The adjacent edges of a table top meet at a right angle (90 degrees). Therefore, they are perpendicular to each other.
- Conclusion: This is a model for perpendicular lines.
2. Analyze Part (b): The lines of a railway track.
- The lines of a railway track run parallel to each other and do not intersect. Since they do not meet at any angle, they cannot be perpendicular.
- Conclusion: This is not a model for perpendicular lines.
3. Analyze Part (c): The line segments forming the letter ‘L’.
- The letter 'L' consists of two line segments that meet at a right angle (90 degrees). Therefore, they are perpendicular to each other.
- Conclusion: This is a model for perpendicular lines.
4. Analyze Part (d): The letter V.
- The letter 'V' consists of two line segments that meet at an angle. However, this angle is not 90 degrees, so they are not perpendicular.
- Conclusion: This is not a model for perpendicular lines.
Final Answer:
The models for perpendicular lines are:
- Part (a): The adjacent edges of a table top.
- Part (c): The line segments forming the letter ‘L’.
- The adjacent edges of a table top meet at a right angle (90 degrees). Therefore, they are perpendicular to each other.
- Conclusion: This is a model for perpendicular lines.
- The lines of a railway track run parallel to each other and do not intersect. Since they do not meet at any angle, they cannot be perpendicular.
- Conclusion: This is not a model for perpendicular lines.
- The letter 'L' consists of two line segments that meet at a right angle (90 degrees). Therefore, they are perpendicular to each other.
- Conclusion: This is a model for perpendicular lines.
- The letter 'V' consists of two line segments that meet at an angle. However, this angle is not 90 degrees, so they are not perpendicular.
- Conclusion: This is not a model for perpendicular lines.
The models for perpendicular lines are:
- Part (a): The adjacent edges of a table top.
- Part (c): The line segments forming the letter ‘L’.
Topper's Solved these Questions
Similar Questions
.Knowledge Check
- Question 1 - Select One
Which of the following is an equivalent of a line that is perpendicular to the line y=−2(x+1)?
Ax+2y=7B8x−4y=9Cx−16=y3Dy−2x=0
Identify rays and line segments form the following figures:
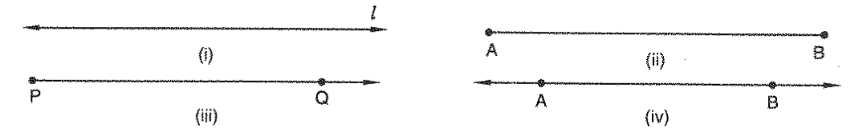View SolutionState which of the following statements are true and which are false:
The letter Z has one line of symmetry.View SolutionGive a definition for each of the following terms.
(a) parallel lines
(b)perpendicular lines
(c) radius of a circle
(d) squareView SolutionWhich of the following has a definite length? A. a ray B. a line segment C. a line D. none of these
View SolutionA straight line L is perpendicular to the line 5x−y=1. The area of the triangle formed by the line L and the coordinate axes is 5'□units.F∈dtheequationofthel∈eL
View SolutionStudy the diagram. The line l is perpendicular to line m (a) Is CE=EG (b) Does PE bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) AC>EG (ii) CD=GH (iii) BC<EH
View SolutionA straight line segment of length/moves with its ends on two mutually perpendicular lines. Find the locus of the point which divides the line segment in the ratio 1:2
View Solution
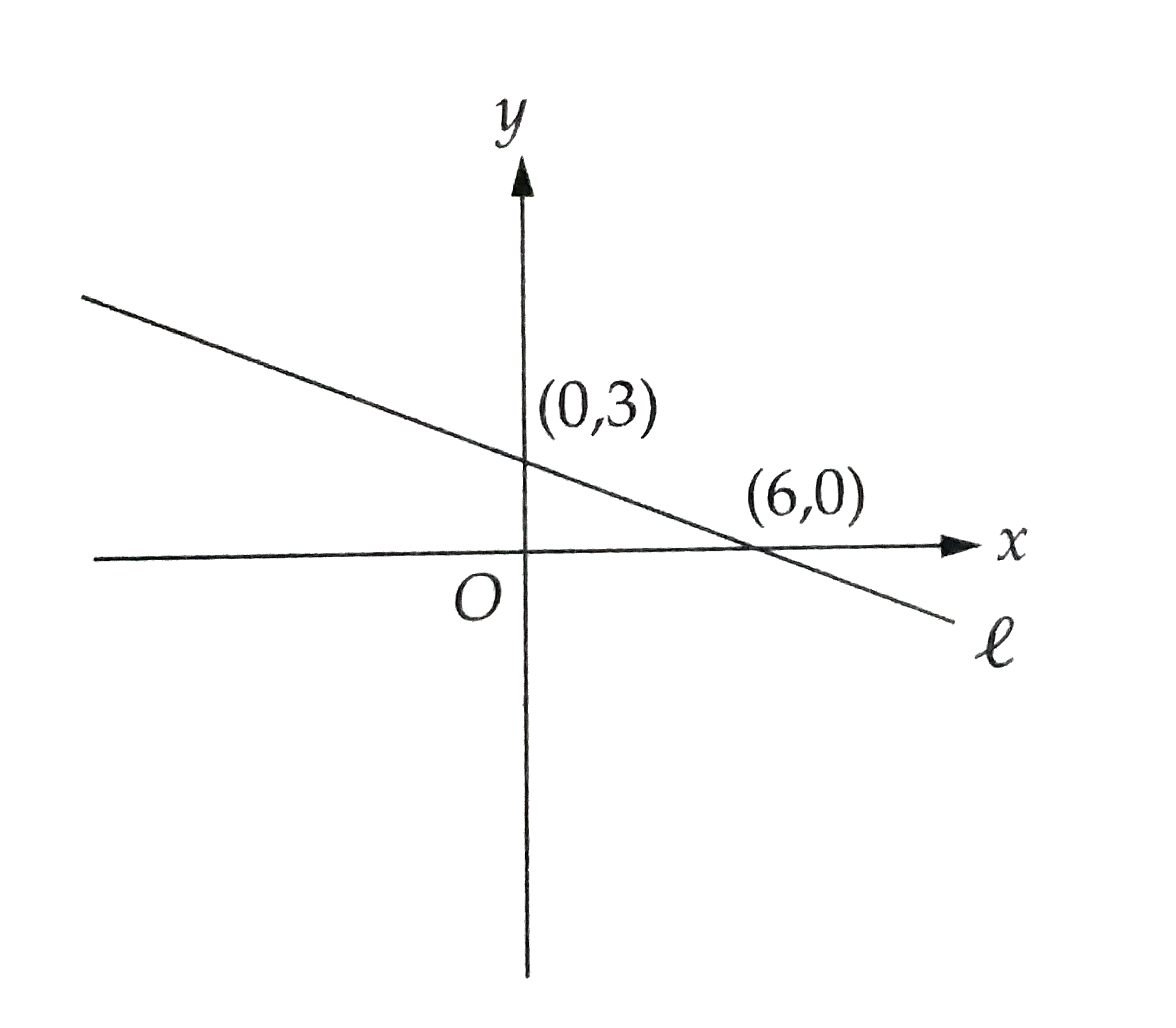