Which of the following pairs represent equivalent rational numbers ?
14and−4−16
−23and812
1215and1018
2754and32
The correct Answer is:A
To determine which pairs represent equivalent rational numbers, we will analyze each option by converting the fractions to their lowest forms and checking for equality.
1. Identify the pairs of rational numbers:
- Option A: 14 and −4−16
- Option B: −23 and 812
- Option C: 1215 and 1018
- Option D: 2754 and 32
2. Analyze Option A:
- First number: 14 is already in its lowest form.
- Second number: −4−16
- The negatives cancel out: −4−16=416
- Simplifying 416: Divide both numerator and denominator by 4:
4÷416÷4=14
- Since both are 14, they are equivalent.
3. Analyze Option B:
- First number: −23 is already in its lowest form.
- Second number: 812
- Simplifying 812: Divide both by 4:
8÷412÷4=23
- Now we compare −23 and 23. They are not equal due to the negative sign, so they are not equivalent.
4. Analyze Option C:
- First number: 1215
- Simplifying 1215: Divide both by 3:
12÷315÷3=45
- Second number: 1018
- Simplifying 1018: Divide both by 2:
10÷218÷2=59
- Now we compare 45 and 59. They are not equal, so they are not equivalent.
5. Analyze Option D:
- First number: 2754
- Simplifying 2754: Divide both by 27:
27÷2754÷27=12
- Second number: 32 is already in its lowest form.
- Now we compare 12 and 32. They are not equal, so they are not equivalent.
Conclusion:
The only equivalent pair of rational numbers is Option A: 14 and −4−16.
- Option A: 14 and −4−16
- Option B: −23 and 812
- Option C: 1215 and 1018
- Option D: 2754 and 32
- First number: 14 is already in its lowest form.
- Second number: −4−16
- The negatives cancel out: −4−16=416
- Simplifying 416: Divide both numerator and denominator by 4:
4÷416÷4=14
- Since both are 14, they are equivalent.
- First number: −23 is already in its lowest form.
- Second number: 812
- Simplifying 812: Divide both by 4:
8÷412÷4=23
- Now we compare −23 and 23. They are not equal due to the negative sign, so they are not equivalent.
- First number: 1215
- Simplifying 1215: Divide both by 3:
12÷315÷3=45
- Second number: 1018
- Simplifying 1018: Divide both by 2:
10÷218÷2=59
- Now we compare 45 and 59. They are not equal, so they are not equivalent.
- First number: 2754
- Simplifying 2754: Divide both by 27:
27÷2754÷27=12
- Second number: 32 is already in its lowest form.
- Now we compare 12 and 32. They are not equal, so they are not equivalent.
The only equivalent pair of rational numbers is Option A: 14 and −4−16.
Topper's Solved these Questions
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS) (LEVEL-2)15videos
RATIONAL NUMBERS
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - EXERCISE (MATCHING)2videos
RATIONAL NUMBERS
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - NCERT SECTION (EXERCISE -9.2)25videos
RATIONAL NUMBERS
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PREP TEST 2 EXERCISE - SECTION-B30videos
PREP TEST 2
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - SIMPLE EQUATIONSEXERCISE - Olympiad /Hots Corner18videos
SIMPLE EQUATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following pairs represents anomers?
A B
B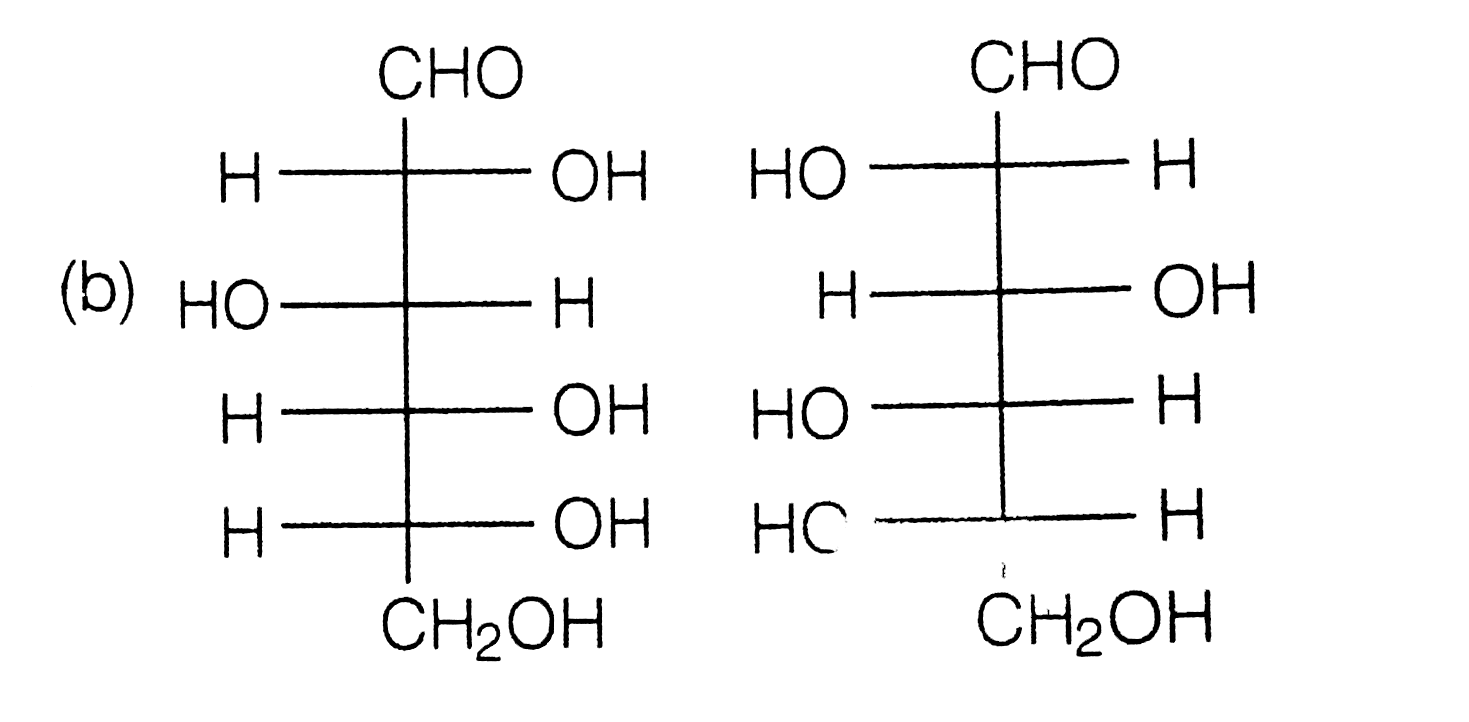 C
C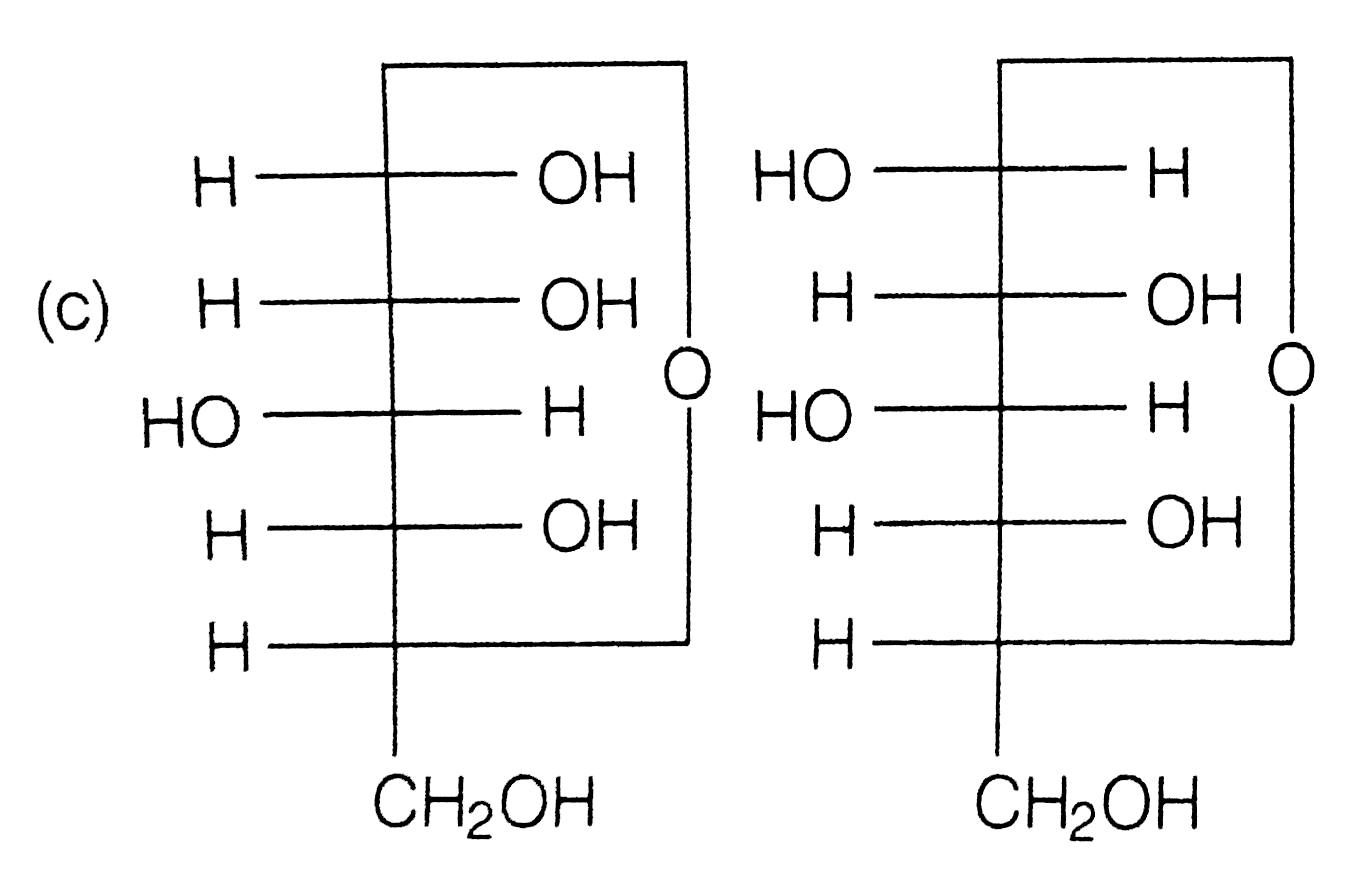 D
D
- Question 2 - Select One
Which of the following pairs represents anmors?
A B
B C
C D
D
- Question 3 - Select One
Which of the following pairs represents isobars?
A.19K40 and .11Na23B.2He3 and .2He4C.12Mg24 and .12Mg25D.19K40 and .20Ca40
If each of the following pairs represents a pair of equivalent rational numbers, find the values of x. 35 andx−25 (ii) 136 and −65x
View SolutionWhich of the following pairs represents anomers ?
View SolutionWhich of the following pairs represents anomers ?
View SolutionWhich of the following pairs represents anomers ?
View SolutionWhich of the following pairs represents anomers ?
View Solution

