Which of the following set is not a convex set?
{(x,y):1≤x2+y2≤3}
{(x,y):x2+y2≤2}
{(x,y):x+y≤1}
{(x,y):2x2+3y2≤6}
The correct Answer is:A
To determine which of the following sets is not a convex set, we first need to understand the definition of a convex set. A set is considered convex if, for any two points within the set, the line segment connecting those two points lies entirely within the set.
1. Understand the Definition of Convex Set:
- A set S is convex if for any two points A and B in S, the line segment joining A and B is also in S.
2. Analyze the Given Options:
- We have four options to evaluate:
- Option A: 1≤x2+y2≤3
- Option B: x2+y2≤2
- Option C: x+y≤1
- Option D: 2x2+3y2≤6
3. Evaluate Each Option:
- Option A: 1≤x2+y2≤3
- This represents the area between two circles: one with radius √1 and the other with radius √3. Points on the inner circle (radius 1) are not included in the set, while points on the outer circle (radius √3) are included. Therefore, if you take two points on the outer circle, the line segment between them may include points that lie inside the inner circle, which are not part of the set. Thus, this set is not convex.
- Option B: x2+y2≤2
- This represents a filled circle with radius √2. Any line segment between two points inside this circle will also lie inside the circle, hence this set is convex.
- Option C: x+y≤1
- This represents a half-plane below the line x+y=1. Any line segment between two points in this half-plane will also lie in the half-plane, making this set convex.
- Option D: 2x2+3y2≤6
- This represents an ellipse. Any line segment between two points inside the ellipse will also lie inside the ellipse, indicating that this set is convex.
4. Conclusion:
- After evaluating all options, we conclude that Option A (1≤x2+y2≤3) is the set that is not convex.
Final Answer:
The set that is not a convex set is Option A: 1≤x2+y2≤3.
- A set S is convex if for any two points A and B in S, the line segment joining A and B is also in S.
- We have four options to evaluate:
- Option A: 1≤x2+y2≤3
- Option B: x2+y2≤2
- Option C: x+y≤1
- Option D: 2x2+3y2≤6
- Option A: 1≤x2+y2≤3
- This represents the area between two circles: one with radius √1 and the other with radius √3. Points on the inner circle (radius 1) are not included in the set, while points on the outer circle (radius √3) are included. Therefore, if you take two points on the outer circle, the line segment between them may include points that lie inside the inner circle, which are not part of the set. Thus, this set is not convex.
- Option B: x2+y2≤2
- This represents a filled circle with radius √2. Any line segment between two points inside this circle will also lie inside the circle, hence this set is convex.
- Option C: x+y≤1
- This represents a half-plane below the line x+y=1. Any line segment between two points in this half-plane will also lie in the half-plane, making this set convex.
- Option D: 2x2+3y2≤6
- This represents an ellipse. Any line segment between two points inside the ellipse will also lie inside the ellipse, indicating that this set is convex.
- After evaluating all options, we conclude that Option A (1≤x2+y2≤3) is the set that is not convex.
The set that is not a convex set is Option A: 1≤x2+y2≤3.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Which of the following sets is not convex?
A{(x,y)∣2x+5y<7}B{(x,y)∣x2+y2≤4}C{x|x∣=5}D{(x,y)∣3x2+2y2≤6}Which of the following is not a convex set ?
A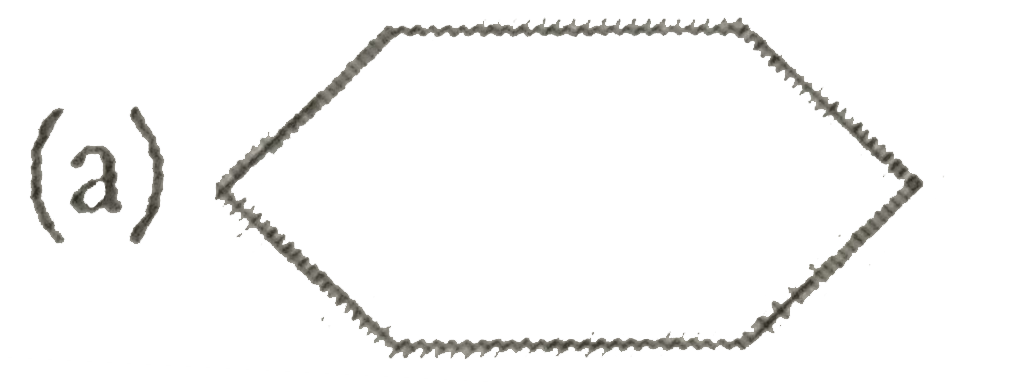 B
B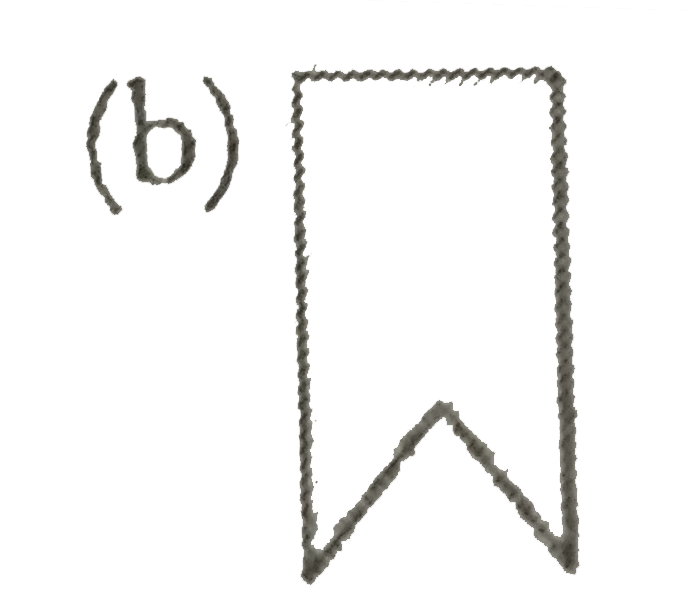 C
C D
D
which of the following is not a convex set?
A{(x,y):2x+2y≤7}B{(x,y):x2+y2≤4}C{x|x∣=5}D{(x,y):2x2+3y2≤6}