Will the median class and modal class of grouped data always by different? Justify your answer.
To determine whether the median class and modal class of grouped data are always different, we can analyze the definitions and characteristics of both classes.
1. Understanding the Definitions:
- Median Class: The median class is the class interval that contains the median of the data. The median is the value that separates the higher half from the lower half of the data.
- Modal Class: The modal class is the class interval that has the highest frequency, meaning it contains the mode of the data.
2. Example of Grouped Data:
Let's consider a hypothetical grouped data with the following class intervals and frequencies:
- Class Interval: 100-120, 120-140, 140-160, 160-180, 180-200
- Frequencies: 12, 14, 8, 6, 10
3. Calculate Total Frequency (N):
- Total frequency (N) = 12 + 14 + 8 + 6 + 10 = 50
4. Finding the Median Class:
- Calculate N/2:
N/2=50/2=25
- Now, we find the cumulative frequency (CF):
- CF for 100-120 = 12
- CF for 120-140 = 12 + 14 = 26
- CF for 140-160 = 26 + 8 = 34
- CF for 160-180 = 34 + 6 = 40
- CF for 180-200 = 40 + 10 = 50
- The median class is the first class where the cumulative frequency is greater than or equal to 25. Here, the median class is 120-140.
5. Finding the Modal Class:
- The modal class is the class with the highest frequency. In our case, the highest frequency is 14, which corresponds to the class interval 120-140.
6. Conclusion:
- In this example, both the median class and modal class are the same (120-140). However, this is not always the case. Depending on the distribution of the data, the median class and modal class can be different. For instance, if the frequencies were altered such that the highest frequency was in a different class, the two classes could differ.
Final Justification:
Thus, we conclude that the median class and modal class of grouped data are not always different; they can be the same depending on the distribution of the data.
- Median Class: The median class is the class interval that contains the median of the data. The median is the value that separates the higher half from the lower half of the data.
- Modal Class: The modal class is the class interval that has the highest frequency, meaning it contains the mode of the data.
Let's consider a hypothetical grouped data with the following class intervals and frequencies:
- Class Interval: 100-120, 120-140, 140-160, 160-180, 180-200
- Frequencies: 12, 14, 8, 6, 10
- Total frequency (N) = 12 + 14 + 8 + 6 + 10 = 50
- Calculate N/2:
N/2=50/2=25
- Now, we find the cumulative frequency (CF):
- CF for 100-120 = 12
- CF for 120-140 = 12 + 14 = 26
- CF for 140-160 = 26 + 8 = 34
- CF for 160-180 = 34 + 6 = 40
- CF for 180-200 = 40 + 10 = 50
- The median class is the first class where the cumulative frequency is greater than or equal to 25. Here, the median class is 120-140.
- The modal class is the class with the highest frequency. In our case, the highest frequency is 14, which corresponds to the class interval 120-140.
- In this example, both the median class and modal class are the same (120-140). However, this is not always the case. Depending on the distribution of the data, the median class and modal class can be different. For instance, if the frequencies were altered such that the highest frequency was in a different class, the two classes could differ.
Thus, we conclude that the median class and modal class of grouped data are not always different; they can be the same depending on the distribution of the data.
Topper's Solved these Questions
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - SHORT ANSWERS QUESTIONS
42 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- REAL NUMBERSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - REAL NUMBERSEXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS
20 Videos
Similar Questions
It is true to say that the mean, mode and median of grouped data always be different? Justify your answer.
View SolutionState the modal class.

View SolutionCan Q and S, both be metals ? Justify your answer.
View SolutionDo different species of plant a growing in the same area show the same rate of tranpiration at a particular time ? Justify your answer.
View SolutionCan a stable molecule have zero bond order ? Justify your answer.
View SolutionWould you expect the first ionisation enthalpies for two isotopes of the same element to be the same or different? Justify your answer.
View SolutionIs the position of a star as seen by us its true position? Justify your answer.
View SolutionGive an example of an ecological pyramid which is always upright. Justify your answer.
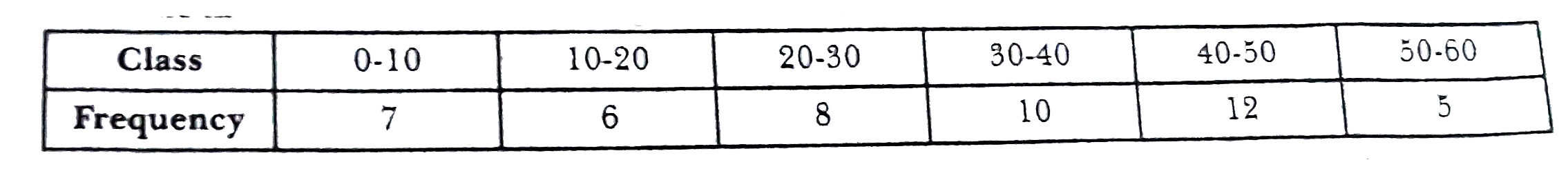
View SolutionWrite the median class of the following distribution:
View SolutionWill 35Cl and 37Cl have different valencies? Justify your answer.
View Solution
