Write an equation of a line passing through the point representing solution of the pair of linear equations x+y=2 and 2x-y=1, How many such lines can we find?
Given pair of linear equations is x+y−2=0 ...(i)
and 2x−y−1=0 ...(ii)
On comparing with ax+by+c=0, we get
a1=1,b1=1 and c1=−2 [from Eq. (i) ]
a−(2)=2,b2=−1 and c2=−1 [from Eq. (ii)]
Here, a1a2=12,b1b2=1−1
and c1c2=−2−1=21⇒a1a2≠b1b2
So, both lines intersect at a point. Therefore, the pair of equations has a unique solution.
Hence, these equations are consistent.
Now, x+y=2⇒y=2−x
If If x=0, then y=2 and if x=2, then y=0
x02y20PointsAB
and 2x−y−1=0⇒y=2x−1
If x=0, then y=-1, if x=12, then y=0 and if x=1, then y=1
x01/21y−101PointsCDE
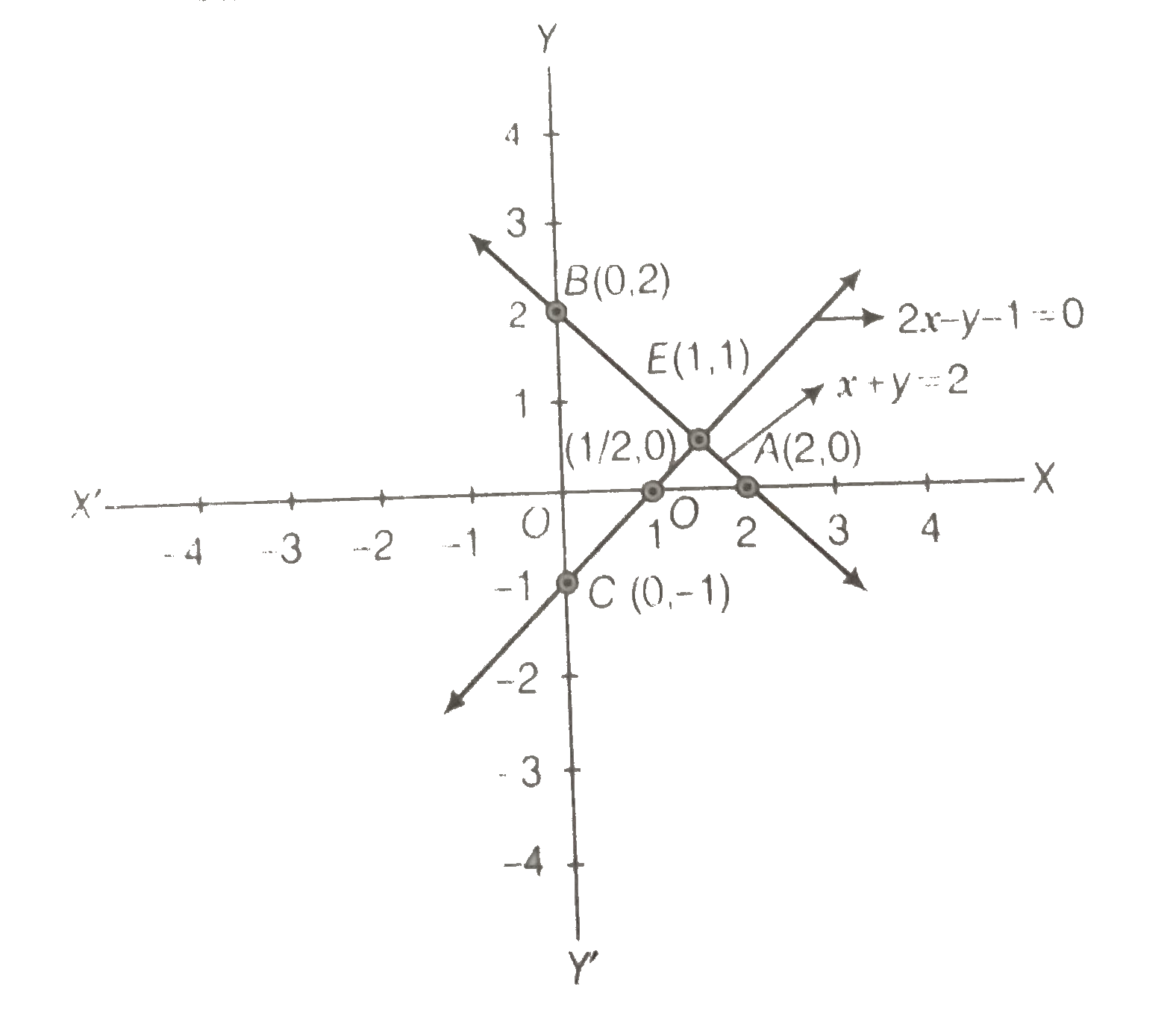
Plotting the points A (2,0) and B (0,2), we get the straight line AB. Plotting the points C (0, -1) and D(1/2, 0), we get the straight line CD. The lines AB and CD intersect at E (1,1).
Hence, infinite lines can pass through the intersection point of linear equations x+y=2 and 2x-y=1 i.e., E (1,1)like as y=x, 2x+y=3, x+2y=3. So on.
Topper's Solved these Questions
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 3.4 Long Answer Type Questions
13 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 3.2 Very Short Answer Type Questions
6 Videos
- INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - LONG ANSWER TYPES QUESTIONS
18 Videos
- POLYNOMIALS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - POLYNOMIALS EXERCISE - Long Answer Type Questions
6 Videos
Similar Questions
Find the equation of a line passing through the intersection of the lines x+3y=4 and 2x−y=1 and (0,0) lies on this line.
View SolutionFind the equation of a line passing through the point (−1,0) and perpendicular to the line x+5y=4.
View SolutionFind the equation of a line passing through the point (2,3) and parallel to the line 3x−4y+5=0 .
View SolutionEquation of a line passing through the point (2,3) and perpendicular to the line x+y+1=0 is :
View SolutionFind the equation of a line passing through the point of intersection of the lines x+3y+1=0 and 2x−y+3=0 and parallel to the line 3x−2y+1=0.
View SolutionFind the equation of a line passing through the point of intersection of the lines 3x+5y=−2 and 5x−2y=7 and perpendicular to the line 4x−5y+1=0.
View SolutionFind the equation of line joining origin to the point of intersection of the pair of lines 3x+y=10 and x−y=2.
View SolutionFind the equation of a line passing through the intersection of the lines 3x+2y=5 and 2x−y=1 and cuts equal intercepts on the axes.
View SolutionFind the equation of the straight line passing through origin and the point of intersection of the lines x+2y=7 and x−y=4 .
View SolutionThe equation of the line passing through the point (1,2) and perpendicular to the line x+y+1=0 is
View Solution
