Write class size and class limits of the following:
47,52,57,62,67,72,77
To solve the problem of finding the class size and class limits for the given data set (47, 52, 57, 62, 67, 72, 77), we will follow these steps:
Step 1: Identify the Class Size
The class size is the difference between consecutive data points. We can calculate it using the first two values in the data set.
- Calculation:
Class Size(h)=52−47=5
Step 2: Calculate Class Limits
Class limits can be calculated using the formula:
Lower Limit=xi−h2
Upper Limit=xi+h2
where xi is the midpoint of the class.
We will apply this formula for each value in the data set.
1. For x1=47:
- Lower Limit: 47−52=47−2.5=44.5
- Upper Limit: 47+52=47+2.5=49.5
- Class Interval: 44.5−49.5
2. For x2=52:
- Lower Limit: 52−2.5=49.5
- Upper Limit: 52+2.5=54.5
- Class Interval: 49.5−54.5
3. For x3=57:
- Lower Limit: 57−2.5=54.5
- Upper Limit: 57+2.5=59.5
- Class Interval: 54.5−59.5
4. For x4=62:
- Lower Limit: 62−2.5=59.5
- Upper Limit: 62+2.5=64.5
- Class Interval: 59.5−64.5
5. For x5=67:
- Lower Limit: 67−2.5=64.5
- Upper Limit: 67+2.5=69.5
- Class Interval: 64.5−69.5
6. For x6=72:
- Lower Limit: 72−2.5=69.5
- Upper Limit: 72+2.5=74.5
- Class Interval: 69.5−74.5
7. For x7=77:
- Lower Limit: 77−2.5=74.5
- Upper Limit: 77+2.5=79.5
- Class Interval: 74.5−79.5
Final Result:
- Class Size: 5
- Class Limits:
- 44.5 - 49.5
- 49.5 - 54.5
- 54.5 - 59.5
- 59.5 - 64.5
- 64.5 - 69.5
- 69.5 - 74.5
- 74.5 - 79.5
The class size is the difference between consecutive data points. We can calculate it using the first two values in the data set.
Class Size(h)=52−47=5
Class limits can be calculated using the formula:
Lower Limit=xi−h2
Upper Limit=xi+h2
where xi is the midpoint of the class.
- Lower Limit: 47−52=47−2.5=44.5
- Upper Limit: 47+52=47+2.5=49.5
- Class Interval: 44.5−49.5
- Lower Limit: 52−2.5=49.5
- Upper Limit: 52+2.5=54.5
- Class Interval: 49.5−54.5
- Lower Limit: 57−2.5=54.5
- Upper Limit: 57+2.5=59.5
- Class Interval: 54.5−59.5
- Lower Limit: 62−2.5=59.5
- Upper Limit: 62+2.5=64.5
- Class Interval: 59.5−64.5
- Lower Limit: 67−2.5=64.5
- Upper Limit: 67+2.5=69.5
- Class Interval: 64.5−69.5
- Lower Limit: 72−2.5=69.5
- Upper Limit: 72+2.5=74.5
- Class Interval: 69.5−74.5
- Lower Limit: 77−2.5=74.5
- Upper Limit: 77+2.5=79.5
- Class Interval: 74.5−79.5
- Class Size: 5
- Class Limits:
- 44.5 - 49.5
- 49.5 - 54.5
- 54.5 - 59.5
- 59.5 - 64.5
- 64.5 - 69.5
- 69.5 - 74.5
- 74.5 - 79.5
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - PART D3videos
STATISTICS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - QUARILATERAL EXERCISE - Part - D5videos
QUARILATERAL
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Practice Test8videos
SURFACE AREAS AND VOLUMES
Similar Questions
Knowledge Check
- Question 1 - Select One
If a class mark of a class-interval 8.5. The class size is 5,then the class limits of the corresponding class-interval is
A6.5-11.5B6−11C5.5−10.5D7−12
Write the median class of the following distribution:
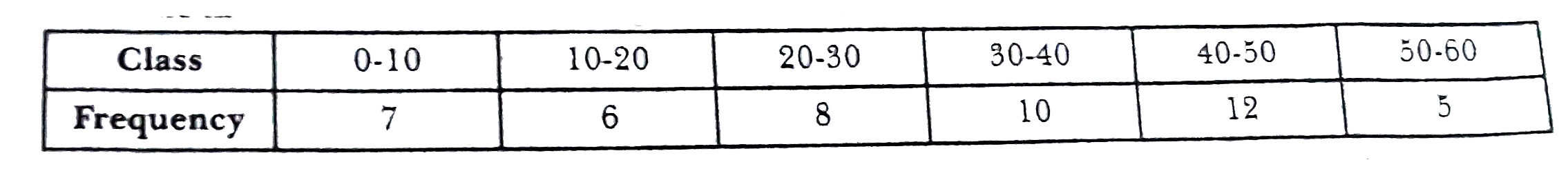 View Solution
View SolutionWrite the modal class of the following distribution :
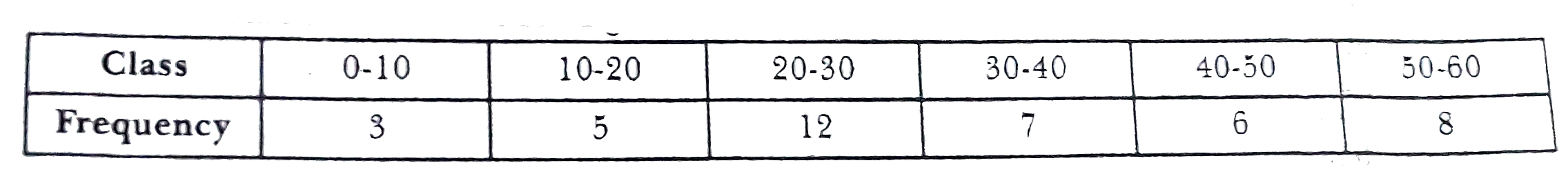 View Solution
View SolutionFind the class marks and class size of the following distribution.
 View Solution
View SolutionThe class marks of a distribution are : 47,52,57,62,67,72,77,82,87,92,97,102, determine the class size ,the class limits and the true class limits.
View SolutionThe class marks of a distribution are: 17,52,57,62,67,72,77,82,87,92,97,102 Determine the class size, the class limits and the true class limits
View SolutionWrite the upper classs limit and the lower class limit of the class 35-40.
View SolutionWrite upper and lower class limit of calss 27.5 to 30.5
View Solution

