|x|−1|x|−2≤0 then x lies in the interval
A
[−1,2]
B
(−2,2)
C
(−2,−1]∪[1,2)
D
[−1,1]
Video Solution
Text Solution
The correct Answer is:C
We have,
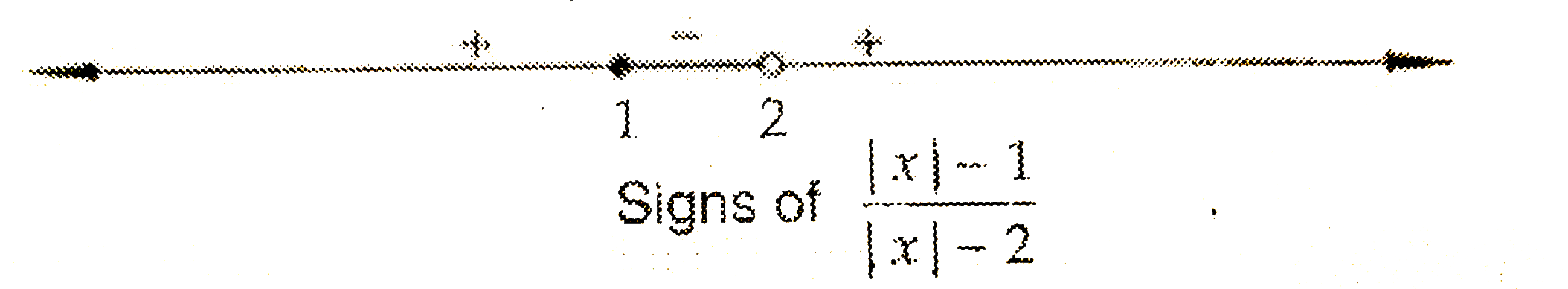
|x|−1|x|−2≤0
⇒1≤|x|<2
⇒x∈(−2,−1]∪[1,2)
|
Topper's Solved these Questions
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Section I - Solved Mcqs
20 Videos
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39 Videos
- ALGEBRA OF VECTORSBOOK - OBJECTIVE RD SHARMACHAPTER - ALGEBRA OF VECTORSEXERCISE - Chapter Test
30 Videos
- APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
Similar Questions
Knowledge Check
(x+1x)(x−1x)(x2+1x2−1)(x2+1x2+1) is equal to
Ax6+1x6Bx8+1x8Cx8−1x8Dx6−1x6If f(x)=∣∣ ∣ ∣∣1xx+12xx(x−1)(x+1)x3x(x−1)x(x−1)(x−2)(x+1)x(x−1)∣∣ ∣ ∣∣ then
Af(50)=50Bf(X)=xCf(100)=0Df(0)=0
If y=1+1x−1+2x(x−1)(x−2)+3x2(x−1)(x−2)(x−3), then the value of −9y'(4) is equal to
View Solution⎛⎝x+1x23−x13+1−x−1x−x12⎞⎠=
View SolutionIf D(x)=∣∣ ∣ ∣∣(x−1)(x−1)2x3(x−1)x2(x+1)3x(x+1)2(x+1)3∣∣ ∣ ∣∣ then the coefficient of x in D(x) is
View Solutionlimx→1[√x−1x−1+x2−1x2−x]
View SolutionSolve: (x−1)(x−2)(x−3)(x+1)(x+2)(x+3)>1
View SolutionSolve the following inequalities (x−1)(x−2)(x−3)(x+1)(x+2)(x+3)>1
View Solution
