X and Y are two correlated variables with the same standard deviation and the correlation coefficient r, the correlation coefficient between X and X+Y is
r2
√1−r2
√1+r2
0
The correct Answer is:C
To find the correlation coefficient between X and X+Y, we can follow these steps:
Step 1: Understand the variables
We are given two correlated variables X and Y with the same standard deviation. Let's denote the standard deviation of both X and Y as σ. The correlation coefficient between X and Y is given as r.
Step 2: Define the correlation coefficient
The correlation coefficient RX,X+Y can be defined as:
RX,X+Y=Cov(X,X+Y)σX⋅σX+Y
where Cov(X,X+Y) is the covariance between X and X+Y, and σX and σX+Y are the standard deviations of X and X+Y respectively.
Step 3: Calculate the covariance
To find Cov(X,X+Y):
Cov(X,X+Y)=Cov(X,X)+Cov(X,Y)=Var(X)+Cov(X,Y)
Since Var(X)=σ2 and Cov(X,Y)=r⋅σ2, we can substitute:
Cov(X,X+Y)=σ2+r⋅σ2=σ2(1+r)
Step 4: Calculate the variance of X+Y
Next, we need to find the variance of X+Y:
Var(X+Y)=Var(X)+Var(Y)+2Cov(X,Y)=σ2+σ2+2r⋅σ2=2σ2(1+r)
Thus, the standard deviation of X+Y is:
σX+Y=√Var(X+Y)=√2σ2(1+r)=σ√2(1+r)
Step 5: Substitute into the correlation coefficient formula
Now we can substitute back into the correlation coefficient formula:
RX,X+Y=Cov(X,X+Y)σX⋅σX+Y=σ2(1+r)σ⋅σ√2(1+r)
This simplifies to:
RX,X+Y=(1+r)√2(1+r)=√1+r√2=√1+r√2
Final Result
Thus, the correlation coefficient between X and X+Y is:
RX,X+Y=√1+r√2
We are given two correlated variables X and Y with the same standard deviation. Let's denote the standard deviation of both X and Y as σ. The correlation coefficient between X and Y is given as r.
The correlation coefficient RX,X+Y can be defined as:
RX,X+Y=Cov(X,X+Y)σX⋅σX+Y
where Cov(X,X+Y) is the covariance between X and X+Y, and σX and σX+Y are the standard deviations of X and X+Y respectively.
To find Cov(X,X+Y):
Cov(X,X+Y)=Cov(X,X)+Cov(X,Y)=Var(X)+Cov(X,Y)
Since Var(X)=σ2 and Cov(X,Y)=r⋅σ2, we can substitute:
Cov(X,X+Y)=σ2+r⋅σ2=σ2(1+r)
Next, we need to find the variance of X+Y:
Var(X+Y)=Var(X)+Var(Y)+2Cov(X,Y)=σ2+σ2+2r⋅σ2=2σ2(1+r)
Thus, the standard deviation of X+Y is:
σX+Y=√Var(X+Y)=√2σ2(1+r)=σ√2(1+r)
Now we can substitute back into the correlation coefficient formula:
RX,X+Y=Cov(X,X+Y)σX⋅σX+Y=σ2(1+r)σ⋅σ√2(1+r)
This simplifies to:
RX,X+Y=(1+r)√2(1+r)=√1+r√2=√1+r√2
Thus, the correlation coefficient between X and X+Y is:
RX,X+Y=√1+r√2
Topper's Solved these Questions
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - PROBLEM SET (1) TRUE/FALSE
6 Videos
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - PROBLEM SET (1) (FILL IN THE BLANKS)
10 Videos
- CORRELATION AND REGRESSION BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - QUESTION (STATE GIVING REASONS, WHETHER EACH OF THE FOLLOWING STATEMENTS IS TRUE OR FALSE)
10 Videos
- CONCEPTS OF SET THEORY BOOK - ML KHANNACHAPTER - CONCEPTS OF SET THEORY EXERCISE - Self Assessment Test
13 Videos
- DEFINITE INTEGRALBOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - Miscellaneous Questions (Assertion/Reason)
1 Video
Similar Questions
Knowledge Check
The two variates X and Y are uncorrelated and have standard deviations σXandσY respectively, the correlation coefficient between X+Y and X−Y is
AσXσYσ2X+σ2YB12(1σX+1σY)Cσ2X−σ2Yσ2X+σ2YDnone of theseThe coefficient of correlation is independent of
Achange of origin onlyBchange of scale onlyCboth changes of scale and originDneither change of scale nor change of originThe correlation between X and a−X is
A−1B−12C−14D0
There is no relationship between correlation coefficient and regression coefficient.
View SolutionFind out coefficient of rank correlation between X and Y
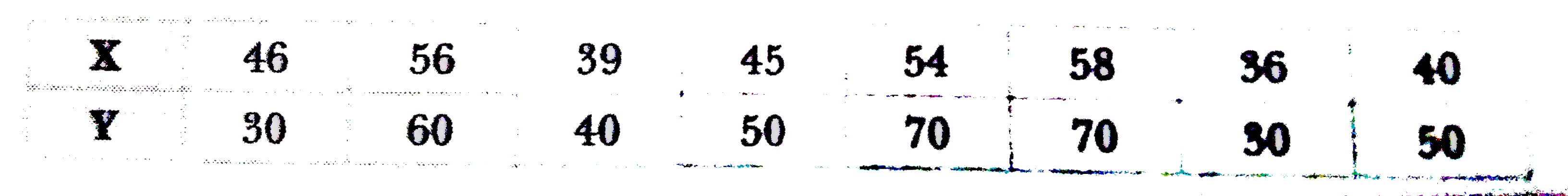
View SolutionThe value of product moment correlation coefficient between x and x is _____
View SolutionCalculate coefficient of correlation between series X and Y.

View Solutionif the regression coefficient of Y on X is4/3,then the regression coefficient of X and Y :
View Solution