Zoe won the raffle at a fair. She will receive the prize money in 5 monthly payments. If each payments is half as much as the previous month's payments and the total of the payments is $496, what is the amount of the first payment?
256
96
84
16
The correct Answer is:A
To solve the problem step by step, we can follow these instructions:
Step 1: Define the first payment
Let the amount of the first payment be X.
Step 2: Define the subsequent payments
The payments for the subsequent months will be:
- Second payment: X2
- Third payment: X4
- Fourth payment: X8
- Fifth payment: X16
Step 3: Write the equation for the total payments
The total of all the payments is given as $496. Therefore, we can write the equation:
X+X2+X4+X8+X16=496
Step 4: Find a common denominator
The common denominator for the fractions is 16. We can rewrite the equation as:
16X16+8X16+4X16+2X16+X16=496
Step 5: Combine the fractions
Now, combining the fractions gives:
16X+8X+4X+2X+X16=496
This simplifies to:
31X16=496
Step 6: Solve for X
To find X, multiply both sides by 16:
31X=496×16
Now, calculate 496×16:
496×16=7936
So, we have:
31X=7936
Now, divide both sides by 31:
X=793631
Step 7: Calculate X
Calculating 793631:
X=256
Conclusion
The amount of the first payment is 256.
Let the amount of the first payment be X.
The payments for the subsequent months will be:
- Second payment: X2
- Third payment: X4
- Fourth payment: X8
- Fifth payment: X16
The total of all the payments is given as $496. Therefore, we can write the equation:
X+X2+X4+X8+X16=496
The common denominator for the fractions is 16. We can rewrite the equation as:
16X16+8X16+4X16+2X16+X16=496
Now, combining the fractions gives:
16X+8X+4X+2X+X16=496
This simplifies to:
31X16=496
To find X, multiply both sides by 16:
31X=496×16
Now, calculate 496×16:
496×16=7936
So, we have:
31X=7936
Now, divide both sides by 31:
X=793631
Calculating 793631:
X=256
The amount of the first payment is 256.
Topper's Solved these Questions
- OTHER ALGEBRA STRATEGIESBOOK - PRINCETONCHAPTER - OTHER ALGEBRA STRATEGIESEXERCISE - Algebra Strategies Drill 1: No Calculator Section
4 Videos
- OTHER ALGEBRA STRATEGIESBOOK - PRINCETONCHAPTER - OTHER ALGEBRA STRATEGIESEXERCISE - Algebra Strategies Drill 2: Calculator-Permitted Section
6 Videos
- MATH TEST 01BOOK - PRINCETONCHAPTER - MATH TEST 01EXERCISE - MCQs
60 Videos
- PRACTICE SECTION 2 BOOK - PRINCETONCHAPTER - PRACTICE SECTION 2 EXERCISE - MCQs
60 Videos
Similar Questions
Question 37 and 38 refer to the following information
The U.S. federal Government tracks the Consumer Price Index (CPI)- a comprehensive standard used to estimate the average price change for the typical goods and services purchased by consumers. This measure gives economics a useful way to estimate the rates of the inflation or deflation, which reflects the respective general increase or decrease of prices of goods and services in the economy. The accompanying tables summarizes the changes in the CPI for the years 2005 through 2014, which can be assumed to be the corresponding percent rates of inflation.
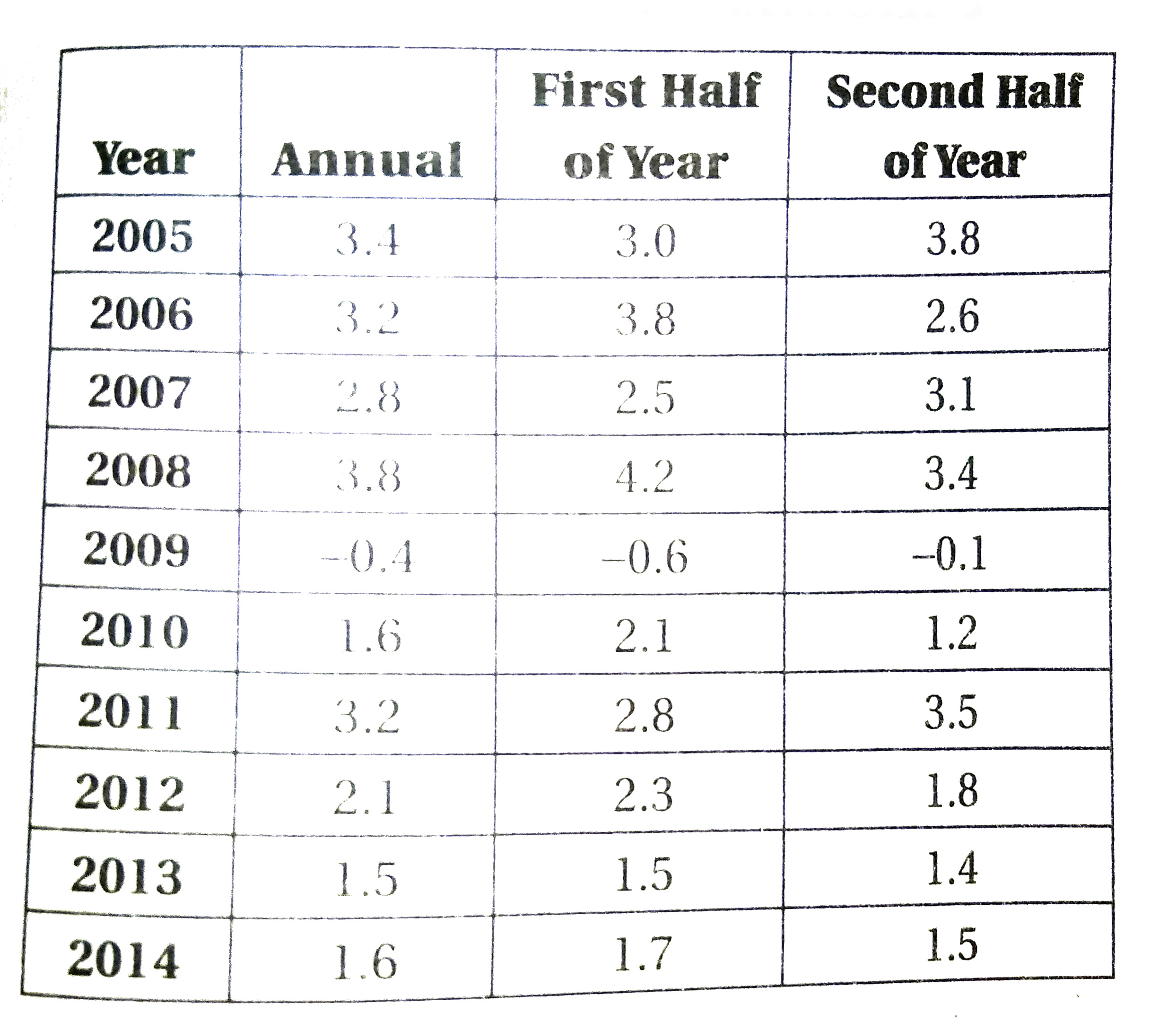
Q. At a beginning of 2015, a retired person is shopping for a retirement annunity, which is an investment policy that will give him fixed monthly payments for the rest of his life. He would like the amount of his annuity payments to more than keep up with the rate of inflation. He decides that he will choose a policy that issuse payments that increase annually at a rate of that is at least 1.5% greater than the average yearly compounded rate of inflation calculated from the period that extends from the second half of 2005 through the first half of 2008. What should be the minimum annual rate of increase in his monthly annuity payments, correct to the nearest tenth?
View SolutionA 7% car loan, which is compounded annually, has an interest payment of $210 after the first year. What is the principle on the loan?
View Solution
Knowledge Check
Ming purchased a car that had a purchase price of $5,400, which included all other costs and tax. She paid $1,000 as a down payment and got a loan for the rest of the purchase price. Ming paid off the loan by making 28 payments of $200 each. The total of all her payments, including the down payment, was how much more than car's purchase price?
A$ 200B$ 1,200C$4,400D$5,600Bob wishes to borrow some money. He needs to defer to the following formula, where M is the monthly payment, r is the monthly decimal interest rate, P is the amount borrowed, and t is the number of months it will take to repay the loan :
M=rP1−(11+r)t
If Bob secures a loan of $4,00 that he will pay back in 36 months with a monthly interest rate of 0.01, what is his monthly payment ?A$111.11B$119.32C$132.86D$147.16
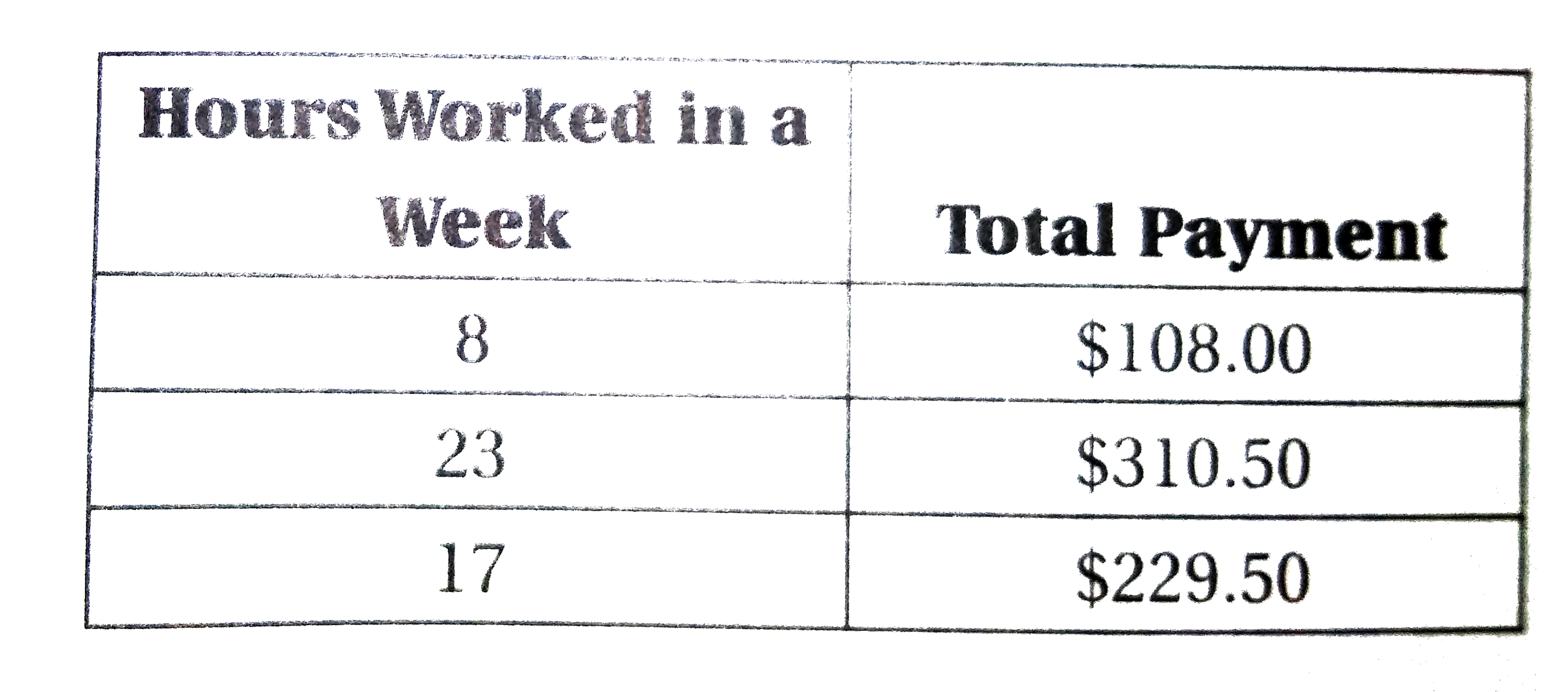
Andrew keeps track of his paychecks over the past several weeks, recording the number of hours he worked and his total payments as indicated in the table above. Hw wants to model the relationship between h hours worked and total payments, p , in dollars, using an equation of the form p=kh where k is a constant. Based on the data in the table, what value of k should be use?
View SolutionList price of a washing machine is Rs 9000. If the dealer allows a discount of 5% on the cash payment, how much money will a customer pay to the dealer in cash, if the rate of VAT is 10%?
View SolutionA man repays a loan of Rs.3250 by paying Rs.20 in the first month and then increases the payment by Rs.15 every month. How lon will it take him to clear the loan?
View SolutionAssume an auto loan in the amount of $12,000 is made. The loan carries and interest change of 14%. What is the amount of interest owed in the first three years of the loan, assuming there are no payments on the loan, and there is no––– compounding?
View SolutionA man repays a loan of Rs.3250 by paying Rs.20 in the first month and then increases the payment by Rs.15 every month. How long will it take him to clear the loan?
View SolutionA washing machine is available for Rs. 13,500 cash or Rs. 6,500 as cash down payment followed by three monthly instalments of Rs. 2,500 each. Find the rate of interest charged under instalment plan.
View Solution
