Complete Solutions to EXERCISE of chapter SAMPLE PAPER 7 of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SAMPLE PAPER 7 |
| AGRAWAL PUBLICATION | EXERCISE |
| Number Of Questions | 81 |
| Academic Year | 2023-24 |
Two positive integers p and q are expressible as `p=a^(3)b` and `q=ab^(2)`. Find the HCF (p,q) and LCM(p,q).
View SolutionCheck whether `-150` is a term of the A.P: 11,8,5,2`…..`
View SolutionThe roots of the equation `2x^(2)-6x+3=0` are
View SolutionFind the solution of the pair of equations :
`2x+3y=9`
`3x+4y=5`.
View SolutionTwo vertices of a triangle are (4,-5) and (-5,-2). If the centroid of the triangle of the origin determine the third vertex of the triangle.
View SolutionWhat is mid - point of line segment AB, where A (-5,0) and B(0,5) ?
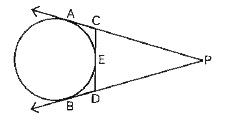
View SolutionIn the adjoining figure, if PA=10 cm, then find the perimeter of `Delta PCD`.
View SolutionIf `x sec 45^(@)=2`, then what is the value of x.
View SolutionIf `tan theta+cot theta=4`, then find the value of `tan^(4)theta+cot^(4)theta`.
View SolutionProve that : `(sin i)/(1+cos i)=(1-cos i)/(sin i)`
View SolutionIn an A.P., if a = 3.5, d = 0, n = 101, then find the value of `a_(n)`.
View SolutionIf A=900, `Sigmaf_(i)d_(l)=-400` and `Sigmaf_(i=100`, then what is the value of `bar(x)` ?
View SolutionA 6 faced cube has letters A,B,C,D,A and C on its six faces. This cube is rolled once. What is the probability of getting B or C ?
View SolutionA letter is chosen from letters of the word MAINTENANCE. What is the probability that it is N ?
View SolutionIf the equation `x^(2)+4x+k=0` has real and distinct roots, then find the value of 'k'.
View SolutionExamine if 1 and 2 are zeros of the polynomial `p(x)=x^(3)-3x^(2)-x+3`.
View SolutionWhich term of the A.P. -2,-7,-12, . . . will be -77 ?
View SolutionWhat type of lines are represented by the pair of equations :
`10x+6y=9` and `5x+3y+4=0` ?
View SolutionIf an event is sure to occur, then what is its probability of occurrence ?
View SolutionThe decimal expansion of the rational number `(14587)/(1250)` will terminate after:
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by `h=-16t^(2)+64t+80`
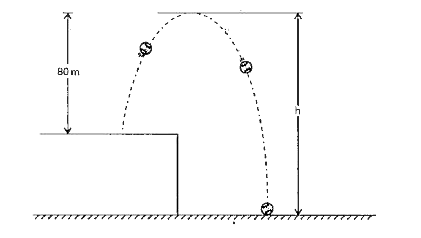
What is the height reached by the ball after 1 second ?
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by `h=-16t^(2)+64t+80`
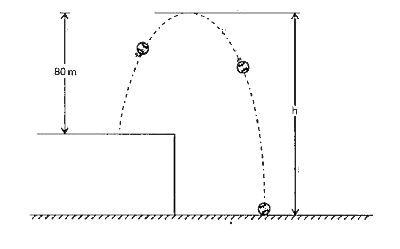
What is the maximum height reached by the ball ?
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by `h=-16t^(2)+64t+80`
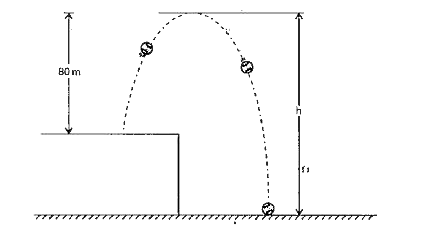
How long will the ball take to hit the ground ?
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by `h=-16t^(2)+64t+80`
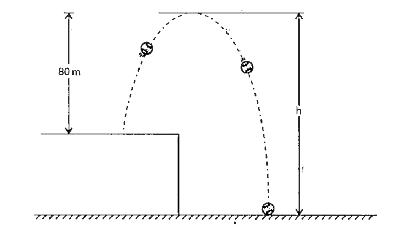
What are the two possible times to reach the ball at the same height of 128 m ?
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by `h=-16t^(2)+64t+80`
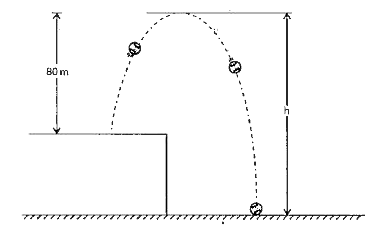
After 6 seconds, where is the ball ?
View SolutionAssuming that `sqrt(2)` is irrational, show that `5+sqrt(2)` is an irrational number.
View SolutionFind the greatest number that divides 45 and 240 completely.
View SolutionIf `x=acos^(3)theta and y = bsin^(3)theta`, prove that `((x)/(a))^(2//3)+((y)/(b))^(2//3)=1.`
View SolutionProve that `sqrt(sec^(2)theta + cosec^(2)theta) = tantheta + cottheta`.
View SolutionThe largest possible sphere is carved out of wooden solid cube of side 7 cm. What s the radius of this sphere ?
View SolutionA solid cuboid with dimensions 18 cm `xx` 12 cm `xx` 8 cm is melted and turned into a cube. What is the length of its edge ?
View SolutionA line intersects the y-axis and x-axis at the points P and Q respectively. If (2,-5) is the mid-point of PQ then find the coordinates of P and Q.
View SolutionA ladder 10m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.
View SolutionSolve for x and y :
`x+(y)/(4)=11," "(5x)/(6)-(y)/(3)=7`
View SolutionA 2-digit number is such that the product of the digit is 20. If 9 is subtracted from the number, the digits interchange their places. Find the number.
View Solution`triangle` ABC with vertices A(0-2,0),B(2,0) and C(0,2) is similar to `triangle` DEF with vertices D(-4,0) ,E(4,0) and F(0,4).
View SolutionProve that the length of the tangents drawn from an external point to a circle are equal.
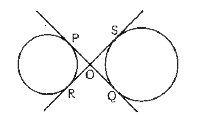
View SolutionIn the figure, PQ and RS are the common tangents to two circles intersecting at O.
Prove that PQ=RS
View SolutionA number x is selected from the numbers 1,2,3 and then a second number y is randomly selected from the numbers 1,4,9. What is the probability that the product xy of the two numbers will be less than 9?
View SolutionFind the value of :
`(5sin^(3)30^(@)+cos^(2)45^(@)-4tan^(2)30^(@))/(2sin 30^(@).cos30^(@)+tan45^(@))+cos 0^(@)`
View SolutionThe first and the last terms of an A.P. are 17 and 350 respectively. If the common difference is 9, then how terms are there in the A.P. ?
View SolutionTheorem 6.1 : If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
View SolutionBL and CM are medians of a triangle ABC right angled at A. Prove that `4(B L^2+C M^2)=5B C^2`
View SolutionWrite a prime number greater than 91 but less than 100.
View SolutionFind a zero of the polynomial
`x^3 - 8`
View SolutionWrite a quadratic polynomial whose sum of zeros is `( -1/4 )` and product of zeros is `( 1/4 ) `
View SolutionDetermine the roots of the equation `sqrt3x^2-2x-sqrt3=0`
View SolutionFind the `15^(th)` term of the AP, `x-7,x-2,x+3….`
View SolutionFind The discriminant of the equation `(x+1)^3=4-x+x^3`
View SolutionWrite the next term of the A.P :
`3,3+sqrt2,3+2sqrt2,3+3sqrt2` …
View SolutionSolve for x and y:
x + 2y = 9
2x - y = 8
View SolutionObtain the condition for the points (a,0), (0, b) and (1, 1) to be collinear.
View SolutionFind the coordinates of a point on y-axis which is equidistant from the points (6, 5) and (-4, 3)
View SolutionState the ASA criterion of similarity of triangles.
View SolutionDetermine the length of the altitude of an isosceles triangle of sides 6 cm, 6 cm and 4 cm.
View SolutionDraw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.
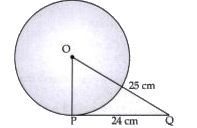
View SolutionFrom a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is
View SolutionIf 3cos A = 1, then find the value of cosec A.
View SolutionShow that, `(1+tan^2theta)/(1+cot^2 theta)=tan^2theta`
View SolutionFind the perimeter of a quadrant of a circle of radius 'r'.
View SolutionFind the total surface area of a quadrant of a sphere of radius 'r'
View SolutionFind the probability of drawing a green coloured ball from a bag containing 6 red and 5 black balls.
View SolutionFind the median of the first 50 even natural numbers.
View SolutionSatellite TV manufacturing businesses tend to have what economists call "economies of scale." When economies of scale exist, bigness can be its own reward. The more TV's you manufacture in a single run, lower the costs per unit, which in turn increases your bottom-line margins.
Keeping that in mind, a T.V. manufacturing company increases its production uniformly by fixed number every year. The company produces 8000, sets in the `6^(th)` year and 11,300 sets in the `9^(th)` year.
The company's production of the first year is:
View SolutionSatellite TV manufacturing businesses tend to have what economists call "economies of scale." When economies of scale exist, bigness can be its own reward. The more TV's you manufacture in a single run, lower the costs per unit, which in turn increases your bottom-line margins.

Keeping that in mind, a T.V. manufacturing company increases its production uniformly by fixed number every year. The company produces 8000, sets in the `6^(th)` year and 11,300 sets in the `9^(th)` year.
The company's production of the 8th year is:
View SolutionSatellite TV manufacturing businesses tend to have what economists call "economies of scale." When economies of scale exist, bigness can be its own reward. The more TV's you manufacture in a single run, lower the costs per unit, which in turn increases your bottom-line margins.

Keeping that in mind, a T.V. manufacturing company increases its production uniformly by fixed number every year. The company produces 8000, sets in the `6^(th)` year and 11,300 sets in the `9^(th)` year.
The company's total production of the first 6 years is:
View SolutionSatellite TV manufacturing businesses tend to have what economists call "economies of scale." When economies of scale exist, bigness can be its own reward. The more TV's you manufacture in a single run, lower the costs per unit, which in turn increases your bottom-line margins.

Keeping that in mind, a T.V. manufacturing company increases its production uniformly by fixed number every year. The company produces 8000, sets in the `6^(th)` year and 11,300 sets in the `9^(th)` year.
The company's production increases every year by:
View SolutionSatellite TV manufacturing businesses tend to have what economists call "economies of scale." When economies of scale exist, bigness can be its own reward.
The more TV's you manufacture in a single run, lower the costs per unit, which in turn increases your bottom-line margins.

Keeping that in mind, a T.V. manufacturing company increases its production uniformly by fixed number every year. The company produces 8000 sets in the 6th year and 11,300 sets in the 9th year.
In which year the company's production is 9100 sets ?
View SolutionEshan purchased a new building for her business. Being in the prime location, she decided to make some more money by putting up an advertisement sign for a rental ad income on the roof of the building.
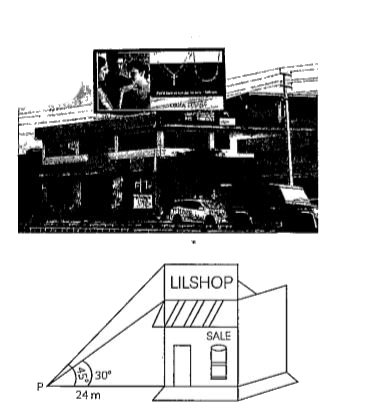
From a point P on the ground level, the angle of elevation of the roof of the building is `30^@` and the angle of elevation of the top of the sign board is `45^@`. The point P is at a distance of 24 m from the base of the building.
The height of the building (without the sign board) is
View SolutionEshan purchased a new building for her business. Being in the prime location, she decided to make some more money by putting up an advertisement sign for a rental ad income on the roof of the building.
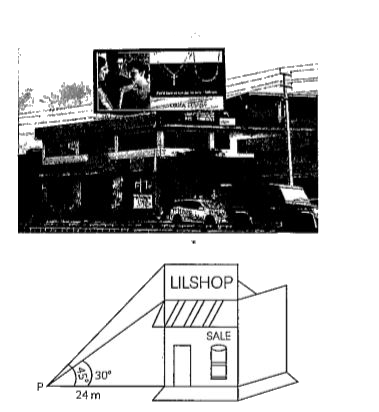
From a point P on the ground level, the angle of elevation of the roof of the building is `30^@` and the angle of elevation of the top of the sign board is `45^@`. The point P is at a distance of 24 m from the base of the building.
The height of the building (with the sign board) is
View SolutionEshan purchased a new building for her business. Being in the prime location, she decided to make some more money by putting up an advertisement sign for a rental ad income on the roof of the building.
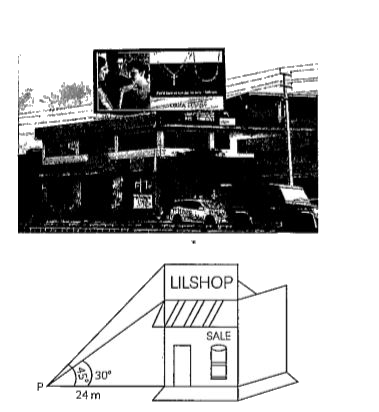
From a point P on the ground level, the angle of elevation of the roof of the building is `30^@` and the angle of elevation of the top of the sign board is `45^@`. The point P is at a distance of 24 m from the base of the building.
The height of the sign board is
View SolutionEshan purchased a new building for her business. Being in the prime location, she decided to make some more money by putting up an advertisement sign for a rental ad income on the roof of the building.
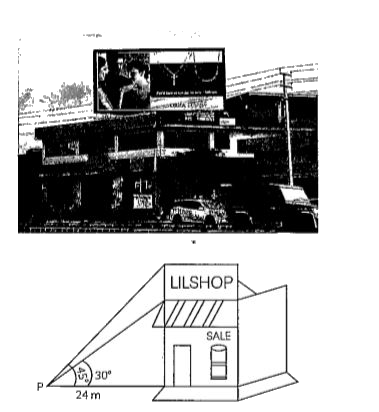
From a point P on the ground level, the angle of elevation of the roof of the building is `30^@` and the angle of elevation of the top of the sign board is `45^@`. The point P is at a distance of 24 m from the base of the building.
On the basis of the above information, answer any four of the following questions:
The distance of the point P from the top of the sign board, is
View SolutionEshan purchased a new building for her business. Being in the prime location, she decided to make some more money by putting up an advertisement sign for a rental ad income on the roof of the building.
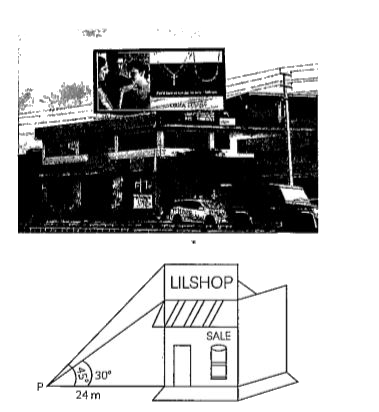
From a point P on the ground level, the angle of elevation of the roof of the building is `30^@` and the angle of elevation of the top of the sign board is `45^@`. The point P is at a distance of 24 m from the base of the building.
If the point of observation P is moved 10 m towards the base of the building, then the angle of elevation `theta` of the roof of the building is given by
View SolutionIn a toys manufacturing company, wooden parts are assembled and painted to prepare a toy. One specific toy is in the shape of a cone mounted on a cylinder.
For the wood processing activity center, the wood is taken out of storage to be sawed, after which it undergoes rough polishing, then is cut, drilled and has holes punched in it. It is then fine polished using sandpaper.
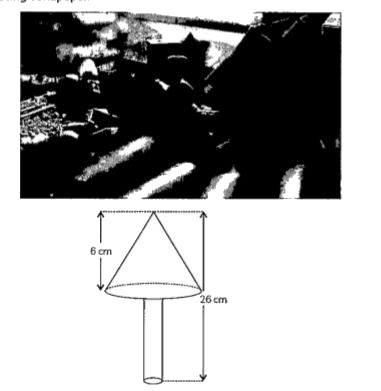
For the retail packaging and delivery activity center, the polished wood sub-parts are assembled together, then decorated using paint.
The total height of the toy is 26 cm and the height of its conical part is 6 cm. The diameters of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm.
If its cylindrical part is to be painted yellow, the surface area need to be painted is
View SolutionIn a toys manufacturing company, wooden parts are assembled and painted to prepare a toy. One specific toy is in the shape of a cone mounted on a cylinder.
For the wood processing activity center, the wood is taken out of storage to be sawed, after which it undergoes rough polishing, then is cut, drilled and has holes punched in it. It is then fine polished using sandpaper.
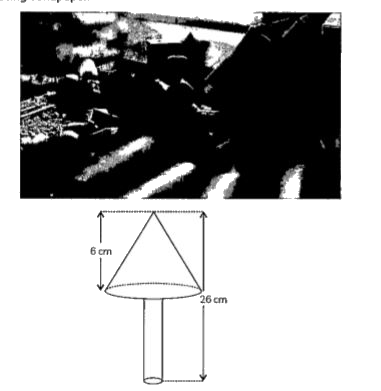
For the retail packaging and delivery activity center, the polished wood sub-parts are assembled together, then decorated using paint.
The total height of the toy is 26 cm and the height of its conical part is 6 cm. The diameters of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm.
If its conical part is to be painted green, the surface area need to be painted is
View SolutionIn a toys manufacturing company, wooden parts are assembled and painted to prepare a toy. One specific toy is in the shape of a cone mounted on a cylinder.
For the wood processing activity center, the wood is taken out of storage to be sawed, after which it undergoes rough polishing, then is cut, drilled and has holes punched in it. It is then fine polished using sandpaper.
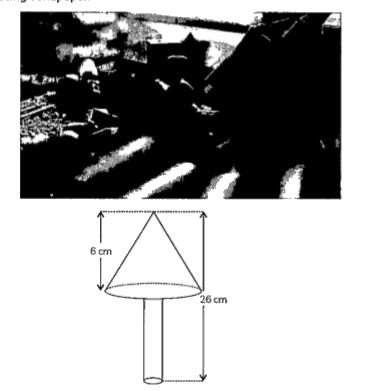
For the retail packaging and delivery activity center, the polished wood sub-parts are assembled together, then decorated using paint.
The total height of the toy is 26 cm and the height of its conical part is 6 cm. The diameters of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm.
The volume of the wood used in making this toy, is
View SolutionAs part of the 'Swachh Bharat Abhyan', some houses of a locality in Agra decided to clean up and beautify a Primary School of their locality by planting a number of plants. They involved the school kids and the local community in doing so.

Here is the data indicating the number of plants contributed by different houses:

If the mean number of plants contributed be 8.9, then how many houses contributed 7 to 9 plants?
View SolutionAs part of the 'Swachh Bharat Abhyan', some houses of a locality in Agra decided to clean up and beautify a Primary School of their locality by planting a number of plants. They involved the school kids and the local community in doing so.

Here is the data indicating the number of plants contributed by different houses:

On the basis of the above information, answer any four of the following questions:
The mode of the frequency distribution is
View SolutionAs part of the 'Swachh Bharat Abhyan', some houses of a locality in Agra decided to clean up and beautify a Primary School of their locality by planting a number of plants. They involved the school kids and the local community in doing so.

Here is the data indicating the number of plants contributed by different houses:

The median class of the frequency distribution is
View SolutionWrite the prime factorisation of 8190.
View SolutionFind the HCF of 2205, 5145 and 4410.
View Solution
EXERCISE questions and complete solutions for chapter SAMPLE PAPER 7 of AGRAWAL PUBLICATION of Class 10