Complete Solutions to EXERCISE of chapter MODEL PAPER of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | MODEL PAPER |
| BEYOND PUBLICATION | EXERCISE |
| Number Of Questions | 71 |
| Academic Year | 2023-24 |
If `alpha`, `beta` are zeroes of the polynomials `2x^2+7x+5`, find the value of `alpha+beta+alpha beta`?
View SolutionWrite the set A = {1, 4, 9, 16, 25, . . . }in set-builder form.
View SolutionThe larger of two complimentary angles is double the smaller. Find the angles.
View SolutionThe height of a rectangular stockroom is 5m and perimeter of its floor is 50m. Find the outer area of the four walls to be painted.
View SolutionSolve the equation `3^x = 5^(x+2)`
View SolutionFind the roots of the equation `5x^(2) - 6x - 2 = 0` by the method of completing the square.
View SolutionA cone of height 24 cm and radius of base 6 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the radius of the sphere.
View SolutionIf a,b and c are the zeroes of a polynomial of degree 3, then give the relations between the zeroes and the coefficients of the polynomial.
View SolutionCheck whether the equations 2x-3y=5 and 4x-6y=15 are consistent. Also verify by graphical representation.
View SolutionSolve each of the following pairs of equations by reducing them to a pair of linear equations. `(10)/(x+y)+(2)/(x-y)=4` and `(15)/(x+y)-(5)/(x-y)=-2`
View SolutionAn iron pillar consists of a cylindrical portion of 2.8 m. height and 20 cm. in diameter and a cone of 42 cm. height surmounting it. Find the weight of the pillar if 1 `cm^(3)` of iron weighs 7.5 g.
View SolutionA contractor construction job specifies a penalty for delay of completion beyond a certain date as follow. Rs. 200 for the first day. The penalty for each succeeding day being Rs. 50 more than the preceding day. How much money does the contractor pay as penalty if he has delayed the work by 30 days?
View SolutionA rectangular park is to be designed whose breadth is 3 m less than its length. Its area is to be 4 square metres more than area of a park that has already been made in the shape of an isosceles triangle with its base as the breadth of the reatangular park and of altitude 12m. Find its length and breadth.
View SolutionProve that `3 + 2 sqrt 5` is irrational.
View SolutionIf A - {x|x is a prime number and x < 20}
B = {x|2x + 1, `x in w` and x<9} then
Find `BnnnA`?
View SolutionIf A - {x|x is a prime number and x < 20}
B = {x|2x + 1, `x in w` and x<9} then
Find `BnnA`?
View SolutionIf A = {`x//x` is a prime number and x<20 } then
B = {`x//2x+1, x in w` and x<9} then Find
`A-B
View SolutionIf A = {`x//x` is a prime number and x<20 } then
B = {`x//2x+1, x in w` and x<9} then Find
B-A. What do you observe?
View SolutionThe coach of a cricket team of M.K.Nagar High School buys 3 bats and 6 balls for Rs. 3900. Later he buys one more bat and 2 balls for Rs. 1300. Identify the unknowns in each situation. We observe that there are two unknowns in each case.
View SolutionSolve the qudratic polynomial `x^(2) - 3x-4` by graphical method.
View SolutionThe H.C.F of 31, 43 and 47 is…………
View SolutionIf `x+y+z =xyz, ` then `sum(2x)/(1-x^(2))=`
View Solutionn(A) = 14, n(B)= 11, `n(AnnB)=19` then `n(AuuB)`=…..
View SolutionIf a fraction becomes 2 when 9 is added to its numerator and 1 when 2 is substracts from its denominator then the fraction is…..
View SolutionThe sum of squares of two consecutive positive even numbers is 340, then the numbers are…….
View SolutionWhich term of G.P. `3, 3sqrt(3), 9, …….` equals to 243?
View SolutionIf `sqrt a + sqrt b` is an irrational number, then which of the following is false?
View SolutionThe graph y=ax+b is a straight line which intersects X-axis at
View SolutionIf `x^2+ax+b=0,x^2+bx+a=0` have a common roots then
View SolutionCoefficient of x in a polynomial `ax^2 + bx + c` is '0'. Then its zeroes are
View SolutionThe series (n-1),(n-2),(n-3),…. Is a type of
View SolutionA metal cuboid of dimension `22 cm xx 15 cm xx 7.5` cm , was melted and cast into a cylinder of height 14 cm .What is its radius?
View SolutionIf log a, log b, log c are in A.P. then a,b,c are
View SolutionTo calculate the quantity of milk inside a bottle, we need to find out………….
View SolutionThe altitude of a right triangle is 7 cm less than its base. If the hypotenuse is 13 cm, find the other two sides.
View SolutionLength of the dark line given in the diagram
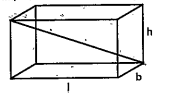
View Solution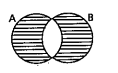
The shaded area in the figure shows
View SolutionSolution of x-y=2,x+y=0 lies in…..quadrant.
View SolutionInconsistent equations may represent.
View SolutionIf C (4, P) is a point on the line segment joining the points A(6,5) and B(2, 11)
Explain condition for the point C to become the mid point of AB.
View SolutionA boy observes that the length of his shadow is equal to his height. What is the angle of elevtation of the Sun rays?
View SolutionIn a class of 35, 28 students brought junk food for their lunch. What was the proabability that a student at random would have brought healthy food?
View SolutionThe circumference of a circle exceeds the diameter by 16.8 cm. Find the circumference of the circle.
View SolutionCompare the areas of two equilateral triangles which are constructed on side of a square and its diagonal.
View SolutionAn ant is at (4,5) on graph sheet mounted of a wall. If it moves to a point (5,2) and turns to reach another point (3,6). Find the distance travelled by the ant.
View SolutionShow that `(1 - sin theta)(1 + sin theta) (1 + tan^2 theta)`=1
View SolutionFind the median of the followiing distribution.

View SolutionA box contains 25 balls numbered as 1,2,3……….25. A ball is drawn from the box at random. What is the probability for getting the ball bearing the number, that
is divisible by 6
View SolutionA box contains 25 balls numbered as 1,2,3……….25. A ball is drawn from the box at random. What is the probability for getting the ball bearing the number, that
is a prime number.
View SolutionProve that a line drawn through the mid-point of one side of a Triangle parallel to another side bisects the third side (Using Basic proportionality theorem).
View SolutionVertices of a triangle ABC are A (3,5) B(7,4) and C (10,8). The mid point of the side BC, CA and AB are D,E and F respectively. Are the centroids of `triangle ABC` and d`triangle DEF` are same or not?
View SolutionIf ` tan x = ( 5)/( 12), ` then find sec x.
View SolutionThere is a tower beside the road, Rahim standing at the top of the tower observes two cars A and B on either side of the tower at an anglea of depression `30^@` and `60^@` are approaching the foot of the tower with a uniform speed of `10m/sec` and 5m/sec respe ctviely.If the height of the tower is `100sqrt 3`, then find the whihc car reaches the tower first and how many seconds the other car is late by the first one.
View SolutionA bag contains 6 yellow balls and some green balls. The probability of getting a green ball is triple that of a yellow ball. Determine number of Green balls in the bag and find the probability of each colour ball when a ball is drawn at time randomly.
View SolutionRamu has a triangular site. He observes the corners of the triangular site are (2,3),(4,1),(-2,5). Find the area of the swimming pool dug by joining of the mid points of the sides of the site.
View SolutionDraw a circle of diameter 6 cm from a point 5 cm away from its centre . Construct the pair of tangents to the circle and measure their length .
View SolutionIf slope of a line is “1” then the angle between the line and X -axis is
View Solution`(sec^4 - tan^4A)/(sec^2 A - tan^2 A)`=………
View SolutionThe angle of elevation of the sun when length of the shadow of a pole is ` sqrt3` times the height of the pole is
View SolutionThree coins are tossed simultaneously then probability of getting at lealst one tail is….
View SolutionThe mean of a data set consisting of 20 observation is 40. If one observation 53 was wrongly recorded as 33, then the correct mean will be
View SolutionFrom the figure if `angle APB` = `70^@` then `angle AOB`=…..
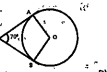
View SolutionThe following line has only one point in common to the circle
View SolutionWhich of the following is not possible?
View SolutionWhich of the following is correct?
View SolutionIn the figure `angle B` is an obtuse angle, then AC^2 =………..
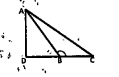
View SolutionModal class of the following distribution is

View SolutionFind the probability of existing 53 sundays in a common year.
View SolutionMatch the following.
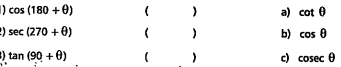
View Solution(0,0) (0,1) (1,0) are verticies of a …………..triangle.
View SolutionIf two towers of height X and Y subtend angles of `30^(@)" and "60^(@)` respectively at the centre of the line joining their feet, then X:Y is equal to
View Solution
EXERCISE questions and complete solutions for chapter MODEL PAPER of BEYOND PUBLICATION of Class 10