Complete Solutions to Practice Thoroughly of chapter CIRCLE of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | CIRCLE |
| KUMAR PRAKASHAN | Practice Thoroughly |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
A point P is 26cm away from the centre O of a circle and the length PT of the tangent drawn from P to the circle is 10cm. Find the radius of the circle.
View SolutionAB is a diameter and AC is a chord of a circle with centre O such that `angleBAC=30^@`. The tangent at C intersects AB at a point D. Prove that BC=BD.
View SolutionProve that the tangent drawn at the midpoint of an arc of a circle is parallel to the chord joining the end points of the arc.
View SolutionIf `d_(1), d_(2) (d_(2) gt d_(1))` be the diameter of the two cocentric circles and c be the length of a chord of a circle which is tangent to the other circle, prove that `d_(2)^(2)=c^(2)+d_(1)^(2)`.
View SolutionFrom an external point P, tangent PA=PB are drawn to a circle with centre O. If `anglePAB=50^@,` then find `angleAOB`.
View SolutionTwo cocentric circles are of diameters 30cm and 18cm. Find the length of the chord of the larger which touches the smaller circle.
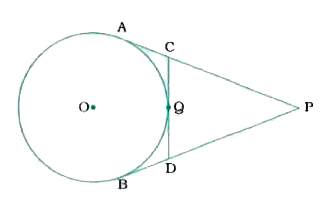
View SolutionIn the given figure, PA and PB are tangent to the circle from an external point P. CD is another tangent touching the circle at Q. If PA=12cm and QC=QD=3cm, then find PC+PD.
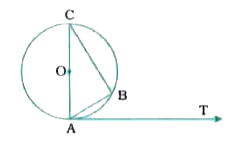
View SolutionAB is a chord of a circle with centre O, AC is a diameter and AT is the tangent at A as shown in the figure. Prove that `angleBAT=angleACB`.
View Solution
Practice Thoroughly questions and complete solutions for chapter CIRCLE of KUMAR PRAKASHAN of Class 10