Complete Solutions to Short Answer Type Questions of chapter ARITHMETIC PROGRESSIONS of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | ARITHMETIC PROGRESSIONS |
| NCERT EXEMPLAR | Short Answer Type Questions |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
Match the AP's given in column A with suitable common differences given in column B.
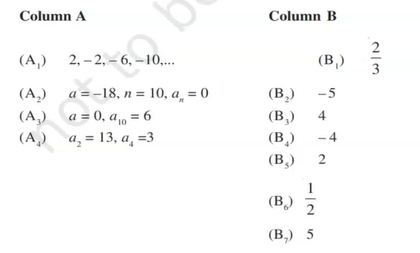
View SolutionVerify that each of the following is an AP and then write its next three terms.
(i) `0, (1)/(4),(1)/(2),(3)/(4), … " " ` (ii) `5,(14)/(3),(13)/(3),4, …`
(iii) `sqrt(3),2sqrt(3),3sqrt(3), … " " ` (iv) `a+b,(a+1)+b,(a+1)+(b+1), ...`
(v) `a,2a+1,3a+2,4a+3, …`
View SolutionWrite the first three terms of the AP's, when a and d are as given below
(i) `a=(1)/(2), d= -(1)/(6) " " `
(ii) `a= -5, d= -3`
View SolutionFind a, b and c such that the following numbers are in AP, a, 7, b, 23 and c.
View SolutionDetermine the AP whose fifth term is 19 and the difference of the eighth term from the thirteenth term is 20.
View SolutionThe 26th, 11th and the last terms of an AP are, 0, 3 and `-(1)/(5)`, respectively. Find the common difference and the number of terms.
View SolutionThe sum of the 5th and the 7th terms of an AP is 52 and the 10th term is 46. Find the AP.
View SolutionFind the 20th term of the AP whose 7th term is 24 less than the 11th term, first term being 12.
View SolutionIf the 9th term of an AP is zero, then prove that its 29th term is twice its 19th term.
View SolutionFind whether 55 is a term of the AP 7, 10, 13, … or not. If yes, find which term it is.
View SolutionDetermine `k`, so that `k^(2)+4k+8, 2k^(2)+3k+6` and `3k^(2)+4k+4` are three consecutive terms of an AP.
View SolutionSplit `207` into three parts such that these are in A.P. and the product of the two smaller parts is `4623`.
View SolutionThe angles of a triangle are in A.P. The greatest angle is twice the least. Find all the angles
View SolutionIf the `n`th terms of the two AP's 9, 7, 5, … and 24, 21, 18, … are the same, then find the value of `n`. Also, that term.
View SolutionIf sum of the 3rd and the 8th terms of an AP is `7` and the sum of the 7th and 14th terms is `-3`, then find the 10th term.
View SolutionFind the 12th term from the end of the AP
`-2, -4, -6, …, -100.`
View SolutionWhich term of the AP `53`, `48`, `43`, … is the first negative term ?
View SolutionHow many numbers lie between `10` and `300`, which divided by `4` leave a remainder `3``?`
View SolutionFind the sum of two middle terms of the AP `-4/3,-1,-2/3,-1/3,...,4(1/3)`
View SolutionThe first term of an AP is `-``5` and the last term is `45`. If the sum of the terms of the AP is `120`, then find the number of terms and the common difference.
View SolutionFind the sum
(i) `1+(-2)+(-5)+(-8)+ … +(-236)`
(ii) `(4-(1)/(n))+(4-(2)/(n))+(4-(3)/(n))+ …` upto `n` terms.
(iii) `(a-b)/(a+b)+(3a-2b)/(a+b)+(5a-3b)/(a+b)+ … ` to 11 terms.
View SolutionWhich term of the AP `-2,-7,-12, …` will be `-77` ? Find the sum of this AP upto the term `-77`.
View SolutionIf `a_(n)=3-4n`, then show that `a_(1),a_(2),a_(3), …` form an AP. Also, find `S_(20)`.
View SolutionIn an AP, If `S_(n)=n(4n+1),` then find the AP.
View SolutionIn an AP, If `S_(n)=3n^(2)+5n` and `a_(k)=164,` then find the value of k.
View SolutionIf `S_(n)` denotes the sum of first `n` terms of an AP, then prove that `S_(12)=3(S_(8)-S_(4)).`
View SolutionFind the sum of first `17` terms of an AP whose 4th and 9th terms are `-15` and `-30`, respectively.
View SolutionIf sum of first 6 terms of an AP is 36 and that of the first 16 terms is 256 , then find the sum of first 10 terms.
View SolutionFind the sum of all the `11` terms of an AP whose middle most term is `30`.
View SolutionFind the sum of last ten terms of the AP `8`, `10`, `12`, …, `126`.
View SolutionFind the sum of first seven numbers which are multiples of `2` as well as of `9`.
View SolutionHow many terms of the AP `-15, -13, -11, … ` are needed to make the sum `-55` ?
View SolutionThe sum of the first n terms of an AP whose first term is `8` and the common difference is `20` is equal to the sum of first `2n` terms of another AP whose first term is `-30` and the common difference is `8`. Find `n`.
View SolutionKanika was given her pocket money on Jan `1st` , `2008`. She puts `Rs.` `1` on day `1`, `Rs.` `2` on day `2`, `Rs.` `3` on day `3` and continued doing so till the end of the month, from this money into her piggy bank she also spent `Rs.` `204` of her pocket money, and found that at the end of the month she still had `Rs.` `100` with her. How much was her pocket money for the month ?
View SolutionYasmeen saves `Rs.` `32` during the first month, `Rs.` `36` in the second month and `Rs.` `40` in the third month. If she continues to save in this manner, in how many months will she save `Rs.` `2000` ?
View Solution
Short Answer Type Questions questions and complete solutions for chapter ARITHMETIC PROGRESSIONS of NCERT MATHS (ENGLISH) of Class 10