Complete Solutions to Unit Exercise-3 of chapter ALGEBRA of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | ALGEBRA |
| SURA PUBLICATION | Unit Exercise-3 |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
Solve `(1)/(3)(x+y-5)=y-z=2x-11=9-(x+2z)`.
View SolutionOne hundred and fifty students are admitted to a school. They are distributed over three sections A, B, C. If 6 students are shifted from section A to Sections C, the students will have equal number of students. If 4 times of students of section C exceeds the number of students of setion A by the number of students in sectionB, find the number of students in the sections.
View SolutionIn a three-digit number, when the tens and the hundreds digit are interchanged the new number is 54 more than three times the original number. If 198 is added three times the original number. If 198 is added to the number, the digits are reversed. The tests difit exceeds the hundreds digit by twice as that of the tens digit exceeds the unit digit. Find the originall number.
View SolutionFind the least number common multiple of `xy(k^(2)=1)+k(x^(2)+y^(2)) and xy(k^(2)-1)+k(x^(2)-y^(2))`.
View SolutionFind the GCD of following by division algorithm `2x^(4)+13x^(3)+27x^(2)+23x+7, x^(3)+3x^(2)+3x+1, x^(2)+2x+1`.
View SolutionReduce the given Rational expression to its lowest form
`(x^(3a)-8)/(x^(2a)+2x^(a)+4)`
View SolutionReduce the given Rational expression to its lowest form
`(10x^(3)-25x^(2)+4x-10)/(-4-10x^(2))`.
View SolutionSimplify `((1)/(p)+(1)/(q+r))/((1)/(p)-(1)/(q+r))times[1+(q^(2)+r^(2)-p^(2))/(2pr)]`
View SolutionArul, Ravi, and Ram working together can clean a store in 6 hours. Working alone, Ravi takes twice as long to clean the store as Arul does. Ram needs three times as long as Arul does. How long would it take each if they are working alone?
View SolutionFind the square root of `289x^(4)-612x^(3)+970x^(2)-684x+361`.
View SolutionSolve `sqrt(y+1)+sqrt(2y-5)=3`.
View SolutionA boat takes 1.6 hours longer to go 36kms up a river than down the river. If the speed of the water current is 4 km per hr, what is the speed of the boat in still water?
View SolutionIs it possible to design a rectangular park of perimeter 320m and area `4800m^(2)`? If so find its length and breadth.
View SolutionAt t minutes past 2pm, the time needed to 3 pm is 3minutes less than `(t^(2))/(4)` find t.
View SolutionThe number of seats in a row is equal to the total number of rows in a hall. The total number of seats in the hall will increase by 375 if the number of rows is doubled and the number of seats in each row is reduced by 5. Find the number of rows in the hall at the beginning.
View SolutionIf a and b are the roots of the polynomial `f(x)=x^(2)-2x+3`, find the polynomial whose roots are
`(alpha+2),(beta+2)'
View SolutionIf a and b are the roots of the polynomial `f(x)=x^(2)-2x+3`, find the polynomial whose roots are
`(alpha-1)/(alpha+1), (beta-1)/(beta+1)`
View SolutionIf -4 is a root of the equation `x^(2)+px-4=0` and if the equation `x^(2)+px+q=0` are equal roots, find the values of p and q.
View SolutionTwo farmers and Ravi cultivates three varieties of grains namely rice, wheat and ragi. If the sale (in ₹) of three varieties of grains by both the farmers in the month of April is given by the matrix.
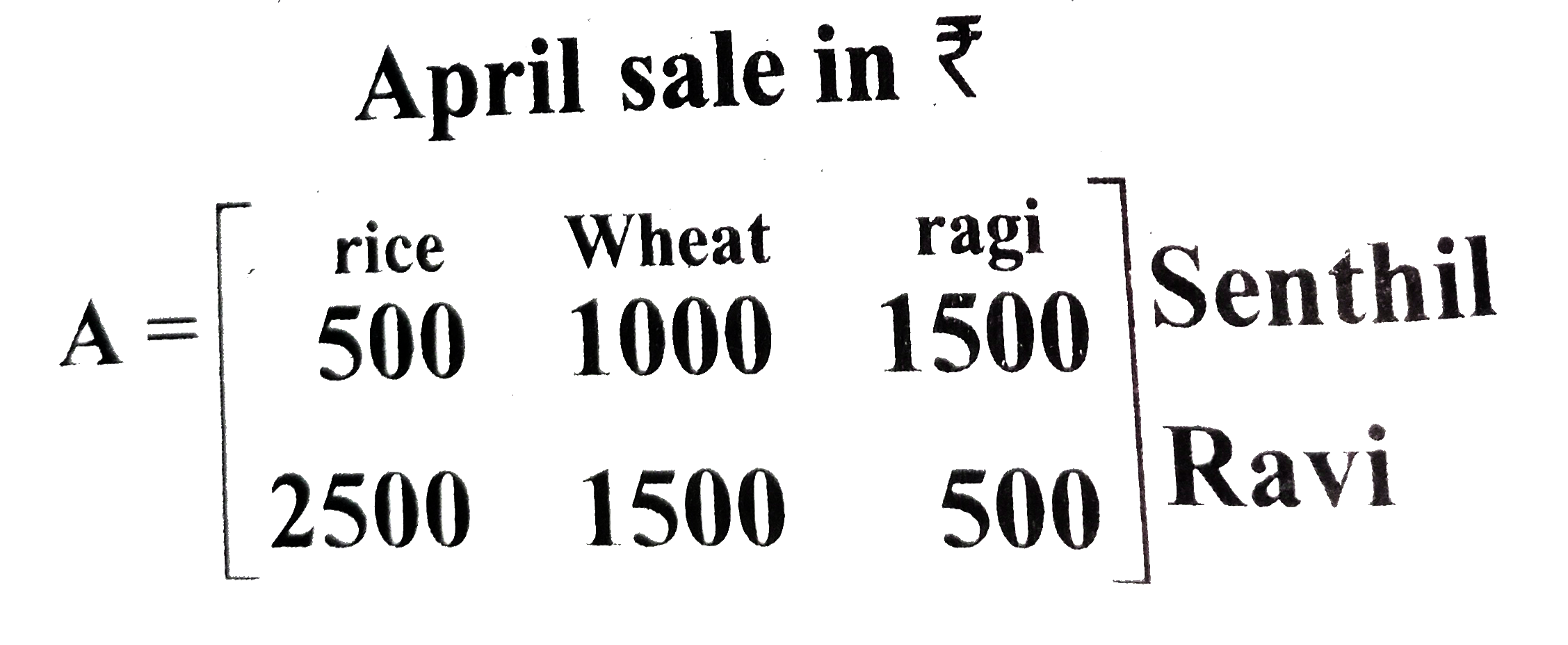 and the May month sale (in ₹) is exactly twice as that of the April month sale for each variety.
and the May month sale (in ₹) is exactly twice as that of the April month sale for each variety.
What is the average sales of the months April and May.
View SolutionTwo farmers and Ravi cultivates three varieties of grains namely rice, wheat and ragi. If the sale (in ₹) of three varieties of grains by both the farmers in the month of April is given by the matrix.
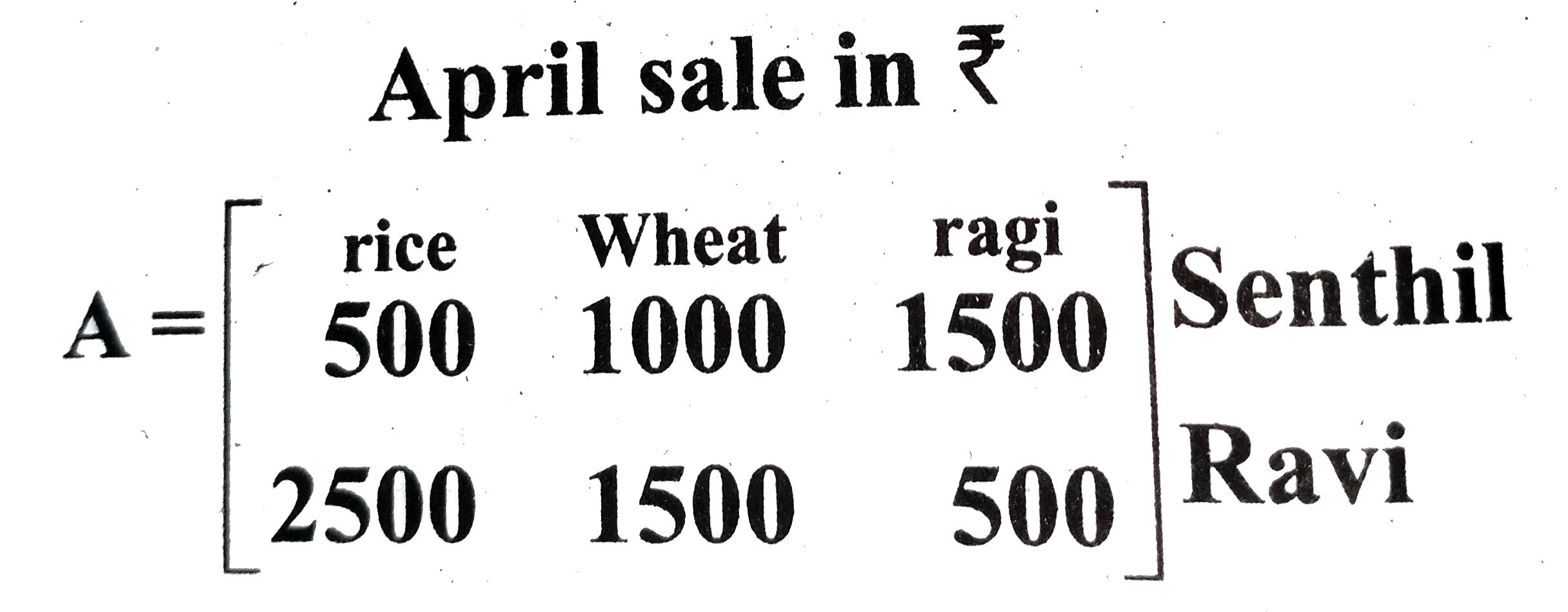 and the May month sale (in ₹) is exactly twice as that of the April month sale for each variety.
and the May month sale (in ₹) is exactly twice as that of the April month sale for each variety.
If the sales continue to increase in the same way in the successive months, what will be sales in the month of August?
View SolutionIf `costheta[(costheata, sintheta), (-sintheta, costheta)]+sintheta[(x, -costheta), (costheta, x)]=I_(2)`. Find x.
View SolutionGiven `A=[(p, 0), (0, 2)], B=[(0, q), (1, 0)], C=[(2, -2), (2, 2)]` and If `BA=C^(2)`, find p and q.
View Solution`A=[(3, 0), (4, 5)], B=[(6, 3), (8, 5)], C=[(3, 6), (1, 1)]` find the matrix D, such that `CD-AB=0`.
View Solution
Unit Exercise-3 questions and complete solutions for chapter ALGEBRA of SURA MATHS (TAMIL ENGLISH) of Class 10
All Exercises from SURA PUBLICATION Class 10 MATHS ALGEBRA
- ADDITIONAL QUESTIONS & ANSWERS
- EXECRISE 3.1
- EXECRISE 3.10
- EXECRISE 3.11
- EXECRISE 3.12
- EXECRISE 3.13
- EXECRISE 3.14
- EXECRISE 3.15
- EXECRISE 3.16
- EXECRISE 3.17
- EXECRISE 3.18
- EXECRISE 3.19
- EXECRISE 3.2
- EXECRISE 3.3
- EXECRISE 3.4
- EXECRISE 3.5
- EXECRISE 3.6
- EXECRISE 3.7
- EXECRISE 3.8
- EXECRISE 3.9
- GOVERNMENT EXAM QUESTIONS
- Unit Exercise-3
- Unit Test