Complete Solutions to ADDITIONAL PROBLEMS FOR PRACTICE of chapter CO-ORDINATE GEOMETRY of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | CO-ORDINATE GEOMETRY |
| TARGET PUBLICATION | ADDITIONAL PROBLEMS FOR PRACTICE |
| Number Of Questions | 33 |
| Academic Year | 2023-24 |
Using distance formula, show that the points `(1,5), (2,4)` and
`(3,3)` are collinear.
View SolutionShow that point `P(-3,2)` `Q(1, -2)` and `R(9, -10)`
are collinear
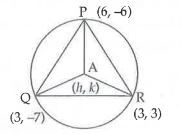
View SolutionFind the co-ordinates of the center of the circle passing through the point. P(6,-6),Q(3,-7) and R(3,3).
View SolutionFind the value of `y` if the distance between the points
`A (2,-2)` and `B(-1,y)` is `5`.
View SolutionShow that point (5,3) is equidistant from the points (1,1) and (3,-1)
View SolutionFind the co-ordinates of a point on Y-axis which is equidistant from M(-5,-2) and N (3,4)
View SolutionIf point `(x,y)` is equidistant from points `(7,1)` and `(3,5)` show that `y=x-2`
View SolutionFind the realation between x and y , such that the point `(x,y)` is equidistant from points (-1,8) and (3,4)
View SolutionShow that the points A(1,2),B(4,3),C(1,0) and D(-2,-1) are the vertices
of a parallelogram.
View SolutionIf `P(–2, 4), Q(4, 8), R(10, 5) and S(4, 1)` are the vertices of a quadrilateral, show that it is a parallelogram.
View SolutionShow that points (1,7),(4,2),(-1,-1) and (-4,4) are vertices of a suare.
View SolutionShow that A(4,-1), B(6,0), C(7,-2) and D(5,-3) are vertices of a square.
View SolutionShow that the points P(0,2) Q(3,-1) R(-2,-6) and S(-5,-3) are the
vertices of a rectangle.
View Solution`A(-3,-4), B(-5,0),C(3,0)` are the vertices of `Delta ABC` . Find the co-ordinates of the circumcenter of `Delta ABC.`
View SolutionIf `A (2,7) ,B (-6,1)` and `C (-5,8) `are the vertices of a triangle, then find the co-ordinates of circumcenter of that triangle .
View SolutionIn `Delta` PQR , if P (5,-1) ,Q(-3,3) ,R (-2,6) are the verties, then find the
co-ordinates of the circumcentre and the radius of the circumcircle.
View SolutionIf A (3,5) , B (7,9) and Q divides seg AB in the ratio `2:3`,
then find the co-ordinates of points Q .
View SolutionIf C(-2,-6), D (2,10) and Q divides seg CD in the ratio `4:3`.
Find the co-ordinates of points Q.
View SolutionIf point T divides the segment AB with A (-7,4) and B (-6,-5) in the
ratio `7:2`, find the co-ordinates of T
View SolutionIf point P(-4,6) divides the line segment AB with A(-6,10) in the
ratio ` 2 :1` , then coordinates of the point B are _ _ _ _ _ _ _ _
View SolutionThe line segment LM is divided by point `B (-7,2)` in the ratio 2:1
if `l (5,4)`, then find the co-ordinates of `M `
View SolutionA (12,5) ,B (4,-3) and A-P-B . Find the ratio in which point P (9,2)
divides segment AB.
View SolutionFind the coordinates of the points of trisection of the line segment joining the points (2,-2) and (-7,-4)
View SolutionIf P is the midpoint of line segment AB with`A(-4,2)` and `B(6,2)` then coordinates of point `P` are.
View SolutionIf A(-14,-10),B(6,-2) is given,find the co-ordinates of the points which divide segment AB into four equal parts.
View SolutionThe centroid of `Delta` PQR is G (2,-4), and P(3,-5) and Q(-1,-6) are its vertices. Then find the co-ordinates of R.
View SolutionIf slope of the line joining points P(k,0) and Q(-3,-2) is `2/7` , then find k.
View SolutionShow that points P (-2,3) ,Q(1,2) ,R(4,1) are collinear.
View SolutionShow that points P (3,1) ,Q(-1,9) ,and R(4,-1) are collinear.
View SolutionFind the value of k, if the points A(-1,1) B(5,7) and C(8,k) are collinear
View SolutionFind the value of k, so that line joining the points A (3,k) and B(2,7) is parallel to line joining the points C(-1,4) and D(0,6).
View SolutionShow that `square` ABCD is a parallelogram if A (4,8) ,B(5,5), C(2,4) ,D(1,7) .
View SolutionIf A(6,1) ,B(8,2) ,C(9,4) and D (7,3) are the vertices of `square` ABCD ,
show that `square` ABCD is a parallelogram.
View Solution
ADDITIONAL PROBLEMS FOR PRACTICE questions and complete solutions for chapter CO-ORDINATE GEOMETRY of TARGET PUBLICATION of Class 10