Complete Solutions to Exercise of chapter QUESTION PAPER 2018 of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | QUESTION PAPER 2018 |
| UNITED BOOK HOUSE | Exercise |
| Number Of Questions | 53 |
| Academic Year | 2023-24 |
Choose the correct option in each case from the following questions : Interest on ₨. A at the simple interest 10% per annum for b months is ____
01:45View SolutionIf x prop y, then ___
02:21View SolutionIf `angle A = 100^@` of a cyclic quadrilateral ABCD, then the value of `angle C` is _____
01:36View SolutionThe sexagesimal value of `(7Pi)/(12)` is ___
01:22View SolutionIf side of a cube is a unit and the diagonal of the cube is d unit then the relation between a and d will be ___
05:18View SolutionIf the mean of the numbers 6, 7, x, 8, y, 16 is 9 then ____
02:47View SolutionFill up the blanks (any Five) : If the simple interest of a principal for n years at r% p.a. be ₨.pnr/25, then the principal will be ₨. = _____________
02:13View SolutionThe equation `(a - 2) x^2 + 3x + 5 = 0` will not be a quadratic equation for a = ___________
02:24View SolutionIf ABCD is a cyclic parallelogram then `angle A` is _________
03:05View SolutionIf `tan 35^@ tan 55^@ = sin theta`, then the lowest positive value of `theta` will be _______
01:39View SolutionThe shape of a pencil with one end sharpened is the combination of a cylinder and a __________
01:29View SolutionThe measures of central tendency are Mean, Median and ___________________
01:30View SolutionWrite True or False:
At same rate of interest the simple interest for 2 years is more then the compound interest on the same principal.04:29View Solution` x^3y, x^2y^2 and xy^3` are in continued proportion or not.
01:58View SolutionThe angle in the segment of a circle which is less than a semi circle is an obtuse angle.
01:02View SolutionThe simplest value of `(cos 53^@)/(sin 37^@)` is _______
01:23View SolutionIf the radius of a sphere is twice that of 1 st sphere then the volume of the sphere will be ------that of 1 st sphere.
02:47View SolutionScore 1 2 3 4 5 No. of students 3 6 4 7 5 The mode of the distribution is 3.
01:05View SolutionThe rate of simple interest per annum reduces from 4% to 3 3/4% and for this, a person's annual income decreases by ₨. 60. Determine the principal of that person.
02:18View SolutionA and B start a business with ₨. 15,000 and ₨. 45,000 respectively. After 6 months B received ₨. 3,030 as profit. What is A's profit?
03:17View SolutionIf 2x + 1/x = 2, then find the value of `(x)/(2x^2+x+1)`.
01:20View SolutionIf the roots of a quadratic equation be 2 and -3, then write the equation.
01:32View SolutionThe line parallel to BC of `triangleABC` meets AB and AC at P and Q respectively. If AP = 4cm, QC = 9cm and PB = AQ, then find the length of PB.
02:49View SolutionThe radius of a circle with centre O is 5cm. P is a point at a distance 13cm from O. PQ and PR are two tangents to this circle. Find the area of the quadrilaterral PQOR.
02:12View SolutionThe two chords AB and CD of a circle are at equal distance from the centre O. If `angleAOB = 60^@` and CD = 6cm, then calculate the length of the raduis of the circle.
04:26View SolutionIf `tan theta + cot theta = 2`, then find the value of `tan^7theta + cot^7theta`.
01:35View SolutionIf the ratio of length of shadow of a tower and height of the tower is `sqrt3 : 1`, find the angle of elevation of the Sun.
01:02View SolutionThe volumes of two right circular cylinders are same. The ratio of their height is 1 : 2. Find the ratio of their radii.
01:41View SolutionThe volumes of a solid hemisphere is `144Pi` cu. Cm, then find the diameter of the sphere.
01:43View SolutionThe mean of a frequency distribution is 8.1, if fixi = 132 + 5K and fi = 20 then what is the value of K?
01:09View SolutionAminur has taken a loan of ₨. 64,000 from a bank. If the rate of interest be 2.5 paise per rupee per annum, calculate the compound interest payable after 2 years.
04:00View SolutionA, B and C start a business with the capical of ₨. 6,000, ₨. 8,000 and ₨. 9,000 respectively. After few months A invests ₨. 3,000 more in the business. At the end of the year they gained ₨. 30,000 and C got ₨. 10,800 as share of profit. When did A invest ₨. 3,000 more?
08:05View SolutionAnswer any One question : Solve : `((x+4)/(x-4))^2 - 5((x+4)/(x-4)) + 6 = 0`, `(x ne 4)`.
02:58View SolutionThe digit in the unit's place of a two figited number is 6 more than that at the ten's place. The product of the digits is 12 less than the number. Find the possible values of the digit in the unit place.
02:25View SolutionAnswer any One question : Find the simplest value of : `sqrt7(sqrt5 - sqrt2) - sqrt5(sqrt7 - sqrt2) + (2sqrt2)/(sqrt5+sqrt7)`
01:27View SolutionIf `x prop y and y prop z`, then prove that : `(x^2+y^2+z^2) prop (xy+yz+zx)`.
02:36View SolutionAnswer any One question : If a+b-c/a+b = b+c-a/b+c = c+a-b/c+a and a+b+c ne 0, then prove that a = b = c.
00:56View SolutionIf x : a = y : b = z : c,then show that : `(a^2+b^2+c^2)(x^2+y^2+z^2) =(ax+by+cz)^2`.
02:30View SolutionAnswer any One queation : Prove that, if a perpendicular is draw on the hypotenuse from the right angled triangle, two triangles so formed on the two sides of the perpendicular are each similar to the original triangle and also similar to each other.
03:09View SolutionProve that the tangent and the radius through the point of contact of a circle are perpendicular to each other.
03:34View SolutionAnswer any One question : In `triangleABC`, AD is perpendicular on BC and `AD^2 = BD`. DC, prove that `angleBAC` is a right angle.
03:40View SolutionA straight line intersects one of the two concentric circles at the points A and B and other at the points C and D. Prove that AC = BD.
06:52View SolutionAnswer any One queation : Construct two circles of radii 4 cm and 2 cm and the distance between their centres is 7 am. Construct a direst common tangent of the circles. (Only traces of contruction are required).
02:33View SolutionConstruct a triangle whose two sides are 9 cm and 7 cm and the angle between them is `60^@` Construct the incircle of the triangle. (Only traces of construction are required).
02:25View SolutionAnswer any Two questions : An are of length 220 cm of a circle makes an angle `60^@ at the centre. Find the radius of the circle.
00:55View SolutionIf `cos^2 theta - sin^2 theta =1/2`, then find value of `tan^2 theta`.
01:34View SolutionFind the value of : `(sec17^@)/(cosec73^@)+(tan68^@)/(cot22^@)+cos^244^@+cos^246^@`.
01:13View SolutionAnswer any One question : The length of the shodow of a post becomes 3 meters smaller when the angle of elevation of the Sun increases from `45^@` to `60^@`. Find the height of the post.
02:27View SolutionA man standing on a railway bridge `5sqrt3` meters high, observes the engine of a train at an angle of depression `30^@`. But after 2 seconds, he observes the engine at an angle of depression `45^@` on the other side of the bridge. Find the speed of the train.
02:47View SolutionEach side of a cube is decreased by 50%. Calculate the ratio of the volumes of original and changed cube.
02:25View SolutionThe total surface area of a right circular cylinrical pot without lid be 2002 sq. cm. If the radius of the base be 7cm. Find the quantity of water in litres contained in the pot. (1 litre = 1000 `cm^3`)
04:50View SolutionA tank of length 21 dcm, breadth 11 dcm and 6 dcm deep is half filled with water. If 100 solid iron balls of diameter 21 cm are completely immersed in the tank, then how much dcm of water level is raised?
05:58View SolutionFind the median of given data :
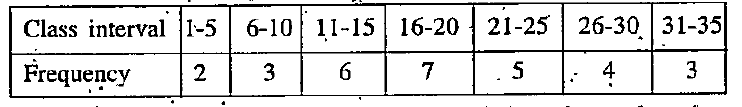 01:49View Solution
01:49View Solution
Exercise questions and complete solutions for chapter QUESTION PAPER 2018 of UNITED BOOK HOUSE of Class 10
