Complete Solutions to EXERCISE of chapter GARDEN REACH BIHARI DAS GIRL'S of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | GARDEN REACH BIHARI DAS GIRL'S |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 55 |
| Academic Year | 2023-24 |
If the ration of principal and amount in yearaly is 25:28 then rate of interest per annum is
01:59View SolutionIf one of the root of the quadratic equation `ax^2+bx+c=0,(a!=0)` be zero then
01:48View SolutionIf two circle do not intersect or touch each other, then the maximum number of common tangents can be drawn is
01:35View SolutionIf `sin theta = cos theta` then `2 theta`=
01:43View SolutionIf two cubes of length of each side `2sqrt6` cm are placed side by side, then the length of the diagonal of the cuboid so produced is ___
02:11View SolutionIf the mean of 6,7,x,8,y,16,16 is 9, then.
01:39View SolutionFill in the blanks
The compound interest and simple interest for ________year at the fixed rate of interest of fixed sum of money are equal.02:40View SolutionIf in a quadratic equation `ax^2 + bx + c = 0 (a le 0) b^2 = 4ac`, then the roots of the equation will be real and _________.
02:58View SolutionIf the length of the sides of two triangles are in proportion, then two triangles are ______________
03:14View SolutionFill in the blanks
If `cos^2theta-sin^2 theta = 1/x` (x>1), then `cos^4 theta- sin^4 theta`=___.01:10View SolutionThe shape of a pencil with one end sharpened is the combination of a cylinder and a __________
01:29View SolutionFill in the blanks
Median of the data 2,3,4,5,6is______.01:18View SolutionWrite True or False:
The compound interest will be always less than simple inerest for some money at fixed rate of interest for fixed time.02:02View SolutionTrue or false: The compound ratio of `ab : c^2` ,`bc : a^2` and `ca : b^2` is 1 : 1.
01:33View SolutionOnly one circle can be drawn through three non-colinear points.
02:54View SolutionWrite True or False:
Value of `sin^25theta+cos^25theta = 5`02:36View SolutionWrite True or False:
By melting a solid right circular cylinder a cubioid is made. The volume of both are same.02:01View SolutionWrite True or False
Mode of 3,4,5,2,3,4,1,6,4,2 is 4.02:47View SolutionThe rate of simple interest per annum reduces from 4% to 3 3/4% and for this, a person's annual income decreases by ₨. 60. Determine the principal of that person.
02:18View SolutionIn a business capital of A is `1 1/2` times of the capital of B. If the profit share of B is Rs 1,500 at the end year, then calculate the profit share of A.
01:48View SolutionIf `(2x)/3=(4y)/5=(7z)/9` then find the value of `(4x+12y-21z)/(3y)`.
03:07View SolutionIf `x alpha yz` and `y alpha zx`, then show that `(z!=0)` is a constant.
02:06View SolutionAB is the diameter of a semicircle with length of radius 4 cm C is any point on the semicircle. If `BC = 2sqrt7` cm then find the length of AC.
06:34View SolutionThe ratio of the consequtive three angles of a cyclic quadrilateral is 1:2:3 . Calculate the measurement of 1st and 3rd angle.
01:38View SolutionIn `triangleABC`, if AB = (2a - 1) cm, AC = `2sqrt2a` cm and Bc = (2a + 1) cm, then write down the value of `triangleBAC`.
02:44View SolutionIf `tan theta = 4/3`, find the value of `(sin theta + cos theta)`.
02:51View SolutionIf x = a `sec theta` and y = b `tan theta`, then find the relation between x and y free from `theta`.
01:45View SolutionThe numerical values of total surface area and volume of a hemisphere are same. Find the length of its base radius.
01:29View SolutionThe curved surface area of a right circular cone is `sqrt(10)` times of its base area. Find the ratio of its height and the length of radius.
02:31View SolutionIn a frequency distribution, mean is 8.1, `sum f_ix_i = 132+5k` and `sumf_i = 20` . Find the value of k.
01:33View SolutionIf interest is compounded half yearly what will be the compound interest and amound on ₨. 8,000 at the rate of 10% compound interest per annum for 1 1/2 years?
03:58View SolutionA, B, C start a business jointly investing ₨. 1,80,000 in together. A gives ₨. 20,000 more than that of B and B gives ₨.20,000 more than that of C. Distribute the profit of ₨. 10,800 among them.
07:29View SolutionIf 5 times of a positive whole number is less by 3 than twice of its square, then find the numbers?
05:31View SolutionIn a garden trees are planed in rows. The number of trees in each row is 5 less than the number of rows. If the total number of trees planted be 336, then find the numbner of trees planted in each row.
07:10View SolutionSimplify : `1/(sqrt2+sqrt3)-(sqrt3+1)/(2+sqrt3)+(sqrt2+1)/(3+2sqrt2)`
06:25View SolutionIf `x/y alphax+y` and `y/x alpha x-y`, then show that `x^2-y^2`= constant.
04:15View SolutionIf `x/y= (a+2)/(a-2)` show that `(x^2-y^2)/(x^2+y^2)=(4a)/(a^2+4)`
02:25View SolutionAnswer any one : If a/b+c = b/c+a = c/a+b, then prove that each ratio is equal to 1/2 or - 1.
06:24View SolutionProve that if any straight line passing through the centre of a circle bisects any chord, which is not a diameter, then the straight line will be perpendicular on that chord.
05:33View SolutionIf two tangents are drawn to a circle from a point outside it, then the line segment joining the point of contact and the exterior point are equal and they subtend equal angles at the centre.
12:48View SolutionIn `triangleABC`, `anglaBAC = 90^@` , if CD is a median of `triangleABC`, then prove that `BC^2= CD^2+3AD^2`.
05:38View SolutionAC is a diamter of a circle with centre O, If `triangleABC` is cyclic and `OP bot AB`, then prove that OP:BC = 1:2.
04:19View SolutionConstruct an equilateral triangle with side 6 cm. Draw the in circle of this triangle.
05:49View SolutionDraw a cricle with centre at O having the length of radius 2.5 cm. Take a point P at a distance 5 cm from O. Now draw two tangents from P to the circle.
06:05View SolutionFind the value of `sin^2 pi/3-sec^2 pi/4-cosec^2 pi/4+ cot pi/4`.
01:37View SolutionIf `(sin theta) (x) = (cos theta)/(y)`, then prove that `sin theta - cos theta = (x - y)/(sqrtx^2 + y^2)`.
03:27View Solutionshow that `(1-sin^2 alpha)(1-cos^2 alpha)(1+cot^2 alpha)(1+tan^2 alpha)`= 1.
04:24View SolutionThe heights of two towers are `h_1` metre and `h_2` metre. If the angle of elevation of the top of the first tower from the foot of the 2nd tower is `60^@` and the angle of elevation of the 2nd tower from the foot of the 1st tower is 45% then prove that `h_1^2= 3h_2^2`.
05:32View SolutionFrom a point at a height of 30 metre from the water level of a lake the angle of elevation of an aeroplane is `30^@` and the angle of depression of the shadow of the aeroplane in water of the lake is `60^@`. What is the height of the aeroplane from the water surface of the lake?
09:44View SolutionEach side of a cube is decreased by 50%. Calculate the ratio of the volumes of original and changed cube.
02:25View SolutionThe curved surface area of a solid right circular cylinder is 1,320 sq.cm. If the length of base diametre of the cylinder is 14 cm then find the height of the cylinder.
02:16View SolutionIf two solid spheres with the radii of 1 cm and 6 cm lengths are melted and a hollow sphere with the thickness of 1 cm is made, calculate the outer curved surface area of the hollow sphere.
11:14View SolutionIf the median of the given data is 32, then find the value of x and y when total frequency is 100.
 05:31View Solution
05:31View SolutionFind the mean of the following frequency distribution table.
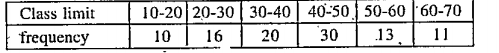 02:55View Solution
02:55View SolutionDraw an ogiven (less than type) from following data.
 04:32View Solution
04:32View Solution
EXERCISE questions and complete solutions for chapter GARDEN REACH BIHARI DAS GIRL'S of UNITED BOOK HOUSE of Class 10
