Complete Solutions to EXERCISE of chapter PROGRESSIONS of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | PROGRESSIONS |
| VGS PUBLICATION-BRILLIANT | EXERCISE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Which of these are arithmetic progressions and why?
(i) 2,3,5,7,8,10,15, ......
View SolutionWhich of these are arithmetic progressions and why?
(ii) 2,5,7,0,12,15,....
View SolutionWhich of these are arithmetic progressions and why?
(iii) `-1, -3, -5, -7,`.......
View SolutionWrite 3 more Arithmetic Progressions.
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(d) Cash prizes (in Rs.) given by a school to the toppers of classes I to XII are 200, 250, 300, 350,.......,750 respectively.
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(a) Heights (in cm) of some students of a school standing in a queue in the morning assembly are
147, 148, 149,......,157
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(b) Minimum temperatures (in degree celcius) recorded for a week, in the month of January in a city, arranged in assending order are
`-3.1, -3.0, -2.9, -2.8, -2.7, -2.6, -2.5`
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(c) The balance money (in Rs.) after paying 5% of the total loan of Rs. 1000 every month is
950, 900, 850, 800, ....,50
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(d) Cash prizes (in Rs.) given by a school to the toppers of classes I to XII are 200, 250, 300, 350,.......,750 respectively.
View SolutionThink how each of the list given above form an A.P. Discuss with your friends.
(e) Total savings (in Rs.) after every month for 10 month when Rs. 50 are saved each month are 50, 100, 150, 200, 250, 300, 350, 400,450,500.
View SolutionDraw the next two figures in the pattern above.
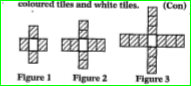
View SolutionMake a positive Arithmetic Progression in which the common difference is a small positive quantity.
View SolutionMake an A.P. in which the common difference is big (large) positive quantity.
View SolutionMake an A.P. in which the common difference is negative.
View SolutionWrite three examples for finite A.P and three for infinite A.P.
View SolutionTake any Arithmetic progression.
View SolutionAdd a fixed number to each and every term of A.P. Wrtie the resulting numbers as a list.
View SolutionSimilarly subtract a fixed number from each and every term of A.P. Write the resulting numbers as a list.
View SolutionMultiply and divide each term of A.P. by a fixed number and write the resulting numbers as a list.
View SolutionWrite the negation of the following statements and check whether the resulting statements are true,
Australia is a continent.
View SolutionYou might have touched soap water or lime water. How do you feel ? What is your conclusion?
View SolutionFor the AP: `1/4, (-1)/4, (-3)/4,(-5)/5`, .........., write the first term a and the common difference d. And find teh `7^(th)` term.
View SolutionWhich of the following forms an AP? If they form AP, then write next two terms.
(i) 4,10 ,16,22 .....
View SolutionWhich of the following forms an AP? If they form AP, then write next two terms.
(ii) `1, -1, -3 ,-5 , ...........`
View SolutionWhich of the following forms an AP? If they form AP, then write next two terms.
(iii) `-2,2,-2,2,-2, ...........`
View SolutionWhich of the following forms an AP? If they form AP, then write next two terms.
(iii) `1,1,1,2,2,2,3,3,3,........`
View SolutionWhich of the following forms an AP? If they form AP, then write next two terms.
(v) `x, 2x ,3x, 4x, ...........`
View SolutionIn which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(i) The taxi fare after each km when the fare si Rs. 20 for the first km and rises by Rs. 8 for each additional km.
View SolutionIn which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(ii) The amount of air present in a cylinder when a vacuum pump removes `1//4^(th)` of the air remaining in the cylinder at a time.
View SolutionIn which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(iii) The cost of digging a well, after every metre of digging, when it costs Rs. 150 for the first metre and rises by Rs. 50 for each subsequent metre.
View SolutionIn which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(iv) The amount of money in the account every year, when Rs. 10000 is deposited at compound intrest at `8%` per annum.
View SolutionWrite first four terms of the AP, when the first term a and the common difference d are given as follows:
(i) a = 10, d = 12.
View SolutionWrite first four terms of the AP, when the first term a and the common difference d are given as follows:
(ii) a = -2 , d = 0.
View SolutionWrite first four terms of the AP, when the first term a and the common difference d are given as follows:
(iii) a = 4, d = -3.
View SolutionWrite first four terms of the AP, when the first term a and the common difference d are given as follows:
(iv) a = -1, d = `1/2`.
View SolutionWrite first four terms of the AP, when the first term a and the common difference d are given as follows:
(v) a = -1.25, d = -0.25.
View SolutionFor the following A.Ps, write the first term and the common difference:
(i) 3,1,-1,-3,…..
View SolutionFor the following A.Ps, write the first term and the common difference:
(ii) -5,-1,3,7,…..
View SolutionFor the following A.Ps, write the first term and the common difference:
(iii) `1/3, 5/3, 9/3, 13/3….`
View SolutionFor the following A.Ps, write the first term and the common difference:
(iv) 0.6, 1.7, 2.8,3.9,…..
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(i) 2,4,8,16,……
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(ii) `2,5/2, 3, 7/2, …….`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(iii) `-1.2, -3.2, -5.2, -7.2,…..`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(iv) `-10, -6, -2, 2, …….`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(v) `3, 3+sqrt(2), 3+2sqrt(2), 3+3sqrt(2)……`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(vi) `0.2, 0.22, 0.222, 0.2222, …….`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(vii) `0, -4, -8, -12, …….`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(viii) `-1/2, -1/2, -1/2, -1/2, ……..`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(ix) 1,3,9,27,……..
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(x) `a, 2a, 3a, 4a,……..`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(x) `a, 2a, 3a, 4a,……..`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(xii) `sqrt(2), sqrt(8), sqrt(18), sqrt(32),…….`
View SolutionWhich of the following are Aps? If they form an AP, find the common difference d and write three more terms.
(xiii) `sqrt(3), sqrt(6),sqrt(9), sqrt(12), ………`
View SolutionFind the `10^(th)` term of the AP: 5,1,-3,-7 .......
View SolutionWhich term of the AP: 21,18,15, ...... Is -81? Is there any term 0? Give reason for your answer.
View SolutionDetermine the AP whose `3^(rd)` term is 5 and the `7^(th)` term is 9.
View SolutionCheck wether 301 is a term of the list of numbers 5, 11, 17, 23, .....
View SolutionHow many two-digit numbers are divisible by 3?
View SolutionFind the `11^(th)` term from the last of the A.P series given below:
A.P : 10, 7, 4, ......, -62
View SolutionA sum of Rs. 1000 is invested at 8% simple interest per year. Calculate the interest at the end of each year. Do these interests form a AP? If so, find the interest at the end of 30 years.
View SolutionIf a flower bed, there are 23 rose plants in the first row, 21 in the second, 19 in the third, and so on. There are 5 rose plants in the last row. How many rows are there in the flower bed?
View SolutionFill in the blanks in the following table, given that 'a' is the first term, d the common difference and `a_n` the `n^(th)` term of the A.P :
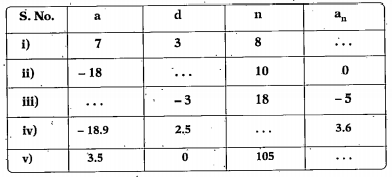
View SolutionFill in the blanks in the following table, given that 'a' is the first term, d the common difference and `a_n` the `n^(th)` term of the A.P :

View SolutionFill in the blanks in the following table, given that 'a' is the first term, d the common difference and `a_n` the `n^(th)` term of the A.P :

View SolutionFillintheblanksinthefollowingtable,giventhat'a'isthefirstterm,dthecommondifferenceand`a_n`the`n^(th)`termoftheA.P:
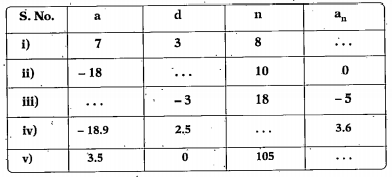
View SolutionFillintheblanksinthefollowingtable,giventhat'a'isthefirstterm,dthecommondifferenceand`a_n`the`n^(th)`termoftheA.P:
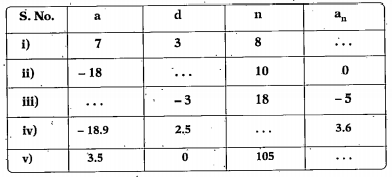
View SolutionFind the
(i) `30^(th)` term of the A.P. : 10,7,4, ……
View SolutionFind the
(ii) `11^(th)` term of the A.P. : `-3, (-1)/2, 2, …….`
View SolutionFind the respective terms for the following lowing Aps.
(i) `a_(1) = 2, a_(3) = 26` , find `a_(2)`.
View SolutionFind the respective terms for the following lowing Aps.
(ii) `a_(2) = 13, a_(4) = 26`, find `a_(1), a_(3)`.
View SolutionFind the respective terms for the following lowing Aps.
(iii) `a_(1) = 5, a_(4) = 9(1)/(2)`, find `a_(2), a_(3)`.
View SolutionFind the respective terms for the following lowing Aps.
(iv) `a_(1) = -4, a_(6) = 6`, find `a_(2),a_(3), a_(4), a_(5)`.
View SolutionFind the respective terms for the following lowing Aps.
(v) `a_(2) = 38, a_(6) = -22`, find `a_(1), a_(3), a_(4),a_(5)`.
View SolutionWhich term of the AP:
3,8,13,18,……, is 78 ?
View SolutionFind the number of terms in the following A.P.
`7, 13, 19,…..205`
View SolutionFind the number of terms in each of the following Aps :
(ii) `18, 15(1)/(2), 13, …., -47`
View SolutionCheck whether, `-150` is a term of the AP: 11,8,5,2,…..
View SolutionFind the `31^(th)` term of an A.P. whose `11^(th)` term is 38 and the `16^(th)` term is 73.
View SolutionIf the `3^(rd)` and the `9^(th)` terms of an A.P are 4 and -8 respectively, which term of this A.P is zero?
View SolutionThe `17^(th)` term of an A.P exceeds its `10^(th)` term by 7. find the common difference.
View SolutionTwo APs have the same common difference. The difference between their `100^(th)` terms is 100, what is the difference between their `1000^(th)` terms ?
View SolutionHow many three-digit numbers are divisible by 7 ?
View SolutionHow many mulitiples of 4 lie between 10 and 250?
View SolutionFor what value of n, are the `n^(th)` terms of two Aps : 63, 65,67, …. and 3, 10, 17, …… equal?
View SolutionDetermine the AP whose third term is 16 and the `7^(th)` term exceeds the `5^(th)` term by 12.
View SolutionFind the `20^(th)` term from the end of the AP : 3, 8 , 13, …..253.
View SolutionThe sum of the `4^(th)` and `8^(th)` terms of an AP is 24 and the sum of the `6^(th)` and `10^(th)` terms is 44. find the first three terms of the AP.
View SolutionSubba Rao started work in 1995 at an annual salary of Rs. 5000 and recived and increment of Rs. 200 each year. In which year did his income reach Rs. 7000 ?
View SolutionFind the sum of indicated number of terms in each of the following A.Ps.
(i) 16, 11, 6, ......, 23 terms.
View SolutionFind the sum of indicated number of terms in each of the following A.Ps.
(ii) `-0.5, -1.0, -1.5,` ....., 10 terms.
View SolutionFind the sum of indicated number of terms in each of the following A.Ps.
(iii) `-1, 1/4, 3/2,....10` terms.
View SolutionIf the sum of the first 14 terms of an AP is 1050 and its first term is 10, find the `20^(th)` term.
View SolutionHow many terms of the AP : 24, 21, 18 .... . Must be taken so that their sum is 78 ?
View SolutionFind the sum of:
(i) the first 1000 natural numbers, (ii) the first n natural numbers.
View SolutionFind the sum of : the first n natural numbers
View SolutionFind the sum of first 24 terms of the list of numbers whose `n^(th)` terms is given by `a_(n)=3+2n`
View SolutionA manufacturer of TV sets produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find:
(i) the production in the 1st yea
(ii) the production in the 10th year
(iii) the total production in first 7 years Solution :
View SolutionA manufacturer of TV sets produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find:
(i) the production in the 1st yea
(ii) the production in the 10th year
(iii) the total production in first 7 years Solution :
View SolutionA manufacturer of TV sets produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find:
(i) the production in the 1st yea
(ii) the production in the 10th year
(iii) the total production in first 7 years Solution :
View SolutionFind the sum of the following Aps,
(i) 2,7,12,…..to 10 terms.
(ii) `-37, -33, -29`, to 12 terms
(iii) 0.6, 1.7, 2.8,…… to 100 terms
(iv) `1/15, 1/12, 1/10`, ………….. To 11 terms.
View Solution
EXERCISE questions and complete solutions for chapter PROGRESSIONS of VGS BRILLIANT MATHS (TELUGU ENGLISH) of Class 10
All Exercises from VGS PUBLICATION-BRILLIANT Class 10 MATHS PROGRESSIONS
- Creative Bits for CCE Model Examination
- Creative Questions For CCE Model Examination
- Do These
- Examples
- EXERCISE
- Exercise 6.1
- Exercise 6.2
- Exercise 6.3
- Exercise 6.4
- Exercise 6.5
- Optional Exercise
- Part - A Observation Material: To solve various questions given in the public Examination(1 mark questions)
- Part - A Observation Material: To solve various questions given in the public Examination(2 mark questions)
- Part - A Observation Material: To solve various questions given in the public Examination(4 mark questions)
- Part - B Observation bits to solve various bits (Given in the public examination)
- Think and Discuss
- Try These