Complete Solutions to EXERCISE of chapter TRIGONOMETRY of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIGONOMETRY |
| VGS PUBLICATION-BRILLIANT | EXERCISE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Find tan C in the given triangle
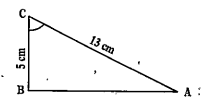
View SolutionExpress sec A and cos A in terms of sides of right angle triangle
View SolutionExpress sec A and cos A in terms of sides of right angle triangle
View SolutionDiscuss among your friends
Does `sin x = ( 4)/(3) ` exists for some value of angle?
View SolutionDiscuss among your friends
The value of sin A and cos A is always less than 1, Why?
View SolutionDiscuss among your friends
tan A is product of tan and A
View SolutionIs `(sin A )/( cos A) `equal to tan A ?
View SolutionIS `(cos A)/( sin A) ` equal to cot A ?
View SolutionIf tan A `= (3)/(4) , ` then find the other trigonometric ratio of angle A.
View SolutionIn right angle triangle ABC, 8cm ,15 cm and 17 cm are the length of AB,BC and CA respectively , Then find `sin A , cos A and tan A .`
View SolutionThe sides of a right angle triangle PQR are PQ = 7cm , PR =25 cm and ` angle Q = 90 ^(@) ` respectively , Then find tan P -tan R
View SolutionIn a right angle triangle ABC with right angle at B, in in which a=24 units, b=25 units and `/_BAC=emptyset`. Then, find `cos emptyset` and `tan emptyset `.
View SolutionIf `cos A =(12)/( 13), `then find `sin A and tan A ( A lt 90 ^(@))`
View SolutionIf 3 tan A =4 ,then find sin A and cos A
View SolutionIn `/_\ ABC` and `/_\ XYZ`, if `/_A` and `/_X` are acute angles such that cos A = cos X then show that `/_A = /_X`.
View Solution`tan theta = 7/8`అయిన `(1+sin theta)(1-sin theta) /(1+cos theta)(1-cos theta) `=
View Solution`cot theta = 7/8 ` అయిన `(1+sin theta)/cos theta` లను కనుగొనుము.
View SolutionIn a right angle triangle ABC, right angle is at B ,If tan `A=sqrt(3)` , then find the value of
(i) ` sin A cos C+ cos A sin C "
View SolutionIn a right angle triangle ABC, right angle is at B if tan `A=sqrt3` then find the value of Sin A cos C + cos a sin c
View SolutionFind the values for tan `90^(@) ,cosec 90 ^(@) , sec 90 ^(@) and cot 90 ^(@) `
View SolutionDiscuss with your friends about the following conditions:
What can you say about `cosec 0^(@) =(1)/( sin 0^(@) ) ? ` Is the defined ? Why ?
View SolutionDiscuss with your friends about the following conditions:
What can you say about `cot 0^(@) =(1)/( tan 0^(@) ) `Is it defined ? Why ?
View SolutionDiscuss with your friends about the following conditions:
`sec 0^(@) =1 `Why?
View SolutionWhat can you say about the values of sin A and cos A, as the value of angle A increases from `0^@` to `90^@`?
`A>B`, then `sin A > sin B`. Is it true?
View SolutionWhat can you say about the values of sin A and cos A, as the value of angle A increases from `0^@` to `90^@`?
If `A>B`, then `cos A > cos B`. Is it true?Discuss.
View SolutionIf one of the sides and any other part (either an acute angle or any side) of a right angle triangle is known, the remeaning sides and angles of the triangle can be determined. Do you agree ? Example with an example.
View SolutionIn `Delta ABC ` , right angle is at B,AB = 5cm and `angle ACB =30^(@) ` Determine the lengths of the sides BC and AC.
View SolutionA chord of a circle of radius 6cm is making an angle ` 60 ^(@) ` at the centre.Find the length of the chord.
View Solutionsin (A-B) = `1/2`, cos (A+B) = `1/2`,`0^(0)B` అయిన A మరియు B విలువలు కనుక్కోండి.
View SolutionEvaluate the following
` sin 45 ^(@) +cos 45^(@) `
View SolutionEvaluate the following
` (cos 45^(@))/( sec 30^(@) +cosec 60^(@)) `
View SolutionEvaluate the following
` (sin 30^(@) +tan 45^(@) -cosec 60^(@) )/( cot 45^(@)+cos 60^(@) -sec 30^(@)) `
View SolutionEvaluate the following
`2 tan ^(2) 45^(@) +cos^(2) 30 ^(@) -sin ^(2) 60^(@) `
View SolutionEvaluate the following
`(sec^(2) 60^(@) -tan ^(2) 60^(@) )/( sin ^(2) 30^(@) +cos ^(2) 30^(@) ) `
View Solution`( 2tan 30 ^(@) )/( 1+tan ^(2) 45^(@) ) =`
View Solution`( 1-tan ^(2) 45^(@) )/( 1+tan ^(2) 45^(@)) = `
View Solution`(2 tan 30^(@))/( 1-tan ^(2) 30^(@)) =`
View SolutionIs it right to say that `cos ( 60^(@) + 30 ^(@) ) = cos 60^(@) cos 30 ^(@) -sin 60^(@) sin 30 ^(@) `
View SolutionIn right angle triangle `Delta PQR ` ,right angle at Q,PQ = 6cm and `angle RPQ =60^(@) ` Determine the lengths of QR and PR.
View SolutionIn `Delta XYZ , `right angle is at Y,YZ =x, and XZ =2x Then determine `angle YXZ and angle YZX.`
View SolutionIs it right to say that `sin (A+B) =sin A +sin B` ? Justify your answer.
View SolutionCheck and discuss the above relations in the case of angles between `0^@` and `90^@` , whether they hold for these angles or not? `Sin (90^@- A) = cos A`
View SolutionCheck and discuss the below relations in the case of angles between 0° and 90° , whether they hold for these angles or not? `cos (90^@- A) = sin A`
View SolutionCheck and discuss the below relations in the case of angles between 0° and 90° , whether they hold for these angles or not? `tan (90- A) = cot A
View SolutionCheck and discuss the below relations in the case of angles between 0° and 90° , whether they hold for these angles or not? `cot (90- A) = tan A`
View SolutionCheck and discuss the above relations in the case of angles between 0° and 90° , whether they hold for these angles or not? `Sec (90^@- A) = cosec A`
View SolutionCheck and discuss the above relations in the case of angles between 0° and 90° , whether they hold for these angles or not? `cosec (90^@- A) = sec A`
View SolutionEvaluate `(sec 35^(@))/( cosec 55^(@)) `
View SolutionIf ` cos 7A =sin (A-6^(@)) ` , where 7A is an acute angle ,find the value of A .
View SolutionIf `sin A =cos B , ` then prove that A+B `=90 ^(@)`
View SolutionExpress `sin 81^(@) +tan 81^(@) `in terms of trigonometric ratio of angles between `0^(@) and 45^(@) `
View SolutionIf A , B and C are interior angles of triangle ABC ,then show that sin ` ((B+C)/( 2) )=cos (A) /(2) `
View SolutionEvaluate
` (tan 36^(@) )/( cot 54^(@)) `
View Solution`cos 12^(0)- sin 78^(0)` =
View SolutionEvaluate
`cosec 31^(@) -sec 59^(@) `
View SolutionEvaluate `sin 15^@.sec 75^@`.
View SolutionEvaluate
` tan 26^(@) tan 64^(@) `
View SolutionShow that
` tan 48^(@) tan 16^(@) tan42^(@) tan 74^(@) =1`
View SolutionShow that
` cos 36^(@) cos 54 ^(@) -sin 36^(@) sin 54^(@) =0`
View SolutionIf tan 2A `=cot (A-18^(@) ) ` where 2A is an acute angle , Find the value of A .
View SolutionIf tan A `=cot B ` where A and B are acute angles prove that `A+B= 90 ^(@) `
View SolutionIf A , B and C are interior angles of a triangle ABC, then show that `tan ((A+B) /(2)) =cot C//(2) `
View SolutionExpression `sin75^(@) +cos 65^(@) ` in terms of trigonometric ratios of angles between `0^(@) and 45^(@) `
View SolutionFor which value of an acute angle ` theta ,(i) ( cos theta )/( 1-sin theta ) +(cos theta )/( 1+sin theta ) =4 `is true ?
For which value of ` 0^(@) le theta le 90 ^(@) ,` above equation is not defined?
View SolutionAre these identities true only for `0^(@) le A le 90 ^(@) ? ` If not ,for which other values of A they are true ?
`sec^(2) A-tan ^(2) A =1 " " cosec ^(2) A - cot ^(2) A=1`
View SolutionAre these identities true only for `0^(@) le A le 90 ^(@) ? ` If not ,for which other values of A they are true ?
`sec^(2) A-tan ^(2) A =1 " " cosec ^(2) A - cot ^(2) A=1`
View SolutionIf ` sin C =( 15)/(17) , `then find ` cos C ,`
View SolutionIf ` tan x = ( 5)/( 12), ` then find sec x.
View SolutionIf ` cosec theta =(25)/( 7),` then find ` cot theta `
View SolutionEvaluate the following and justify your answer.
(i) ` (sin ^(2) 15^(@) +sin ^(2) 75^(@) )/( cos ^(2) 36^(@) +cos ^(2) 54^(@)) `
View SolutionFind ` sin 5^(@) cos 85^(@) +cos 5^(@) sin 85^(@) `
View SolutionFind ` sec 16^(@) cosec 74^(@) -cot 74^(@) tan 16^(@) `
View SolutionShow that `cot theta +tan theta =sec theta cosec theta `
View SolutionShow that ` tan ^(2) theta +tan ^(4) theta =sec ^(4) theta -sec ^(2) theta `
View SolutionProve that `sqrt( (1+costheta)/(1-costheta) )= cosectheta+cottheta.(0^@ < theta <90^@)`
View SolutionEvaluate the following
` (1+tan theta +sec theta ) ( 1+cot theta -cosec theta ) `
View Solution`(sin theta + cos theta)^(2) + (sin theta - cos theta)^(2)`=
View Solution`(sec^(2) theta -1)(cosec^(2) theta-1)`=
View SolutionShow that `(cosec theta -cot theta )^(2) =(1-cos theta )/( 1+cos theta ) `
View Solution` sqrt (( 1 + sin A ) /( 1 - sin A )) = sec A + tan A ` చూపండి.
View SolutionShow that ` (1- tan ^(2) A ) /( cot ^(2) A-1) =tan ^(2) A `
View SolutionShow that `1/cos O/-cos O/ = tan O/ cdot sinO/``(0^o < O/ <90^o) `
View SolutionSimplify sec A ` (1-sin A ) (secA+tan A) `
View SolutionProve that `( sin A+ cosec A)^2+(cos A+sec A)^2=7+tan^2 A+ cot^2 A`.
View SolutionSimplify `(1-cos theta ) (1+cos theta ) (1+ cot ^(2) theta) .`
View SolutionSimplify `(1-cos theta ) (1+cos theta ) (1+ cot ^(2) theta) .`
View SolutionIf `sec theta +tan theta =p, ` then what is the value of ` sec theta -tan theta `?
View SolutionIf `cosec theta + cot theta =k ,` then prove that `cos theta =(k^(2)-1)/( k^(2)+1)`
View SolutionProve that `(cot theta -cos theta )/( cot theta +cos theta ) =(cos ec theta -1)/( cosec theta +1) `
View SolutionProve that `(sin theta -cos theta+1 )/( sin theta + cos theta -1) =(1)/( sec theta -tan theta )` [use the identity `sec ^(2) theta =1 +tan ^(2) theta ]`
View SolutionProve that `(cosec A - sin A ) (sec A -cos A) = ( 1)/( tan A +cot A) `
View SolutionProve that `( 1+ sec A)/( sec A) =(sin ^(2) A)/( 1-cos A) `
View SolutionShow that `((1+tan ^(2) A) /(1+cot ^(2) A ) ) =((1+ tan )/( 1-cot A ))^(2)= tan ^(2) A `
View SolutionProve that `((sec A -1))/( (sec A+1) ) =((1-cos A)/((1+cos A ))) `
View Solution`tan^(2) theta - 1 / cos^(2) theta` =-1 అని చూపండి.
View SolutionExplain the meaning of cos A.
View SolutionIf ` tan O/ = sqrt3 ` (` O/ ` is acute angle) then find the value of `1+cos O/`.
View SolutionEvaluate `(sin 58^o / cos 32^o) + (tan 42^o/ cot 48^o)`
View Solution`sin A = 1 / sqrt 2` tan B =1 sin (A + B) =
View SolutionExpress ` cos O/ ` in terms of ` tan O/ `.
View Solution
EXERCISE questions and complete solutions for chapter TRIGONOMETRY of VGS BRILLIANT MATHS (TELUGU ENGLISH) of Class 10