Complete Solutions to QUESTION BANK of chapter COMPLEX NUMBERS AND QUADRATIC EQUATIONS of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | COMPLEX NUMBERS AND QUADRATIC EQUATIONS |
| A N EXCEL PUBLICATION | QUESTION BANK |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Express the following in the form a+ib (i) `(4i) ((1)/7i)`
View SolutionExpress the following in the form a+ib (ii) (2i) (-3i) `((6)/7i)`
View SolutionExpress each one of the following in the form a+ib. (i) `(5+4i)/(4+5i)`
View SolutionExpress each one of the following in the form a+ib. (i) `(isqrt-9+7i)/(1+sqrt-1)`
View SolutionProve that the complex number `(3+2i)/(2-3i) + (3-2i)/(2+3i)` is purely real
View SolutionIf `(costheta-isintheta)^2 = x -iy`, show that `x^2+y^2 = 1`
View SolutionFind the modulus of the complex number `((1+i)(2+i))/(3+i)`
View SolutionIf `(1+i)(1+2i)(1+3i)…(1+ni)=x+iy`, show that 2.5.10…`(1+n^2) = x^2+y^2`
View SolutionExpress the following complex numbers in a+ib form. (a) (5i)`(-(3)/5i)`
View SolutionExpress the following complex numbers in a+ib form. (b) `i^9+i^(19)`
View SolutionExpress the following complex numbers in a+ib form. (c ) `i^(-39)`
View SolutionExpress the following in `a+ib` form
`3(7+7i)+i(7+7i)`
View SolutionExpress the following in `a+ib` form
`(1-i)-(-1+6i)`
View SolutionExpress the following in `a+ib` form
`(1/5+i2/5)-(4+5/2i)`
View SolutionExpress the following in a+ib form. `[(1/3+i7/3)+(4+i1/3)]-[-4/3+i]`
View SolutionExpress the following in a+ib form. `(1-i)^4`
View SolutionExpress the following in `a+ib` form
`(1/3+3i)^3`
View SolutionExpress the following in a+ib form. `(-2-1/3i)^3`
View SolutionFind the multiplicative inverse of the following complex number 4-3i
View SolutionFind the multiplicative inverse of the following,
`sqrt5+3i`
View SolutionFind the multiplicative inverse of the following complex number -i
View SolutionExpress the following expression in the form a+ib `((3+isqrt5) (3-isqrt5))/((sqrt3+sqrt2i)-(sqrt3-isqrt2))`
View SolutionWrite the values of `i^2, i^4` and `i^6`
View SolutionShow that `1+i^2+i^4+i^6=0`
View SolutionUsing the value of `i^2`, prove that `1/i=-i`
View SolutionProve that `(1+i)^4(1+1/i)^4=16`
View SolutionExpress `(3-i)^2/(2+i)` in the form x+iy
View SolutionFind the conjugate of `(3-i)^2/(2+i)`
View SolutionUsing the results `abs(z^2) = absz^2` and `abs(z_1/z_2) = (abs(z_1))/abs(z_2)`, find the modulus of `(3-i)^2/(2+i)`
View SolutionExpress `(1)/(1-2i)+(3)/(1+i)` in the form x + iy
View SolutionFind the modulus of the complex number `(1/(1-2i)+3/(1+i)) ((3+4i)/(2-4i))`
View SolutionEvaluate `sqrt(-16)+3sqrt(-25)+sqrt(-36)-sqrt(-625)`
View SolutionA student writes the formula `sqrt(ab) = sqrtasqrtb`. Then he substitutes a = -1, b = -1 and finds 1 = -1. Explain where he is wrong?
View SolutionEvaluate `4x^2+8x+35`, when `x=2+sqrt-3`
View SolutionConsider the complex number `z_1 = 3+i` and `z_2 = 1+i` .What is the conjugate of `z_2`?
View SolutionConsider the complex number `z_1 = 3+i` and `z_2 = 1+i` .Find `1/z_2`
View SolutionConsider the complex number `z_1 = 3+i` and `z_2 = 1+i` .Using the value of `1/z_2`, find `(z_1)/z_2`
View SolutionProve that `abs((a+i)^2/(2a-i)) = (a^2+1)/(sqrt(4a^2+1))`
View SolutionIf `(a+i)^2/(2a-i) = p+iq`, prove that `p^2+q^2 = (a^2+1)^2/(4a^2+1)`
View SolutionIf `x=(1+i)/sqrt2`, prove that `x^2 = i`
View SolutionIf `x=(1+i)/sqrt2`.Prove that `x^6+x^4+x^2+1=0`
View SolutionProve that `abs((1+i)/(1-i)) = 1`
View SolutionIf a+ib = `sqrt((1+i)/(1-i))`, prove that `a^2+b^2=1`
View SolutionProve that `abs(costheta-isintheta) = 1`
View SolutionIf `(costheta-isintheta)^2 = x -iy`, show that `x^2+y^2 = 1`
View SolutionComplete the following table
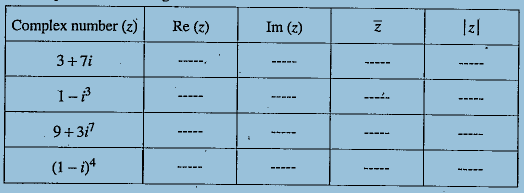
View SolutionWhen two complex numbers are equal? Find the real values of x and y for which 3x+2iy-ix+5y=7+5i
View SolutionMatch the following

View SolutionFill in the blanks by choosing the correct answer from the bracket `overline(2i^3-i^2) = ....., i^(-5)` = ….. `(1-2i, 1+2i, i,-i,1, -1)` Also express `overline(2i^3-i^2) + (12i+i^(-5)) - (overline(5-i^5))` in the form x+iy
View SolutionSuppose `z_1 = 1 - i` and `z_2 = -2 + 4i` Find `z_1z_2`
View SolutionSuppose `z_1 = 1 - i` and `z_2 = -2 + 4i` Find `overlinez_1`
View SolutionSuppose `z_1 = 1 - i` and `z_2 = -2 + 4i` Find Im `((z_1z_2)/z_1)` and Re `((z_1z_2)/z_1)`. Hence, find `abs((z_1z_2)/z_1)`
View SolutionSuppose `z_1 = 1 - i` and `z_2 = -2 + 4i` Joseph evaluted `abs((z_1z_2)/z_1)` using the following properties
(a)modulus of a quotient is the quotient of the moduli
Modulus of a product is the product of the moduli
(c) Modulus of `overlinez` =Modulus of z
Write the steps carried out by Joseph
View SolutionSuppose z = x + iy and w = `(1-iz)/(z-i)` Find 1 - iz and z - 1 in the standard form of a complex number.
View SolutionSuppose z = x + iy and w = `(1-iz)/(z-i)` Find `absw`. If `absw` = 1, prove that z is purely real
View SolutionConsider the complex number `z_1 = 2 - i` and `z_2 = -2 + i` Find `(z_1z_2)/z_1`. Hence, find `abs((z_1z_2)/z_1)`
View SolutionConsider the complex number `z_1 = 2 - i` and `z_1 = -2 + i` Raju prove that `abs((z_1z_2)/z_1) = absz_2` and using it he derived the value of `abs((z_1z_2)/z_1)`. Write the steps written by Raju.
View SolutionConsider the complex number `z_1 = 2 - i` and `z_2 = 2 + i` Find `1/(z_1z_2)`. Hence, prove than `I_m(1/(z_1z_2)) = 0`
View SolutionGiven that for any complex number z, `absz^2 = zoverlinez` . Prove that `abs(z_1+z_2)^2 = abs(z_1)^2 + 2Re(z_1overlinez_2) + abs(z_2)^2`
View SolutionGiven that for any complex number z, `absz^2 = zoverlinez` . Prove that `abs(z_1+z_2)^2 + abs(z_1-z_2)^2 = 2[abs(z_1)^2 + abs(z_2)^2]` where `z_1` and `z_2` are any two complex numbers.
View SolutionRepresent the following complex numbers as points in the argand plane 2i
View SolutionRepresent the following complex numbers as points in the argand plane 3 - i
View SolutionRepresent the following complex numbers as points in the argand plane the conjugate of 4 - i
View SolutionRepresent the complex number `z=1+i` in the polar form.
View SolutionRepresent each of the following numbers in polar form. `-sqrt3 + i`
View SolutionRepresent each of the following numbers in polar form. 4i
View SolutionFind the modulus-amplitude form of the complex number `(1+7i)/(2-i)^2`
View SolutionProve that `(cos45^@ + isin45^@)^2 = i`
View SolutionRepresent the quotient `(7(cos((3pi)/4)+isin((3pi)/4)))/(21(cos(pi/4)+isin(pi/4))` in polar form.
View SolutionShow that the points representing the complex numbers 3+2i, 2-i and -7i are collinear.
View SolutionFind the modulus and argument of the following complex number `z = -1 - isqrt3`
View SolutionRepresent each of the following numbers in polar form. `-sqrt3 + i`
View SolutionConvert the following complex numbers into polar form 1 - i
View SolutionConvert the following complex numbers into polar form -1 + i
View SolutionConvert the following complex numbers into polar form -1 -i
View SolutionConvert the following complex numbers into polar form -3
View SolutionRepresent the complex number `sqrt3+i` in the polar form.
View Solutionexpress the complex number `i` in the polar form.
View SolutionWhat is the conjugate of 2 + i?
View SolutionExpress `(2 + 3i)^2` in the form x + iy
View SolutionExpress `(2 + 3i)^2/(2+i)` in the form x + iy
View SolutionSolve `1/z + 1/(2+i) = 1/(1+3i)`
View SolutionIf `abs(a+ib) = 1` then what is the value of `a^2+b^2`?
View SolutionIf `(a^2+b^2)=1`,Prove that `(1+b+ai)/(1+b-ai)= b+ai`
View SolutionMatch the following
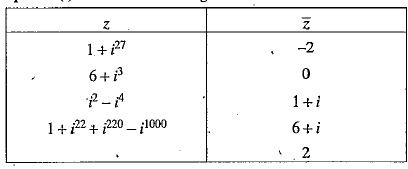
View SolutionIf `x = 4 + sqrt7i`, find `x^2` and `x^3`.Hence, find the value of `x^3-4x^2-9x+97`
View SolutionIf `(a+i)^2/(2a-i) = p+iq`, prove that `p^2+q^2 = (a+1)^2/(4a^2+1)`
View SolutionSheeba proved the same relation stated above by expressing `(a+i)^2/(2a-i)` in x+iy form. Write the steps written by Sheeba
View SolutionMatch the following
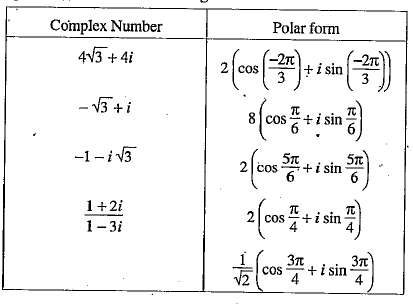
View SolutionSuppose `z_1 = 2(cos60^@ + isin60^@)` and `z_2 = 4(cos30^@ + isin30^@)` Find `z_1z_2`
View SolutionSuppose `z_1 = 2(cos60^@ + isin60^@)` and `z_2 = 4(cos30^@ + isin30^@)` find `z_1/z_2`
View SolutionSuppose `z_1 = 2(cos60^@ + isin60^@)` and `z_2 = 4(cos30^@ + isin30^@)` Find `z_1^2z_2^3`
View SolutionExpress `(1-3i)/(1+2i)` in the form x+iy
View SolutionFind the polar form of the complex number `(1-3i)/(1+2i)`
View SolutionConvert the following complex numbers into polar form 1 - i
View SolutionRepresent the complex number `sqrt3+i` in the polar form.
View SolutionExpress `(1-i)/(sqrt3+i)` in polar form
View SolutionExpress `(1-i)(sqrt3+i)` in polar form
View SolutionPlot the points represented by the complex numbers 1+i, 2+i, 2+3i, 1+3i
View SolutionWhat are the cartesian co-ordinates of the points 1+i, 2+i, 2+3i, 1+3i?
View Solution
QUESTION BANK questions and complete solutions for chapter COMPLEX NUMBERS AND QUADRATIC EQUATIONS of A N EXCEL PUBLICATION of Class 11