Complete Solutions to QUESTION BANK of chapter PERMUTATIONS AND COMBINATIONS of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | PERMUTATIONS AND COMBINATIONS |
| A N EXCEL PUBLICATION | QUESTION BANK |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
If a child can draw two kinds of faces and three kinds of hats, how many cartoons can the child produce?
View SolutionHow many four letter words can be made from the two letters A and B ?
View SolutionHow many 3 digit odd numbers can be formed by using the digits 1,2,3,4,5,6 when the repetition of digits is not allowed?
View SolutionHow many 3 digit odd numbers can be formed by using the digits 1,2,3,4,5,6 when the repetition of digits is allowed?
View SolutionHow many words (with or without meaning) of three distinct letters of the English alphabets are there?
View SolutionIn how many ways can the following prizes be given away to a class of 30 students, first and second in Mathematics, first and second in Physics, first in Chemistry and first in English?
View SolutionHow many odd numbers less than 1000 can be formed by using the digit 0,2,5,7 when the repetition of digits is allowed?
View SolutionHow many 3-digit numbers can be formed from the digits 1,2,3,4 and 5 assuming that
repetition of the digits is allowed?
View SolutionHow many 3-digit numbers can be formed from the digits 1,2,3,4 and 5 assuming that
repetition of the digits is not allowed
View SolutionHow many 3-digit even numbers can be formed from the digits 1,2,3,4,5, 6 if .the digits can be repeated?
View SolutionHow many 4-letter code can be formed using the first 10 letters of the English alphabet, if no letter can be repeated?
View SolutionHow many 5-digit telephone numbers can be constructed using the digits 0 to 9 if each number starts with 67 and no digit appears more than once?
View SolutionA coin is tossed 3 times and the outcomes are recorded. How many possible outcomes are there?
View SolutionGiven 5 flags of different colours. How many different singnals can be generated if each signal requires the use of 2 flags, one below the other?
View SolutionEvaluate `8!`
View SolutionEvaluate `4!-3!`
View SolutionIs `3!+4!`=7!` ?`
View SolutionCompute `(8!)/(6!xx2!)`
View Solutionif `1/(6!) +1/(7!) = x/(8!)` , find x.
View SolutionEvaluate `(n!)/((n-r)!)` n = 6, r = 2
View SolutionEvaluate `(n!)/((n-r)!)` n = 9, r = 5
View SolutionFind n if `3^nP_4=^(n-1)P_5`
View SolutionFind r ,if `^5P_r = 2 xx ^6P_(r-1)`
View SolutionIn how many different ways can the letters of the word HEXAGON be permuted?
View SolutionIn how many ways 4 boys and 3 girls be seated in a row of 7 chairs if the boys and girls are to be seated alternately and the boys occupy odd places.
View SolutionFind the number of all five digit numbers with distinct digits.
View SolutionHow many words can be formed out of the letters of the word “TRIANGLE”? How many of these will begin with T and end in E?
View SolutionIn flow many ways can the letters of the word “PENCIL” be arranged, so that N is always next to E?
View SolutionIn how many ways can 9 examination papers be arranged so that the best and the worst papers never come together?
View SolutionIn how many ways can 5 boys be seated at a round table?
View SolutionIn how many ways can 7 beads of different colours form a necklace?
View SolutionIf all the pemutations of the word ‘ ‘AGAIN” are arranged in a dictionary order, what is the `50^th` word?
View SolutionIn how many ways can 5 men and 4 women be seated at a round table if each woman is to be between two men?
View SolutionIf 20 persons were invited for a party, in how many ways can they and the host be seated at a circular table? In how many of these ways will two particular persons be seated on either side of the host?
View SolutionHow many 3-digit numbers can be formed by using the digits 1 to 9 if no digit is repeated?
View SolutionHow many 4 digit numbers are there with no digit repeated?
View SolutionHow many 3-digit even numbers can be made using the digits 1,2,3,4,6,7 if no digit is repeated?
View SolutionFind the number of 4-digit numbers that can be formed using the digits 1,2,3,4,5 if no digit is repeated. How many of these will be even?
View SolutionFrom a committee of 8 persons, in how many ways can we choose a chairman and a vice chairman, assuming one person can’t hold more than one position?
View SolutionFind the value of n such that
`(n-1)P_3 : nP_4=1:9`
View SolutionFind r ,if `^5P_r = 2 xx ^6P_(r-1)`
View SolutionFind the value of r if
`5P_r = 6P_(r-1)`
View SolutionHow many 8 letter words, with or without meaning, can be formed using the word EQUATION, using each letter exactly once?
View SolutionHow many words, with or without meaning that can be made from the letters of the word ‘MONDAY’, assuming that no letter is repeated if 4 letters are used at a time.
View SolutionHow many words, with or without meaning that can be made from the letters of the word ‘MONDAY’, assuming that no letter is repeated if all letters are used at a time.
View SolutionHow many words, with or without meaning that can be made from the letters of the word ‘MONDAY’, assuming that no letter is repeated if all letters are used but first letter is a vowel?
View SolutionHow many permutations are there of the 11 letters in MISSISSIPPI
all the l's not come together?
View SolutionIn how many way can the letters of the word PERMUTATIONS be arranged if the words starts with P and ends with S.
View SolutionIn how many ways can the letters of the word PERMUTATIONS be arranged in which vowels are all together
View SolutionIn how many ways can the letters of the word ‘PERMUTATIONS’ be arranged if the There are always 4 letters between P and S?
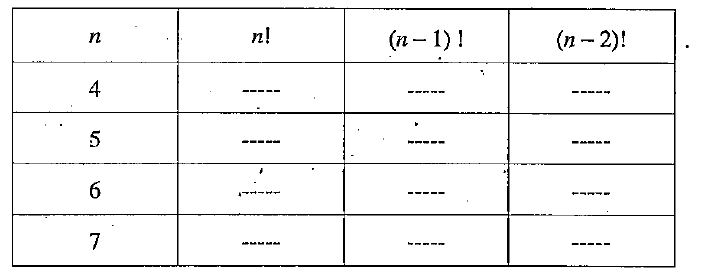
View SolutionComplete the following table
View SolutionGiven the digits 1,2,3 and 8 How many one digit numbers can be formed from the given digits?
View SolutionGiven the digits 1,2,3 and 8 How many two digit numbers can be formed using the given digits?
View SolutionGiven the digits 1,2,3 and 8 How many three digit numbers can be formed using the given digits?
View SolutionGiven the digits 1,2,3 and 8 How many four digit numbers can be formed using the given digits?
View SolutionGiven the digits 1,2,3 and 8 How many numbers can be formed from the given digits?
View SolutionGiven the digits 1,2,3 and 8 If repetition of digits is not allowed, find the number of numbers which can be formed from the given digits
View SolutionHow many 5-digit telephone numbers can be constructed using the digits 0 to 9 if each number starts with 67 and no digit appears more than once?
View SolutionGiven the digits 0,1,2,3,4, 5,6,7, 8,9 Suppose Raju is asked to form 5 - digit telephone numbers using the given digits so that no digit appears more than once. If each number ends in 89, find the number of telephone numbers constructed by Raju
View SolutionGiven the digits 3, 7, 6, 4 and 8. Seema is asked to form different numbers less than 1000 using the given digits If repetition of digits is not allowed, find the number of numbers formed by Seema
View SolutionGiven the digits 3, 7, 6, 4 and 8. Seema is asked to form different numbers less than 1000 using the given digits If repetition of digits is allowed, find the number of numbers formed by Seema
View SolutionExpress `(x+1)!` interms of `(x-1)!`. Hence, solve the equation `(x+1)! =12(x-1)!`
View SolutionExpress `(2x+1)!` in terms of `(2x-1)!,(x+2)!` in terms of `(x-1)!`.Hence, solve the equation `((2x+1)!)/((x+2)!)xx((x-1)!)/((2x-1)!)=3/5`
View SolutionWrite `(2n)! =2n(2n-1)(2n-2)` …3.2.1. Then combine the even factors and prove `(2n)! =2^n.n!.[1.3.5...(2n-1)]`
View SolutionWrite `(33)!` = [32.16.8.4.2]. [33.31.30…3.1] Hence, prove that `(33)!` is divisible by `2^15`
View SolutionSumit goes to a movie. The cinema hall has two entrances and three exits. In How many ways can Sumit enter and exit from the hall?
View SolutionIn a class there are 25 boys and 10 girls. The teacher wants to select 1 boy and 1 girl to represent the class in-a function. In how many ways can the teacher make the selection
View SolutionGiven that `p(n,r)=(n!)/((n-r)!)` What are the values of P(5, r) and P(6, r -1)?
View SolutionIf `5 xx 4P_r = 6 xx 5P_(r-1)` find 'r'.
View SolutionGiven that `P(n, r)=n(n-1)(n-2)`….to r factors What are the values of P(n, 4) and P(n, 2)?
View SolutionGiven that `P(n, r)=n(n-1)(n-2)`….to r factors If P(n, 4) = 20 P(n, 2), find n
View SolutionProve that `^9p_5+5^9p_4`=`(10!)/(5!)`
View SolutionIf `^9p_5+5^9p_4=^10p_r`.prove that r=5
View SolutionProve that `""^(2n+1)P_(n-1)=((2n+1)!)/((n+2)!)` and `""^(2n-1)P_n=((2n-1)!)/((n-1)!)`
View Solutionif `^(2n+1)p_(n-1):^(2n-1)p_n=3:5`, prove that n = 4
View SolutionIn how many ways ‘n’ objects can be arranged together in a line?
View SolutionFor a group photograph, 3 boys and 2 girls stand in a line in all possible ways. How many photos could be taken if each photo corresponds to each such arrangement
View SolutionConsider the word ‘LOGARITHMS' How many distinct letters are there in the given word?
View SolutionConsider the word ‘LOGARITHMS' How many 4 letter words, with or without meaning, can be formed out of the letters of the given word if repetition is allowed
View SolutionConsider the word ‘LOGARITHMS' How many 4 letter words, with or without meaning, can be formed out of the letters of the given word if repetition is not allowed
View SolutionConsider the word ‘RESHMA’ How many distinct letters are there in the given word?
View SolutionConsider the word ‘RESHMA’ How many vowels are there in the given word?
View SolutionConsider the word ‘RESHMA’ How many different words can be formed using the letters of the given word so that the first letter is a vowel
View SolutionConsider the word ‘RESHMA’ How many different words can be formed using the letters of the given word so that the relative positions of the vowels and the consonants remain unaltered.
View SolutionConsider the word ‘RESHMA’ How many different words can be formed using the letters of the given word so that the first letter is R and the last is A
View SolutionIn a certain city, all telephone numbers have six digits How many telephone numbers are there with distinct digits?
View SolutionIn a certain city, all telephone numbers have six digits If repetition of digits is possible, how many telephone numbers are there in the city.
View SolutionIn a certain city, all telephone numbers have six digits How many telephone numbers are there with all digits distinct and which start with 41 or 42 or 46 or 62 or 64
View SolutionSuppose that words are formed by permuting all the letters of the word ‘DHRONA’ Find the numbers of words starting with A
View SolutionSuppose that words are formed by permuting all the letters of the word ‘DHRONA’ Find the number of words starting- with DA
View SolutionIf the letters of the word DHRONA be permuted and arranged as in a dictionary, find the rank of the word.
View SolutionFind the number of arrangements of 6 boys and 5 girls in a row so that
no two girls sit together.
View SolutionFind the number of arrangements of 6 boys and 5 girls in a row so that
boys and girls occupy alternate positons
View SolutionShow that there are 300 numbers `gt` 1000 and `lt` 10,000 which can be formed with the six digits 0,1,2,3,4,5, each digit appearing not more than once in such a number
View SolutionFind the number of permutations of the letters of the word ‘DADOO’
View SolutionConsider the word DADOO. How many end with O?
View SolutionSuppose that all the letters of the word ‘HARYANA’ are permuted and words are formed. How many different words can be formed?
View SolutionSuppose that all the letters of the word ‘HARYANA’ are permuted and words are formed. How many words are there which begin with H and end with N?
View SolutionSuppose that all the letters of the word ‘HARYANA’ are permuted and words are formed. In how many words H and N are together?
View SolutionSuppose that the different permutations of the word ‘EXAMINATION’ are listed as in a dictionary. How many permutations start with A?
View Solution
QUESTION BANK questions and complete solutions for chapter PERMUTATIONS AND COMBINATIONS of A N EXCEL PUBLICATION of Class 11