Complete Solutions to Exercises Linked Comprehension of chapter IONIC EQUILIBRIUM of Class 11 book with complete answers and questions
| Class 11 | CHEMISTRY |
| Chapter | IONIC EQUILIBRIUM |
| CENGAGE CHEMISTRY | Exercises Linked Comprehension |
| Number Of Questions | 45 |
| Academic Year | 2023-24 |
`Pb(IO_(3))_(2)` is a sparingly soluble salt `(K_(sp) = 2.6 xx 10^(-13))`. To `35mL` of `0.15M Pb(NO_(3))_(2)` solution, `15mL` of `0.8M KIO_(3)` solution is added, and a precipiatte of `Pb(IO_(3))_(2)` is formed.
Which is the limiting reactant of teh reaction that takes place in the solution?
View Solution`Pb(IO_(3))_(2)` is a sparingly soluble salt `(K_(sp) = 2.6 xx 10^(-13))`. To `35mL` of `0.15M Pb(NO_(3))_(2)` solution, `15mL` of `0.8M KIO_(3)` solution is added, and a precipiatte of `Pb(IO_(3))_(2)` is formed.
What will be molarity of `Pb^(2+)` ions in the solution after completion of the reactions? `[IO3^(-)]=0.03M`
View Solution`Pb(IO_(3))_(2)` is a sparingly soluble salt `(K_(sp) = 2.6 xx 10^(-13))`. To `35mL` of `0.15M Pb(NO_(3))_(2)` solution, `15mL` of `0.8M KIO_(3)` solution is added, and a precipiatte of `Pb(IO_(3))_(2)` is formed.
What will be molarity of `Pb^(2+)` ions in the solution after completion of the reactions? `[IO3^(-)]=0.03M`
View SolutionAcid-base indicator such as methy`1` orange, phenolphthalein, and bromothymol blue ate substances which change colour accroding to the hydrogen ion concentration of the solution to which they are added.
Most indicators are weak acids (or more rarely weak base) in which the undissociated and dissociated forms have different and distinct colours. If methy`1` orange is used as the examples and the un-dissociated forms is written as `HMO`, then dissociation occurs as shown below:
Reaction: `{:(HMOhArr,H^(o+)+,MO^(Theta),,),(Red,"Colourless","Yellow",,):}`
The indicator should have a sharp colour change with the equivalence point of the titration. Usually the colour change of the indicator occurs over a range of about two `pH` units. It should be noted that the eye cannot detect the exact end point of the tiytration. The `pK_(a)` of the indicator should be near the `pH` of the solution at the equivalance point.
Which of the following sitution exists at the equivalence point of titration?
View SolutionIf methyl orange is used as a solution at pH = 2 containing the indicator would be
View SolutionAcidic solution is defined as a solution whose `[H^(o+)] gt [overset(Theta)OH]`. Base solution has `[overset(Theta)OH] gt [H^(o+)]`. During acid-base titrations, `pH` of the mixture will change depending on the amount base added. This variation is shown in the form of graph by making plot as titration curves `100mL` of `1.0 M H_(3)A (K_(a_(1)) = 10^(-3), K_(a_(2)) = 10^(-5), K_(a_(3)) = 10^(-7))` is titrated against `0.1M NaOh`. The titration curve is as follows.

What is the `pH` at point `A?
View SolutionAcidic solution is defined as a solution whose `[H^(o+)] gt [overset(Theta)OH]`. Base solution has `[overset(Theta)OH] gt [H^(o+)]`. During acid-base titrations, `pH` of the mixture will change depending on the amount base added. This variation is shown in the form of graph by making plot as titration curves `100mL` of `1.0 M H_(3)A (K_(a_(1)) = 10^(-3), K_(a_(2)) = 10^(-5), K_(a_(3)) = 10^(-7))` is titrated against `0.1M NaOh`. The titration curve is as follows.

What is the `pH` at point `A?
View SolutionAcidic solution is defined as a solution whose `[H^(o+)] gt [overset(Theta)OH]`. Base solution has `[overset(Theta)OH] gt [H^(o+)]`. During acid-base titrations, `pH` of the mixture will change depending on the amount base added. This variation is shown in the form of graph by making plot as titration curves `100mL` of `1.0 M H_(3)A (K_(a_(1)) = 10^(-3), K_(a_(2)) = 10^(-5), K_(a_(3)) = 10^(-7))` is titrated against `0.1M NaOh`. The titration curve is as follows.

What will be the change in `pH` from point `B` to point `C?`
View SolutionIn equalitative analysis, cations of graph `II` as well as group `IV` both are precipitated in the form of sulphides. Due to low value of `K_(sp)` of group `II` sulphides, group reagent is `H_(2)S` in the presence of dil. `HC1`, and due to high value of `K_(sp)` of group `IV` sulphides, group reagent is `H_(2)S` in the presence of `NH_(4)OH` and `NH_(4)C1`. In a solution containing `0.1M` each of `Sn^(2+), Cd^(2+)`, and `Ni^(2+)` ions, `H_(2)S`gas is passed.
`K_(sp) of SnS = 8 xx 10^(-29), K_(sp) of CdS = 15 10^(-28), K_(sp) of NiS - 3 xx 10^(-21), K_(1) of H_(2)S = 1 xx 10^(-7), K_(2) of H_(2)S = 1 xx 10^(-14)`
If `H_(2)S` is passed into the above mixture in the presence of `HC1`, which ion will be precipitated first?
View SolutionIn equalitative analysis, cations of graph `II` as well as group `IV` both are precipitated in the form of sulphides. Due to low value of `K_(sp)` of group `II` sulphides, group reagent is `H_(2)S` in the presence of dil. `HC1`, and due to high value of `K_(sp)` of group `IV` sulphides, group reagent is `H_(2)S` in the presence of `NH_(4)OH` and `NH_(4)C1`. In a solution containing `0.1M` each of `Sn^(2+), Cd^(2+)`, and `Ni^(2+)` ions, `H_(2)S`gas is passed.
`K_(sp) of SnS = 8 xx 10^(-29), K_(sp) of CdS = 15 10^(-28), K_(sp) of NiS - 3 xx 10^(-21), K_(1) of H_(2)S = 1 xx 10^(-7), K_(2) of H_(2)S = 1 xx 10^(-14)`
At what value of `pH, NiS` will start to precipitate?
View SolutionIn equalitative analysis, cations of graph `II` as well as group `IV` both are precipitated in the form of sulphides. Due to low value of `K_(sp)` of group `II` sulphides, group reagent is `H_(2)S` in the presence of dil. `HC1`, and due to high value of `K_(sp)` of group `IV` sulphides, group reagent is `H_(2)S` in the presence of `NH_(4)OH` and `NH_(4)C1`. In a solution containing `0.1M` each of `Sn^(2+), Cd^(2+)`, and `Ni^(2+)` ions, `H_(2)S`gas is passed.
`K_(sp) of SnS = 8 xx 10^(-29), K_(sp) of CdS = 15 10^(-28), K_(sp) of NiS - 3 xx 10^(-21), K_(1) of H_(2)S = 1 xx 10^(-7), K_(2) of H_(2)S = 1 xx 10^(-14)`
Which of the following sulphides is more soluble in pure water?
View SolutionIn equalitative analysis, cations of graph `II` as well as group `IV` both are precipitated in the form of sulphides. Due to low value of `K_(sp)` of group `II` sulphides, group reagent is `H_(2)S` in the presence of dil. `HC1`, and due to high value of `K_(sp)` of group `IV` sulphides, group reagent is `H_(2)S` in the presence of `NH_(4)OH` and `NH_(4)C1`. In a solution containing `0.1M` each of `Sn^(2+), Cd^(2+)`, and `Ni^(2+)` ions, `H_(2)S`gas is passed.
`K_(sp) of SnS = 8 xx 10^(-29), K_(sp) of CdS = 10^(-28), K_(sp) of NiS - 3 xx 10^(-21)`
`K_(1) of H_(2)S = 1 xx 10^(-7), K_(2) of H_(2)S = 1 xx 10^(-14)`
If `0.1M HC1` is mixed in the solution containing only `0.1 M Cd^(2+)` ions and saturated with `H_(2)S`, then `[Cd^(2+)]` remaining in the solution after `CdS` stopes to precipitate is:
View SolutionThe degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
`alpha = sqrt((K_(a))/(c))` As the tempertaure increases, degree of dissociation will increase.
`(alpha_(1))/(alpha_(2)) = sqrt((K_(a_(1)))/(K_(a_(2))))` if concentration is same.
`(alpha_(1))/(alpha_(2)) = sqrt((c_(2))/(c_(1)))` if acid is same.
`0.01M CH_(3)COOH` has `4.24%` degree of dissociation, the degree of dissociation of `0.1M CH_(3)COOH` will be
View SolutionThe degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
`alpha = sqrt((K_(a))/(c))` As the tempertaure increases, degree of dissociation will increase.
`(alpha_(1))/(alpha_(2)) = sqrt((K_(a_(1)))/(K_(a_(2))))` if concentration is same.
`(alpha_(1))/(alpha_(2)) = sqrt((c_(2))/(c_(1)))` if acid is same.
`pH` of `0.005 M HCOOH [K_(a) = 2 xx 10^(-4)]` is equal to
View SolutionThe degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
`alpha = sqrt((K_(a))/(c))` As the tempertaure increases, degree of dissociation will increase.
`(alpha_(1))/(alpha_(2)) = sqrt((K_(a_(1)))/(K_(a_(2))))` if concentration is same.
`(alpha_(1))/(alpha_(2)) = sqrt((c_(2))/(c_(1)))` if acid is same.
`a_(1)` and `a_(2)` are in ratio of `1:2, K_(a_(1)) = 2xx10^(-4)`. What will be `K_(a_(2))`?
View SolutionThe following solutions are mixed: `500mL of 0.01 M AgNO_(3)` and `500mL` solution that was both `0.01M` in `NaCI` and `0.01M` in `NaBr`. Given `K_(sp) AGCI = 10^(-10), K_(sp) AgBr = 5 xx 10^(-13)`.
Calculate the `[CI^(Theta)]` in the equilibrium solution.
View SolutionThe following solutions are mixed: `500mL of 0.01 M AgNO_(3)` and `500mL` solution that was both `0.01M` in `NaCI` and `0.01M` in `NaBr`. Given `K_(sp) AGCI = 10^(-10), K_(sp) AgBr = 5 xx 10^(-13)`.
Calculate the `[Ag^(o+)]` in the equilibrium solution.
View SolutionThe following solutions are mixed: `500mL of 0.01 M AgNO_(3)` and `500mL` solution that was both `0.01M` in `NaCI` and `0.01M` in `NaBr`. Given `K_(sp) AGCI = 10^(-10), K_(sp) AgBr = 5 xx 10^(-13)`,`[Ag^(+)] = 2 xx 10^(-8)`.
Calculate the `[Br^(Theta)]` in the equilibrium solution.
View SolutionWhen `1.5mol` of `CuCI_(2).2H_(2)O` is dissolved in enough water to make `1.0L` of solution.
Given: `K_(f)CuCI^(Theta) 1.0 (K_(f)` is the formation constant of `CuCi^(o+))`
`[Cu^(2+)]` in solution is
View SolutionWhen `1.5mol` of `CuCI_(2).2H_(2)O` is dissolved in enough water to make `1.0L` of solution.
Given: `K_(f)CuCI^(Theta) 1.0 (K_(f)` is the formation constant of `CuCi^(o+))`
`[CI^(Theta)]` in solution is
View SolutionWhen `1.5mol` of `CuCI_(2).2H_(2)O` is dissolved in enough water to make `1.0L` of solution.
Given: `K_(f)CuCI^(Theta) 1.0 (K_(f)` is the formation constant of `CuCi^(o+))`
`[CiCI^(o+)]` in solution is
View SolutionAcid rain takes place dur to combination of acidic oxides with water and it is an environmental concern all over the world. Assuming rain water is uncontaminated with `HNO_(3)` or `H_(2)SO_(4)` and is in equilibrium with `1.25 xx 10^(-4) atm CO_(2)`. The Henry's law constant `(K_(H))` is `1.25 xx 10^(6) "torr". K_(a_(1))` of `H_(2)CO_(3) = 4.3 xx 10^(-7)`
What is the `pH` of neutral rain water ?
View SolutionAcid rain takes place dur to combination of acidic oxides with water and it is an envirronmental concern all over the world. Assuming rain water is uncontaminated with `HNO_(3)` or `H_(2)SO_(4)` and is in equilibrium with `1.25 xx 10^(-4)` atm `CO_(2)`. The Henry's law constant `(K_(H))` is `1.25 xx 10^(6) "torr". K_(a_(1))` of `H_(2)CO_(3) = 4.3 xx 10^(-7)`
Given : `K_(f)CuCI^(Theta) = 1.0 (K_(f)` is formation constant of `CuCI^(o+))`
If `SO_(2)` content is the atomsphere is `0.64 "ppm"` by volume, `pH` of rain water is (assume `100%` ionisation of acid rain as monobasic acid).
View SolutionIn atmosphere, `SO_(2)` and `NO` are oxidised to `SO_(3)` and `NO_(2)`, respectively,w hcih react with water to given `H_(2)SO_(4)` and `HNO_(3)`. The resultant solution is called acid rain. `SO_(2)`dissolves in water to form diprotic acid.
`SO_(2)(g) +H_(2)O(l) hArr HSO_(3)^(Theta) + H^(o+), K_(a_(1)) = 10^(-2)`.
`HSO_(3)^(Theta) hArr SO_(3)^(2-) + H^(o+), K_(a_(2)) = 10^(-7)`
and for equilibrium,
`SO_(2)(aq) + H_(2)O (l) hArr SO_(3)^(2-)(aq) +2H^(o+)(aq)`
`K_(a) = K_(a_(1)) xx K_(a_(2)) = 10^(-9) at 300K`.
Which of the following reagnets will given white precipitate with the aqueous solution of sulphurous acid?
View SolutionIn atmosphere, `SO_(2)` and `NO` are oxidised to `SO_(3)` and `NO_(2)`, respectively,w hcih react with water to given `H_(2)SO_(4)` and `HNO_(3)`. The resultant solution is called acid rain. `SO_(2)`dissolves in water to form diprotic acid.
`SO_(2)(g) +H_(2)O(l) hArr HSO_(3)^(Theta) + H^(o+), K_(a_(1)) = 10^(-2)`.
`HSO_(3)^(Theta) hArr SO_(3)^(2-) + H^(o+), K_(a_(2)) = 10^(-7)`
and for equilibrium,
`SO_(2)(aq) + H_(2)O (l) hArr SO_(3)^(2-)(aq) +2H^(o+)(aq)`
`K_(a) = K_(a_(1)) xx K_(a_(2)) = 10^(-9) at 300K`.
The `pH` of `0.01M` aqueous solutioon of sodium sulphite `(Na_(2)SO_(3))`
View SolutionIn atmosphere, `SO_(2)` and `NO` are oxidised to `SO_(3)` and `NO_(2)`, respectively,w hcih react with water to given `H_(2)SO_(4)` and `HNO_(3)`. The resultant solution is called acid rain. `SO_(2)`dissolves in water to form diprotic acid.
`SO_(2)(g) +H_(2)O(l) hArr HSO_(3)^(Theta) + H^(o+), K_(a_(1)) = 10^(-2)`.
`HSO_(3)^(Theta) hArr SO_(3)^(2-) + H^(o+), K_(a_(2)) = 10^(-7)`
and for equilibrium,
`SO_(2)(aq) + H_(2)O (l) hArr SO_(3)^(2-)(aq) +2H^(o+)(aq)`
`K_(a) = K_(a_(1)) xx K_(a_(2)) = 10^(-9) at 300K`.
The dominant equilibrium in an aqueous solution of sodium hydrogen sulphite `(NaHSO_(3))` is
`2HSO_(3)^(Theta) (aq) hArr SO_(2) (aq) +SO_(3)^(2-) (aq) + H_(2)O(l)`
The equilibrium constant for the above reaction is
View SolutionIn atmosphere, `SO_(2)` and `NO` are oxidised to `SO_(3)` and `NO_(2)`, respectively,w hcih react with water to given `H_(2)SO_(4)` and `HNO_(3)`. The resultant solution is called acid rain. `SO_(2)`dissolves in water to form diprotic acid.
`SO_(2)(g) +H_(2)O(l) hArr HSO_(3)^(Theta) + H^(o+), K_(a_(1)) = 10^(-2)`.
`HSO_(3)^(Theta) hArr SO_(3)^(2-) + H^(o+), K_(a_(2)) = 10^(-7)`
and for equilibrium,
`SO_(2)(aq) + H_(2)O (l) hArr SO_(3)^(2-)(aq) +2H^(o+)(aq)`
`K_(a) = K_(a_(1)) xx K_(a_(2)) = 10^(-9) at 300K`.
Which of the following statement is correct?
View SolutionIn acid-base titration react rapidly to neutralise each other. Equivalence point is a point at which the acid and the base (or oxidising agent and reducing agent) have beem added in equivalent quantities. The end point in the point at which the titration stops. since the purpose of the indicator is to stop the titration close to the point at which the acid and base were added in equivalent quantities, it is important that the equivalent point and the end point be as close as must change colour at a `pH` close to that of a solution of the salt of the acid base. Singificantly, the `pH` changes most rapidly near the equivalent point. The exact shape of a titration curve depends on `K_(a)` and `K_(b)` of acid and base.

The following curve represents titration curve of `HCI` against `KOH`. The `pH` at equivalent point is
Examine the titration curve below and answer the question.
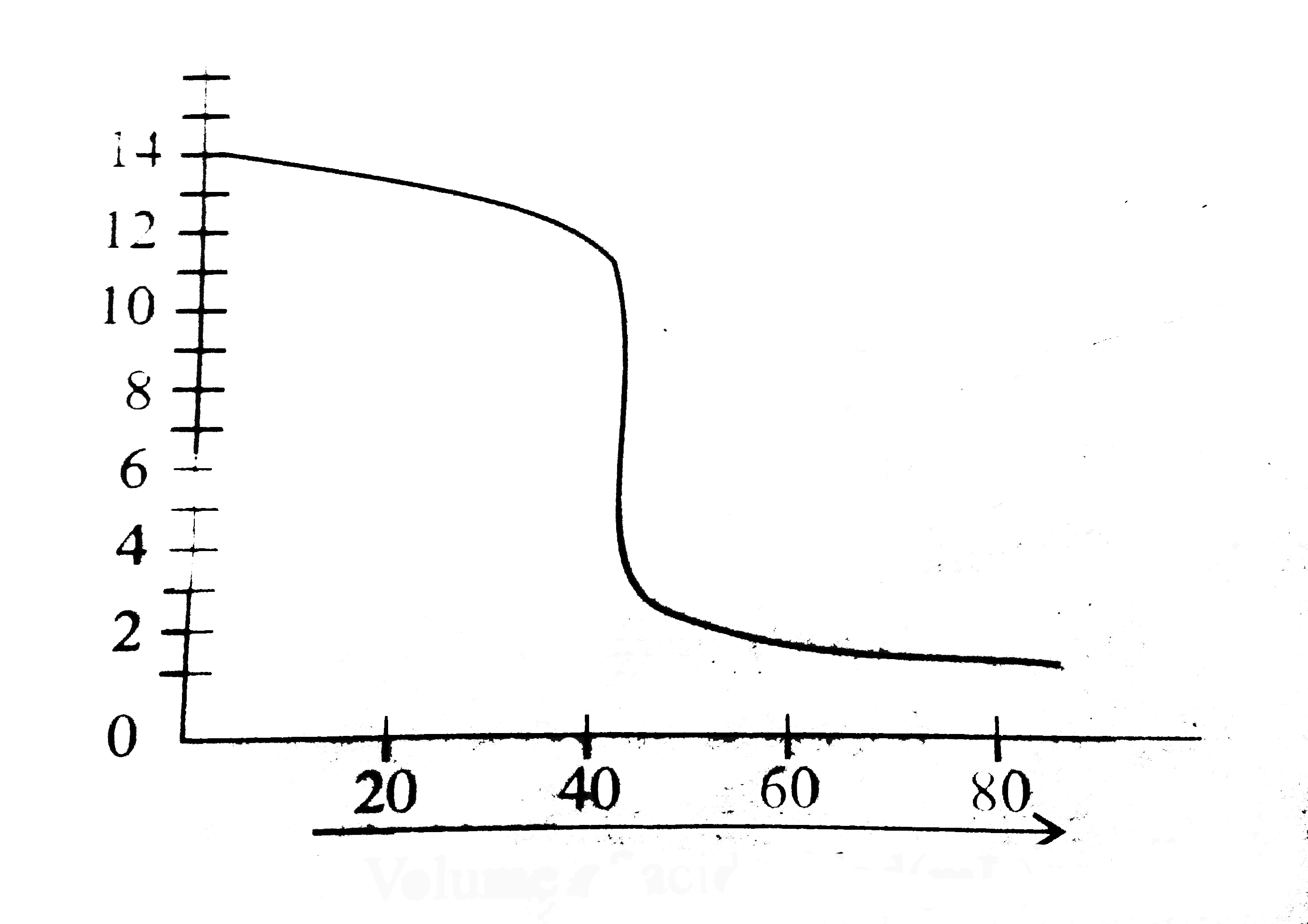
View SolutionIn acid-base titration react rapidly to neutralise each other. Equivalence point is a point at which the acid and the base (or oxidising agent and reducing agent) have beem added in equivalent quantities. The end point in the point at which the titration stops. since the purpose of the indicator is to stop the titration close to the point at which the acid and base were added in equivalent quantities, it is important that the equivalent point and the end point be as close as must change colour at a `pH` close to that of a solution of the salt of the acid base. Singificantly, the `pH` changes most rapidly near the equivalent point. The exact shape of a titration curve depends on `K_(a)` and `K_(b)` of acid and base.

The curve represents the titration of
View Solution
The suitable indicator for the titration is
View SolutionIn acid-base titration react rapidly to neutralise each other. Equivalence point is a point at which the acid and the base (or oxidising agent and reducing agent) have beem added in equivalent quantities. The end point in the point at which the titration stops. since the purpose of the indicator is to stop the titration close to the point at which the acid and base were added in equivalent quantities, it is important that the equivalent point and the end point be as close as must change colour at a `pH` close to that of a solution of the salt of the acid base. Singificantly, the `pH` changes most rapidly near the equivalent point. The exact shape of a titration curve depends on `K_(a)` and `K_(b)` of acid and base.

The `pH` at equivalence point is
View SolutionIn acid-base titration react rapidly to neutralise each other. Equivalence point is a point at which the acid and the base (or oxidising agent and reducing agent) have beem added in equivalent quantities. The end point in the point at which the titration stops. since the purpose of the indicator is to stop the titration close to the point at which the acid and base were added in equivalent quantities, it is important that the equivalent point and the end point be as close as must change colour at a `pH` close to that of a solution of the salt of the acid base. Singificantly, the `pH` changes most rapidly near the equivalent point. The exact shape of a titration curve depends on `K_(a)` and `K_(b)` of acid and base.

Which of the following curves indicates the titration of a weak diprotic acid by `KOH` of equivalent strength?
View SolutionPhysical and chemical equilibrium can respond to a change in their pressure, temperature, and concentration of reactants and products. To describe the change in the equilibrium we have a principle named Le Chatelier principle. According to this principle, even if we make some changes in equilibrium, then also the system even re-establishes the equilibrium by undoing the effect.
In the reaction `N_(2)(g)+3H_(2)(g) hArr 2NH_(3)(g)`. If we increase the pressure of the system, the equilibrium is
View SolutionPhysical and chemical equilibrium can respond to a change in their pressure, temperature, and concentration of reactants and products. To describe the change in the equilibrium we have a principle named Le Chatelier principle. According to this principle, even if we make some changes in equilibrium, then also the system even re-establishes the equilibrium by undoing the effect.
If we add `SO_(4)^(2-)` ion to a saturated solution of `Ag_(2)SO_(4)`, it will result in a//an
View SolutionPhysical and chemical equilibria can respond to a change in their pressure, temperature, and concentration of reactants and products. To describe the change in the equilibrium, we have a principle named Le Chatelier's principle. This we can define in terms of energy, as the free energy change in equilibrium is zero means the system is stable. So if we are doing some changes in equilibrium, then the system having a tendency to reestablish the equilibrium by undoing the effect we brought. Consider the following equilibrium.
Three sparingly soluble salts `A_(2)B, AB`, and `AB_(3)` are given. If all the three having the same value of solubility products `(K_(sp))`, in the saturated solution, the correct order of their solubilites is
View Solution`H_(3)PO_(4)` is a tribasic acid with `pK_(a_(1)), pK_(a_(2))` and `pK_(a_(3)) 1.12, 7.21`, and `12.32`, respectively. It is used in fertiliser productions and its various salts are used in food, detrgent, toothpaste, and in metal treatment.
Small quantities of `H_(3)PO_(4)` are used in inparting the sour or tart taste of soft drinkes, such as Coca Cola, and beers, in which `H_(3)PO_(4)` is prese4nt `0.05%` by weight (density `= 1.0 gmL^(-1))`.
`10^(-3)M H_(3)PO_(4)(pH = 7)` is used in fertilisers as an aqueous soil digesting. Plants can absorb zinc in whater soluble from only. Zinc phosphate is the source of zinc and `PO_(4)^(3-`) ions in the soil. `K_(sp)` of zinc phosphate `= 9.1 xx 10^(-33)`.
Calculate the `pH` of a Coca Cola, assuming that the acidity of the cola arises only from `H_(3)PO_(4)` and `K_(a_(2))` and `K_(a_(3))` are no importance.
View Solution`H_(3)PO_(4)` is a tribasic acid with `pK_(a_(1)), pK_(a_(2))` and `pK_(a_(3)) 1.12, 7.21`, and `12.32`, respectively.`10^(-3)M H_(3)PO_(4)(pH = 7)` is used in fertilizers as an aqueous soil digesting. Plants can absorb zinc in water soluble from only. Zinc phosphate is the source of zinc and `PO_(4)^(3-`) ions in the soil. `K_(sp)` of zinc phosphate `= 9.1 xx 10^(-33)`.
`[PO_(4)^(3-)]` ion in the soil with `pH = 7`, is
View Solution`H_(3)PO_(4)` is a tribasic acid with `pK_(a_(1)), pK_(a_(2))` and `pK_(a_(3)) 1.12, 7.21`, and `12.32`, respectively. 10^(-3)M 'H_(3)PO_(4)'(pH = 7)` . Ksp of Zinc phosphate= 9.1 xx 10^(-33)`.
`[Zn^(2+)]` ion in the soil is
View SolutionThe number of moles of `KMnO_(4)` required to oxidise `1 mol` of `Fe(C_(2)O_(4))` in acidic medium is
View SolutionAqueous solutions of `Na_(2)C_(2)O_(4)` and `CaCI_(2)` are mixed and precipitate of `CaC_(2)O_(4)` formed is filered and dried. `250 mL` of the saturated solution of `CaC_(2)O_(4)` required `6.0 mL` of `0.001M KMnO_(4)` solution in acidic medium for complete titration.
Equivalent of `KMNO_(4)` required in the titration and equivalent of `C_(2)O_(4)^(2-)` ion present in `CaC_(2)O_(4)`, respectively, are
View SolutionAqueous solutions of `Na_(2)C_(2)O_(4)` and `CaCI_(2)` are mixed and precipitate of `CaC_(2)O_(4)` formed is filered and dried. `250 mL` of the saturated solution of `CaC_(2)O_(4)` required `6.0 mL` of `0.001M KMnO_(4)` solution in acidic medium for complete titration.
`K_(sp)` of `CaC_(2)O_(4)` is
View SolutionAqueous solutions of `Na_(2)C_(2)O_(4)` and `CaCI_(2)` are mixed and precipitate of `CaC_(2)O_(4)` formed is filered and dried. `250 mL` of the saturated solution of `CaC_(2)O_(4)` required `6.0 mL` of `0.001M KMnO_(4)` solution in acidic medium for complete titration.
Which is the indicator in the above titration?
View SolutionHin is an acidic indicator `(K_(Ind) =10^(-7))` which dissociates into aqueous acidic solution of `30mL` of `0.05M H_(3)PO_(4) (K_(1) = 10^(-3), K_(2) = 10^(-7), K_(3) = 10^(-13))`
Calculate the `[(Ind^(Theta))/(Hin)]`
View SolutionHin is an acidic indicator `(K_(Ind) =10^(-7))` which dissociates into aqueous acidic solution of `30mL` of `0.05M H_(3)PO_(4) (K_(1) = 10^(-3), K_(2) = 10^(-7), K_(3) = 10^(-13))`
If Hin and `Ind^(Theta)` posses colour `P` and `Q`, respectively, and concentration of `HIn` is `120` times than that of `Ind^(Theta)`. colour `Q` predominates over `P` when concnetration of `Ind^(Theta)` is `127` times of `HIn`.
What is the `pH` range of the indicator.
View Solution`Hin` is an acidic indicator `(K_(Ind) =10^(-7))` which dissociates into aqueous acidic solution of `30mL` of `0.05M H_(3)PO_(4) (K_(1) = 10^(-3), K_(2) = 10^(-7), K_(3) = 10^(-13))`
If this solution is treated with `30mL`of `NaOH` solution, then what molarity of `NaOH` is needed to reach the equivalence point with indicator?
View Solution
Exercises Linked Comprehension questions and complete solutions for chapter IONIC EQUILIBRIUM of CENGAGE CHEMISTRY (ENGLISH) of Class 11
All Exercises from CENGAGE CHEMISTRY Class 11 CHEMISTRY IONIC EQUILIBRIUM
- Archives Fill In The Blanks
- Archives Integer
- Archives Multiple Correct
- Archives Single Correct
- Archives Subjective
- Archives True/False
- Ex 8.1
- Ex 8.2
- Ex 8.3
- Ex 8.4
- Ex 8.5
- Exercises Assertion-Reasoning
- Exercises Integer
- Exercises Linked Comprehension
- Exercises Multiple Correct
- Exercises Single Correct
- Exercises Subjective (Buffer Solutions)
- Exercises Subjective (Hydrolysis Of Salt)
- Exercises Subjective (Polyprotic Acid)
- Exercises Subjective (Weak Acid And Weak Bases)
- Exercises Subjective(Coordination Equilibria)
- Exercises True/ False
- Solved Examples