Complete Solutions to Sample Questions for Competitive Exams of chapter MATHEMATICAL INDUCTION of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MATHEMATICAL INDUCTION |
| CHHAYA PRAKASHINI | Sample Questions for Competitive Exams |
| Number Of Questions | 20 |
| Academic Year | 2023-24 |
If for all `ninNN` and `nge1`, then `(3^(2^(n))-1)` is always divisible by
View SolutionIf `ninNN` , both expression `n(n+1)(n+2)` and `n(n+1)(n+5)` are multiple of -
View SolutionThe value of n for which `n!gt2^(n)` will true, are -
View SolutionIf n is even then the expression `n(n^(2)+20)` is divisible by the numbers, are -
View SolutionIf `p inNN` then the expression `p^(n+1)+(p+1)^(2n-1)` is divisible by the expressions are -
View Solution`10^(n)+3*4^(2)+5` is always divisible by the number -
View Solution`3^(2n)` when divided by 8 leves the remainder-
View SolutionIf n be a positive integer, then the digit in the unit's place of `3^(2n-1)+2^(2n-1)` is -
View SolutionFor a positive integer `n,n(n+1)(2n+1)` when divided by 6 leaves the remainder -
View Solution`ninNN,(n+1)^(3)+(n+2)^(3)+(n+3)^(3)` when divided by 9, then the remainder will be -
View SolutionMatch the entries given in left column with those given in right column.
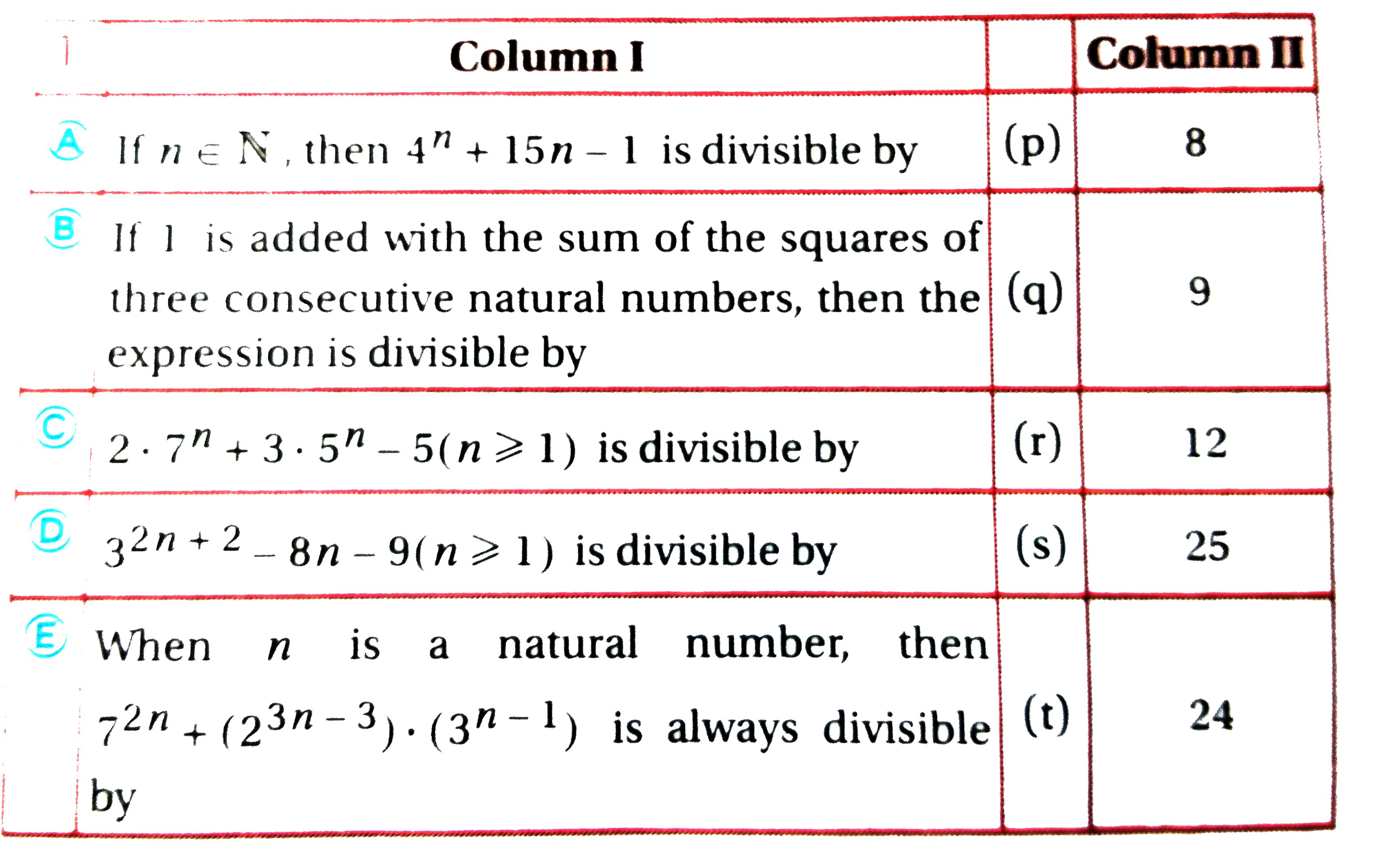
View SolutionMatch the entries given in left column with those given in right column.
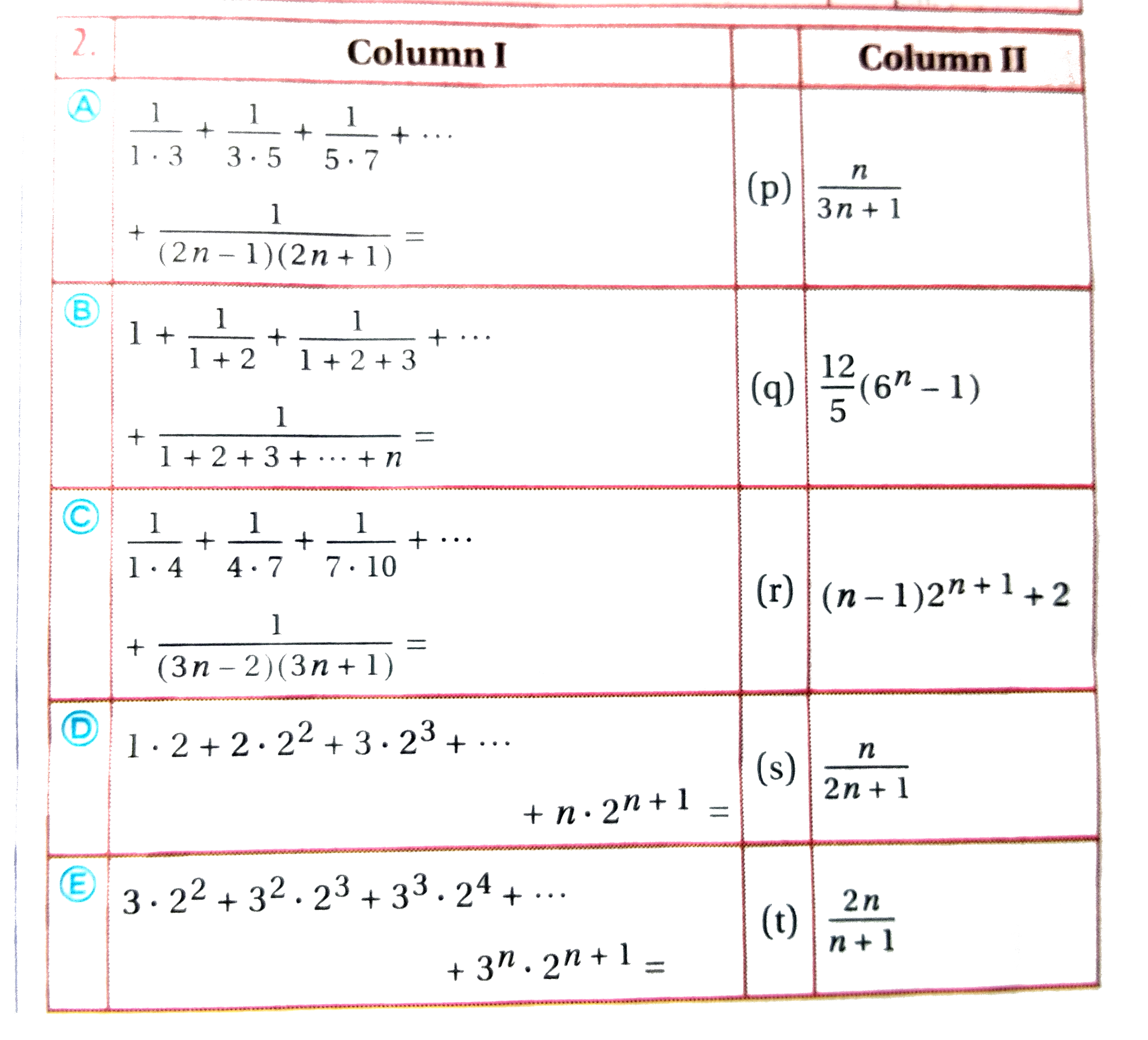
View Solution`P(n):11^(n+2)+1^(2n+1)` where n is a positive integer
p(n) is divisible by -
View Solution`P(n):11^(n+2)+1^(2n+1)` where n is a positive integer
If P(n) = 14642, then the value of n is -
View Solution`P(n):11^(n+2)+1^(2n+1)` where n is a positive integer
If `n=3`, then the last digit of expression is -
View SolutionIf n be a positive integer and `P(n):4^(5n)-5^(4n)`
P(n) is divisible by -
View SolutionIf n be a positive integer and `P(n):4^(5n)-5^(4n)`
If P(n) be negative value, then the value of n is -
View SolutionIf n be a positive integer and `P(n):4^(5n)-5^(4n)`
When n=3 then the last digit of the expression will be -
View Solution`underset(nrarroo)lim[(1)/(sqrtn)+(1)/(sqrt(2n))+(1)/(sqrt(3n))+...+(1)/(n)]`
View SolutionStatement - I : For every natural number `((n+4)!)/((n+1)!)` is divisible by 6.
Statement - II : Product of three consective natural numbers is divisible by 3 !
View Solution
Sample Questions for Competitive Exams questions and complete solutions for chapter MATHEMATICAL INDUCTION of CHHAYA MATHS (BENGALI ENGLISH) of Class 11