Complete Solutions to EXAMPLE of chapter COMBINATORICS AND MATHEMATICAL INDUCTION of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | COMBINATORICS AND MATHEMATICAL INDUCTION |
| FULL MARKS | EXAMPLE |
| Number Of Questions | 68 |
| Academic Year | 2023-24 |
Suppose one girl or one boy has to be selected for a competition from a calss comprising 17 boys and 29 girls. In how many different ways can this selection be made?
View SolutionConsider the 3 cities chennai, Trichy and Tirunelveli. In order to reach Tirunelveli from chennai, one has to pass through Trichy . There are 2 roads connecting chennai with Trichy and there are 3 roads conecting Trichy with Tirunelveli. What are the total number of ways of going chennai to Tirunelveli?
View SolutionA school library has 75 books on Mathematics, 35 books on physics. A student can choose only one book , In how many ways a student can choose a book on Mathematics or physics?
View SolutionIf an electricity consumer has the consumer number say 238:110:29, then describe the linking and count of house connections upto the 29th consumer "connection" linked to the larger capacity transformer number 238 subject to the condtition that each smaller capacity transformer can have a maximal consumer link of say 100.
View SolutionA person wants to buy a car. There are two brands of car available in the market and each brand has 3 veriant models and each model comes in five different colours. In how many ways she can choose a car to buy?
View SolutionA Women wants to select one silk saree and one sungudi saree from a textile shop located at kancheepuram. In that shop, there are 20 different varieties of silk saree and 8 different varieties of sungudi saree, In how many ways she can select her serees?
View SolutionIn a village , out of the total number of people , 80 percentage of the people own coconut groves and 65 percent of the people own paddy fields. What is the minimum percentage of people own both?
View Solution(i) Find the number of strings of length , which can be firmed using the letters of the word BIRD , without repetition of the letters.
(ii) How many strings of length 5 cm be formed out of the letters of the word "PRIME" taking all the letters at a time without repetition.
View SolutionHow many strings of length 6 can be forms using letters of the word FLOWER if (i) either starts with F or ends with R? (ii) neither starts with F nor ends with R?
View SolutionHow many licence plates may be made using either two distinct letters followed by four digits or two digits followed by 4 distinct letters where all digits and letters are distinct?
View SolutionCount the number of positive integers greater than 7000 and less than 8000 which are divisible by 5, provided that no digits are repeated.
View SolutionHow many 4 - digit even numbers can be forms using the digits 0,1,2,3 and 4,if repetition of digits are not permitted?
View SolutionFind the total number of outcomes when 5 coins are tossed once.
View SolutionIn how many ways (i) 5 diffrents balls be distributed among 3 boxes?(ii) 3 different balls be distributed among 5 boxes?
View SolutionThere are 10 bulbs in a room . Each one of them can be operated independently. Find the number of ways in which the room can be illuminated.
View SolutionFind the value of (i) 5! (ii) 6!-5! (iii) `(8!)/(5!xx2!)`
View SolutionSimplify `(7!)/(2!)`
View SolutionEvaluate `(n!)/(r!(n-r)!)` when (i) n=7,r=5 (ii) n=50, r=47 (iii) For any n with r = 3
View SolutionLet N denote the number of days. If the value of N! is equal to the total number of hours in N days then find the value of N?
View SolutionIf `(6!)/(n!)=6`,then find the value of n.
View SolutionIf n! +(n-1) ! =3, then find the value of n.
View SolutionWhat is the unit digit of the sum 2! + 3! + 4! + ….22!?
View SolutionIf ` 1/(7!) + 1/(8!) = A/(9!)` then the value of A is
View SolutionShow that ` ((2n)!)/(n!) = 2^(n) { 1,3,5 ,…( 2n -1) }`
View SolutionEvaluate: (i) `""^(4)P_(4)` (ii) `""^(5)P_(2)` (iii) `""^(8)P_(4)` (iv)`""^(6)P_(4)`
View SolutionIf `""^((n+2))P_(4)=42xx^(n)P_(2),` find n.
View SolutionIf `""^(10)P_(r)=""^(7)P_(r+2)` find r.
View SolutionHow many 'letter strings' together can be formed with the letters of the "VOWELS" so that
(i) the strings begin with E
(ii) the strings begin with E and end with W.
View SolutionA number of four different digits is formed with the use of the digits 1,2,3,4 and 5 in all possible ways. Find the following
(i) How many such numbers can be formed?
(ii) How many of these are even?
(iii) How many of these are exactly divisible by 4?
View SolutionHow many different strings can be formed together using the letters of the word "EQUATION" so that
(i) the vowels always come together?
(ii) the vowels never come together?
View SolutionThere are 15 candidates for an examination. 7 candidates are appearing for mathematics examination while the remaining 8 are appearing for different subjects . In how many ways can they be seated in a row so that no two mathematics candidates are together ?
View SolutionIn how many ways 5 boys and 4 girls can be seated in a row so that no two girls are together.
View Solution4 boys and 4 girls from a line with the boys and girls alternating. Find the number of ways of marking this line.
View SolutionA van has 8 seats. It has two seats in the froot with two row of three seats behind. The van belongs to a family, consisting of seven members, `F,M,S_(1),S_(2),S_(3),D_(1),D_(2).` How many ways can the family sit in the van if
i. Ther are no restriction?
ii. Either F or M drives the van ?
iii. `D_(1),D_(2)` sits next to a window and is driving ?
View SolutionIf the letter of the word TABLE are permuted in all possible ways and the word thus formed are arranged in the dictionary order (alphabetical order) , find the ranks of the word TABLE
View SolutionFind the number of ways of arrangeung the letters of the word BANANA.
View SolutionFind the number of ways of arranging the letters of the word RAMANUJAN so that the relative positions of vowels and consonants are not changed.
View SolutionThree twins pose for a photograph standing in a line. How many arrangement are there (i) when there are no restrictions. (ii) when each person is standing next to his or her twin?
View SolutionHow many numbers can be formed using the digits 1,2,3,4,2,1 such that even digits occupies even place?
View SolutionHow many paths are there from start to end on a ` 6xx4 ` grid as shown in the picture?
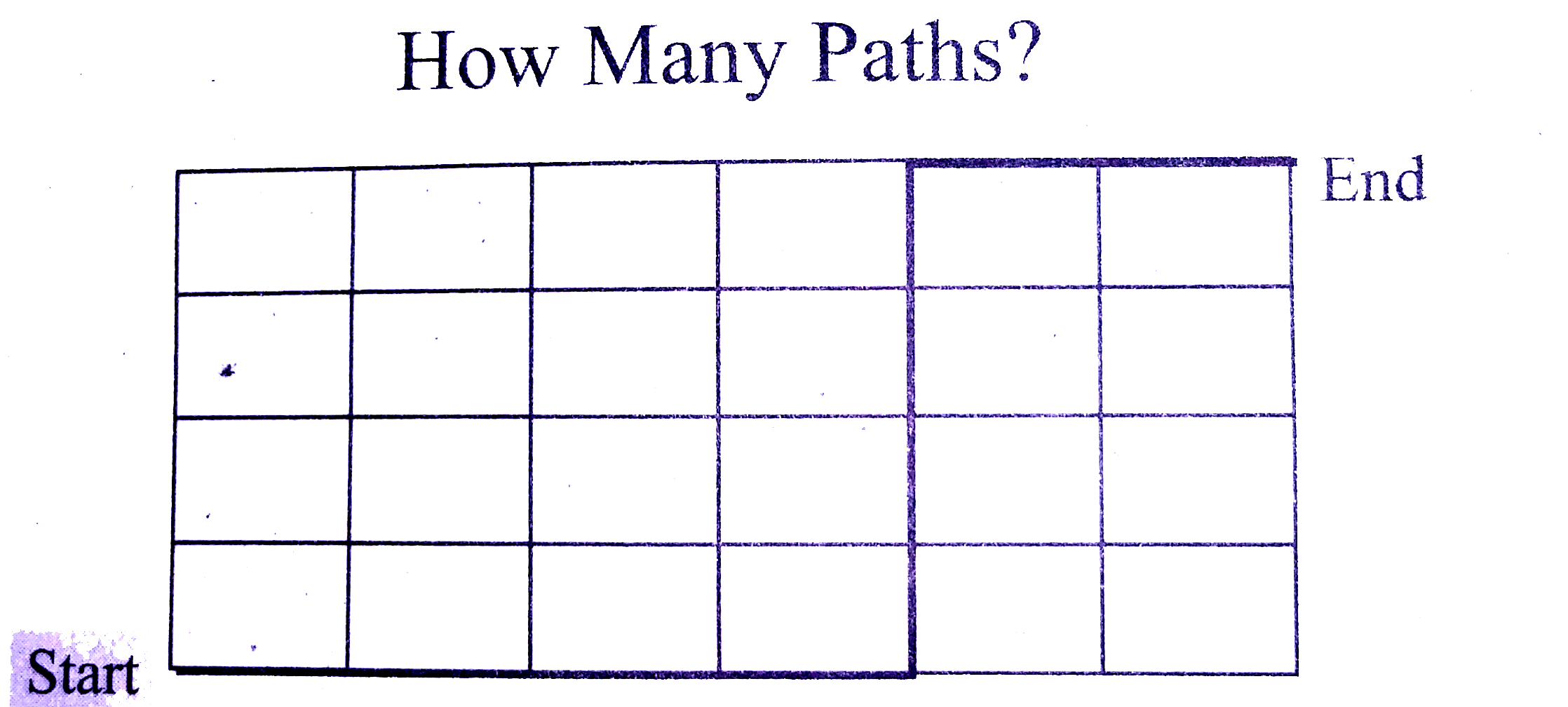
View SolutionIf the different permutations of all letters of the word BHASKARA are listed as in a dictionary, how many strings are there in the list before the first word starting with B?
View SolutionIf the letters of the word IITJEE are permuted in all possible ways and the strings thus are arranged in the dictionary order find the rank of the word IITJEE.
View SolutionEvaluate the following (i) `""^(10)C_(3)` (ii) `""^(15)C_(13)` (iii) `""^(100)C_(99)` (iv) `""^(50)C_(50)`
View SolutionFind the value of `""^(5)C_(2)` and `""^(7)C_(3)` using the property 5
View SolutionIf `nC_(4)=495`, what is n?
View SolutionIf `nP_(r) = 11880 and nC_(r) = 495 ` find n and r .
View SolutionProve that `""^(24)C_(4)+overset(4)underset(r=0)sum^((28-r))C_(3)=^(29)C_(4)`
View SolutionProve that `""^(10)C_(2)+2xx^(10)C_(3)+^(10)C_(4)=^(12)C_(4)`
View SolutionIf `""^((n+2))C_(7):^((n-1))P_(4)=13:24` find n.
View SolutionA salad at a certain restaurant consists of 4 of the following fruits: apple, banana, guava, pomegranate,grapes,papaya and pineapple. Find the total possible number of fruit salads.
View SolutionA Mathematics club has 15 members. In that 8 are girls. 6 of the members are to be selected for a competition and half of them should be girls. How many ways of these selections are possible?
View SolutionIn rating 20 brands of cars, a car magazine picks a first,second,third,fourth and fifth best brand and then 7 more as acceptable, In how many ways can it be done?
View SolutionFrom a class of 25 students, 10 students are to be chosen for an excursion party , There are 4 students who decide that either all of them will join or none of them will join . In how many ways can the excursion party be chosen?
View SolutionA box of one dozen apple contains a rotten apple. If we are choosing 3 apples simultaneously, in how many ways , one can get only good apples.
View SolutionAre exam paper contains 8 questions, 4 in Part A and 4 in Part B. Examiners are required to answer 5 questions. In how many ways can this be done if (i) There are no restrictions of choosing a number of questions in either parts.(ii) At least two questions from Part A must be answered
View SolutionOut of 6 consonants and 4 vowels, how many strings of 3 consonants and 2 vowels can be formed ?
View SolutionIf a set of m paralled lines intersect another set of n parallel lines (not parallelto the
lines in the first set), then find the number of parallelograms fromed in this lattice structure.
View SolutionWhat is the formula to find how many diagonals are there in a polygon with n sides?
View SolutionBy the principle of mathematical induction , prove that , for all integers n`ge`1,
1+2+3+…+`n=(n(n+1))/(2)`
View SolutionProve that the sum of first n positive odd numbers is `n^(2)`
View SolutionUsing the principle of Mathematical Induction , `forall n in N`, prove that `1^2+2^2+3^2+.....n^2=(n(n+1)(2n+1))/6`
View SolutionProve by mathematical induction `1/1.2 + 1/2.3 + . . .+ 1/((n)(n+1)) =n/((n+1))`
View SolutionProve that `x^n-y^n` is divisible by x - y for all positive integers n.
View SolutionProve by mathematical induction that for every natural number n, `3^(2n+2)-8n-9` is divisible by 8 for all n `ge`1.
View SolutionUse the Principle of Mathematical Induction to verify that, for n any positive integer, `6 ^n −1` is divisible by `5`.
View SolutionEvaluate : `((n + 2)! )/ (n!)`
View SolutionBy the principle of mathematical induction , prove that , for `n in NN`
`cos alpha + cos (alpha + beta)+cos (alpha + 2 beta) + .....+ cos (alpha +(n-1)beta) = cos (alpha + ((n-1)beta)/(2)) xx (sin((nbeta)/(2)))/(sin(beta/2))`
View SolutionUsing the mathematical induction, show that for any natural number n, with the assumption `i^(2)=-1`,`(r(costheta+isintheta))^(n)=r^(n)(cosntheta+isinntheta)`
View Solution
EXAMPLE questions and complete solutions for chapter COMBINATORICS AND MATHEMATICAL INDUCTION of FULL MARKS MATHS (TAMIL ENGLISH) of Class 11