Complete Solutions to EXAMPLE of chapter WAVE MOTION AND WAVES ON A STRING of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WAVE MOTION AND WAVES ON A STRING |
| HC VERMA | EXAMPLE |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
A wave is propagating on a long stretched string along its length taken as the positive x-axis. The wave equation is given as
`y=y_0e^(-(t/T-x/lamda)^(2))`
where `y_0=4mm, T=1.0s and lamda=4cm`.(a) find the velocity of wave. (b) find the function `f(t)` giving the displacement of particle at x=0. (c) find the function g(x) giving the shape of the string at t=0.(d) plot the shape g(x) of the string at t=0 (e) Plot of the shape of the string at t=5s.
View SolutionConsider the wave `y=(5mm)sin[(1cm^-1)x-(60s^-1)t]`. Find the amplitude, b. the wave number, c the wavelength d. the frequency e. the time period and f. the wave velocity.
View SolutionFigure showa a string of linear mass density `1.0gcm^-1` on which a wave pulse is travelling. Find the time taken by the pulse in travelling through a distance of 50 cm on the string. Take `g=10 ms^-2`.
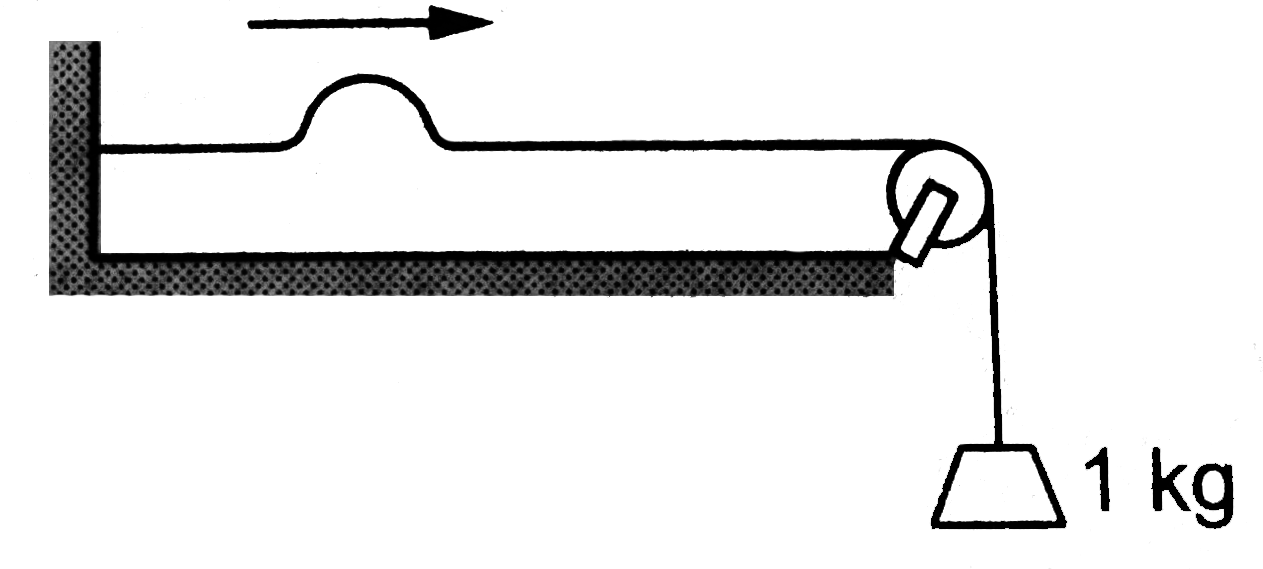
View SolutionThe average power transmitted through a given point on a string supporting a sine wave is 0.20 W when the amplitude of the wave is 2.0 mm. What power will be transmitted through this point if the amplitude is increased to 3.0 mm.
View SolutionTwo waves are smultaneously passing through a string. The equation of the waves are given by
`y_1=A_1sink(x-vt)`
and `y_2=A_2sink(x=vt+x_0)`
where the wave number `k=6.28 cm^-1 and x_0=o1.50c. The ampitudes of `A_1=5.0mm and A_2=4.0mm. find the phase difference between the waves and the amplitude of the resulting wave.
View SolutionTwo travalling wavews of equal amplitudes and equal frequencies move in opposite directions along a string. They interfere to produce a standing wave having the equation `y = A cos kx sin omega t` in which `A = 1.0 mm, k = 1.57 cm^(-1) and omega = 78.5 s^(-1)` (a) Find the velocity of the component travelling waves. (b) Find the node closet to the origin in the x gt 0. (c ) Find the antinode closet to the origin in the region x gt 0 (d) Find the amplitude of the particle at x = 2.33cm.
View SolutionA 50 cm long wire of mass 20 g suports a mass of `1.6 kg ` as shown in figure. Find the fundamental frequency of the portioin of the strig between the wall and the pulley. Take `g=10 ms^-2`.

View SolutionIn a sanometer experiment, resonance is obtained whth the experimental wire has a length of 21 cm between the bridges and the vibrations are excited by a tunning fork of frequency 256 Hz. If a tuning fork of frequency6 384 Hz is used, what should be the lenght of the experimental wire to get the resonance.
View Solution
EXAMPLE questions and complete solutions for chapter WAVE MOTION AND WAVES ON A STRING of HC VERMA of Class 11