Complete Solutions to Exercises of chapter LAWS OF THERMODYNAMICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LAWS OF THERMODYNAMICS |
| HC VERMA | Exercises |
| Number Of Questions | 22 |
| Academic Year | 2023-24 |
A thermally insulaated, closed copper vessel contains water at `15^(0)C`. When the vessel is shaken vigorously for 15 minutes, the tempreture rises to `17^(0)C`. The mass of the vessel is 100 g and that of the water is 200 g. the specific heat capacities of copper and water are `420 Jkg^(-1) K^(-1)` and `4200 J kg^(-1)K^(-1)` respectively. negalect any thermal expansion. (a) how much heat is transferred to the liquid-vessel system ? (b)how much work has been done on this system? (c ) how much is the increase in internal energy if the system ?
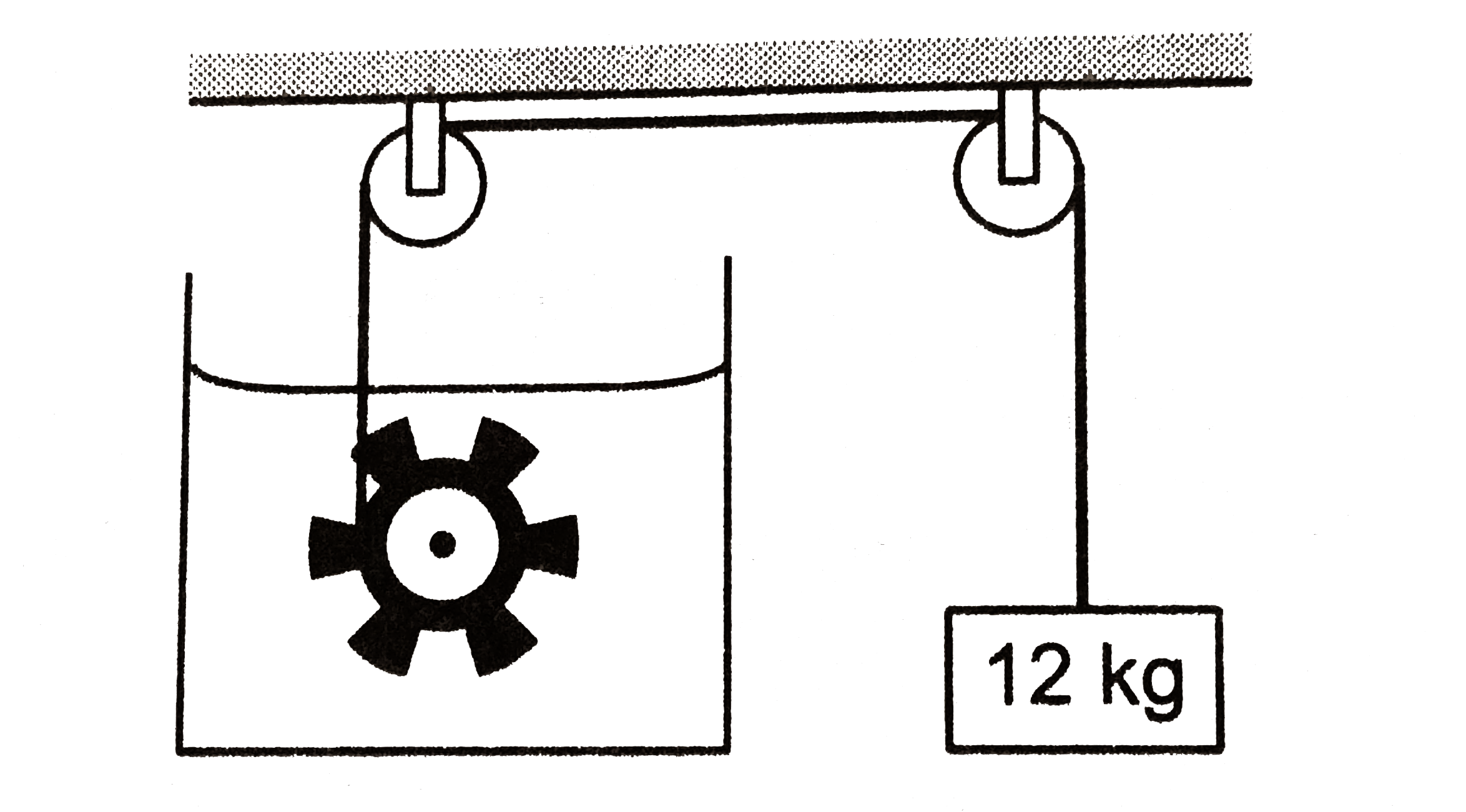
View SolutionShows a peddle wheel couple to a mass of 12 kg through fixed frictionless pulleus. The paddle is immersed in a liquid of heat capacity `4200 J K^(-1)` kept in an adiabatic container. Consider a time interval in which the 12 kg block falls slowly through 70cm. (a) how much heat is given to the liquid ? (b) how much work is done on the liquid? (c ) calculate the rise in the temperature of the liquid neglecting the heat capacity of the container and the paddle.
View SolutionA 100 kg block is started with a speed of `2.0 m s^(-1)` on a long, rough belt kept fixed in a horizontal position. The coefficient of kinetic friction between the block and the belt is 0.20. (a) calculate the change in the internal energy of the block=-belt system as the block comes to a stop on the belt. (b) consider the sutuation from a frame of reference moving at `2.0 m s^(-1)` along the initial velocity of the block. as seen from this frame, the block is gently put on a moving belt and in due time the block starts moving with the belt at `2.0 m s^(-1)`, calculate the increase in the kinetic energy of the block as it stops slipping past the belt. (c ) find the work done in this frame by the external force holding the belt.
View Solutioncalculate the change in internal energy of a gas kept in a rigid container when 100 J of heat is supplied to it.
View Solutionthe pressure of a gas change linearly with volume from 10kPa, 200 cc to 50 kPa, 50 cc. (a) calculate the work done by the gas, (b) what is the change in the internal energy of the gas ?
View SolutionAn ideal gas is taken from an inirial state I to a final state f in such a way that the ratio of the pressure to the absolute temperature remains constant. What will be the work done by the gas ?
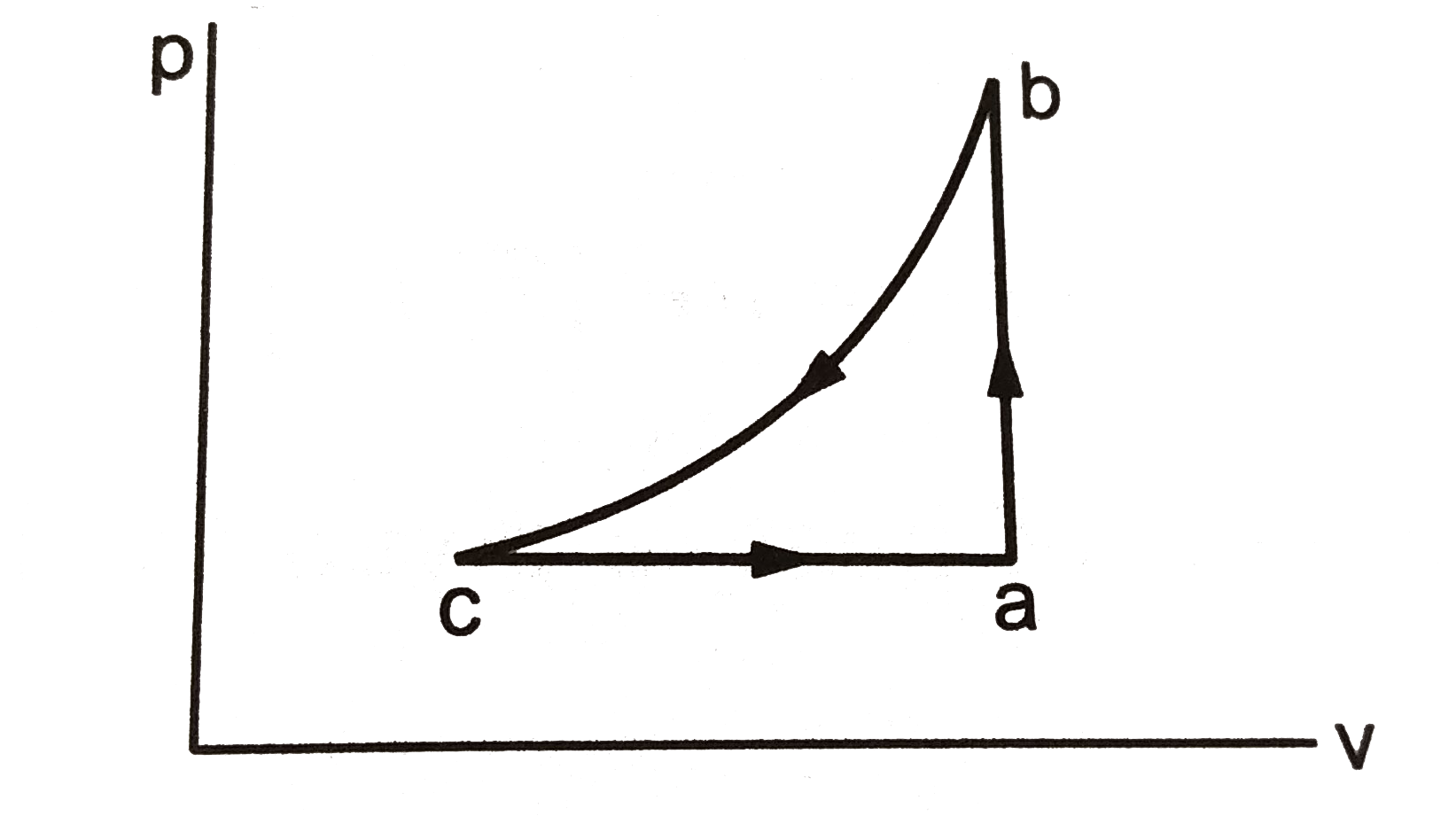
View Solutionshows three paths through which a gas can be taken from the state A to the state B. calculate the work done by the gas in each of the three paths.
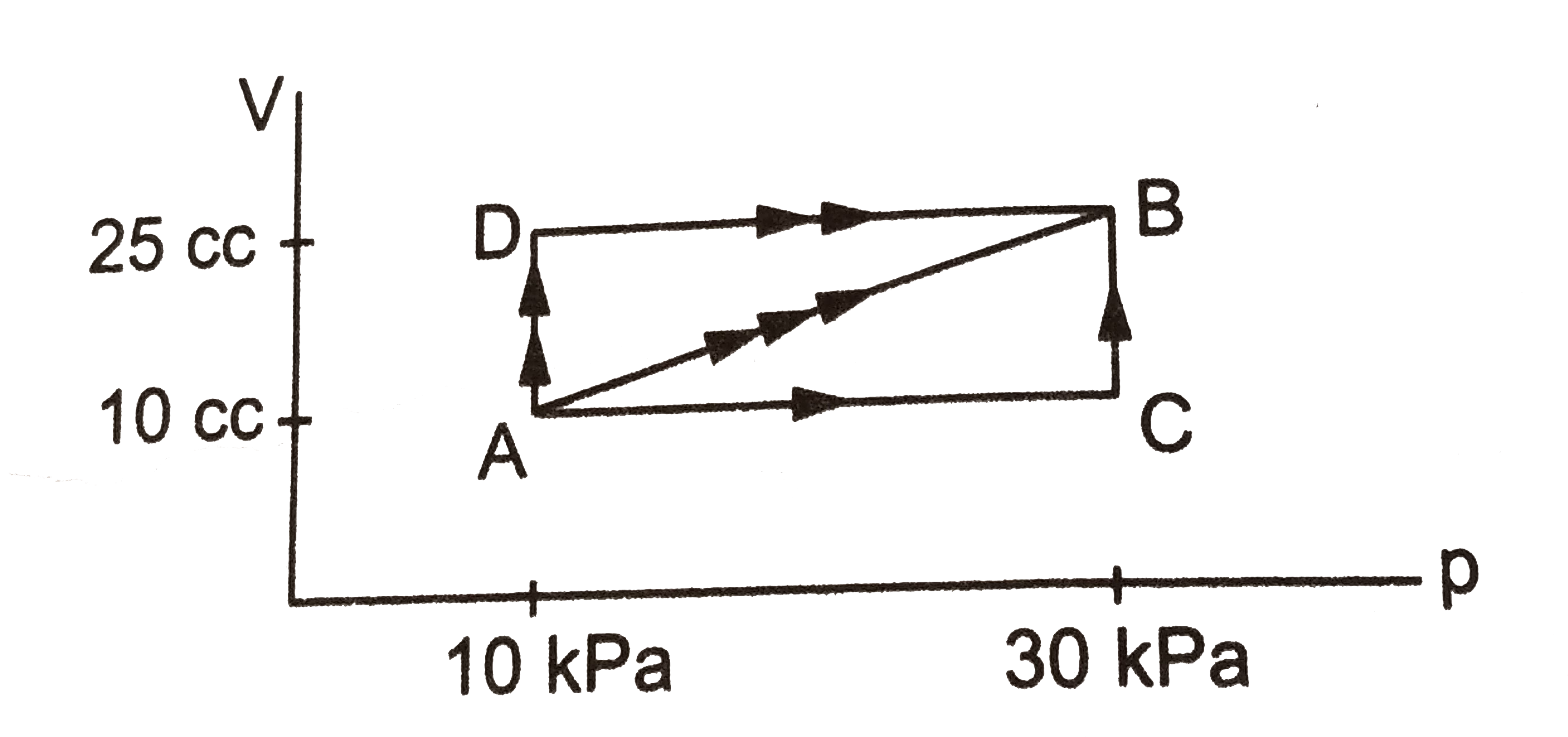
View Solutionwhen a system is taken through the process abc shown in 80 J of heat is absorbed by the system and 30 J of work is done by it. If the system does 10 J work during the process adc, how much heat flows into it during the process ?
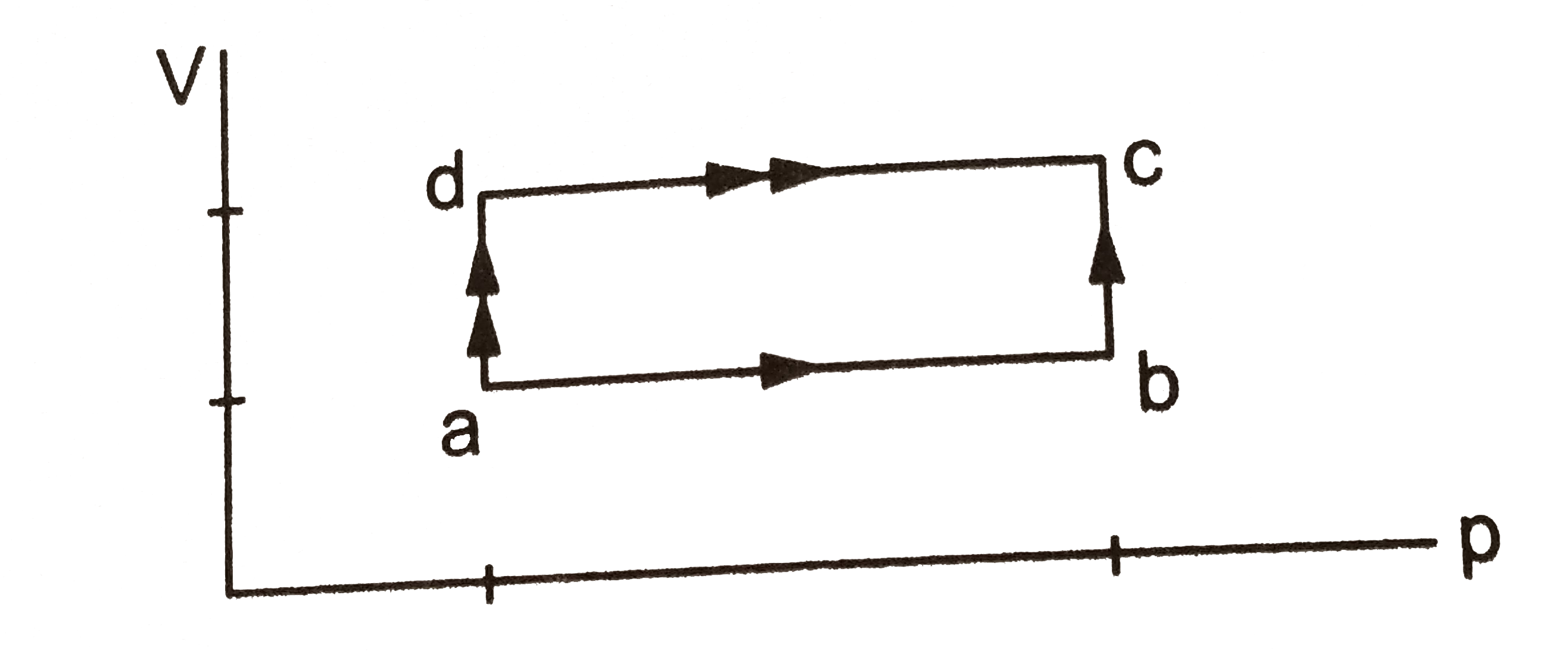
View Solution50 eal of heat should be supplied to take a system from the state A to the state B through the path ABC as shown in ,find the quantity of heat to be supplied to take it from A to B via ADB.
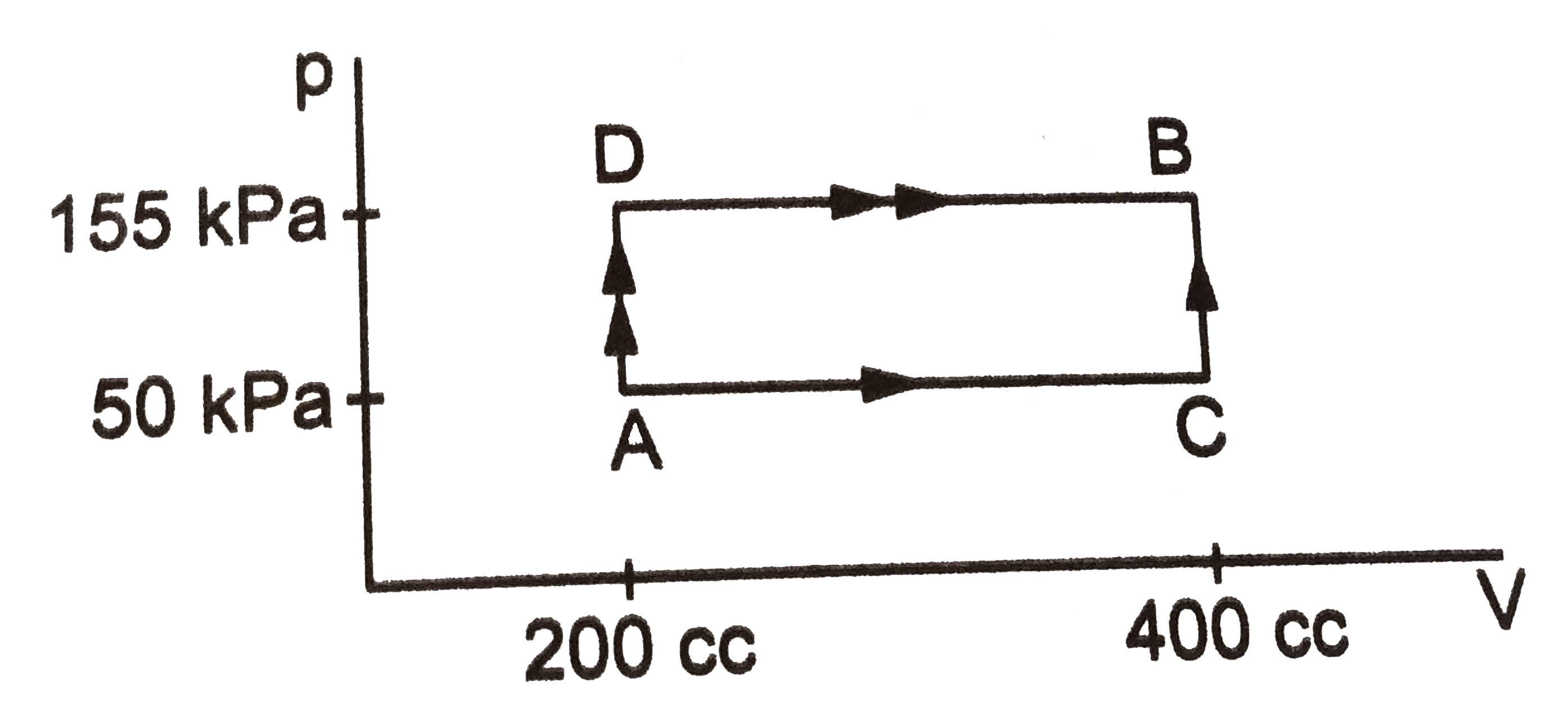
View Solutioncalculate the heat absorbed by a system in going through the cyclic process shown in ,
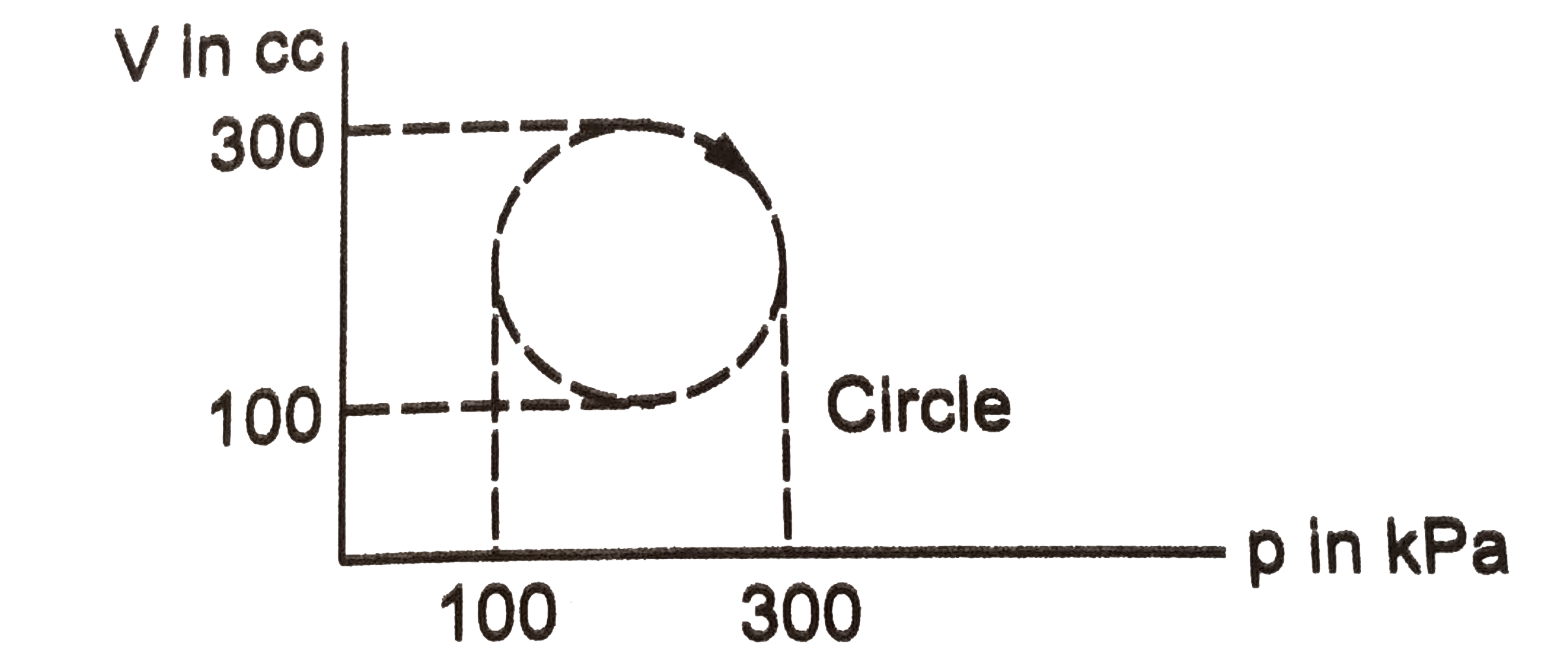
View SolutionA gas is taken through a cyclic process ABCA as shown in, if 2.4 cal of heat is given in the process, what is the value of J ?
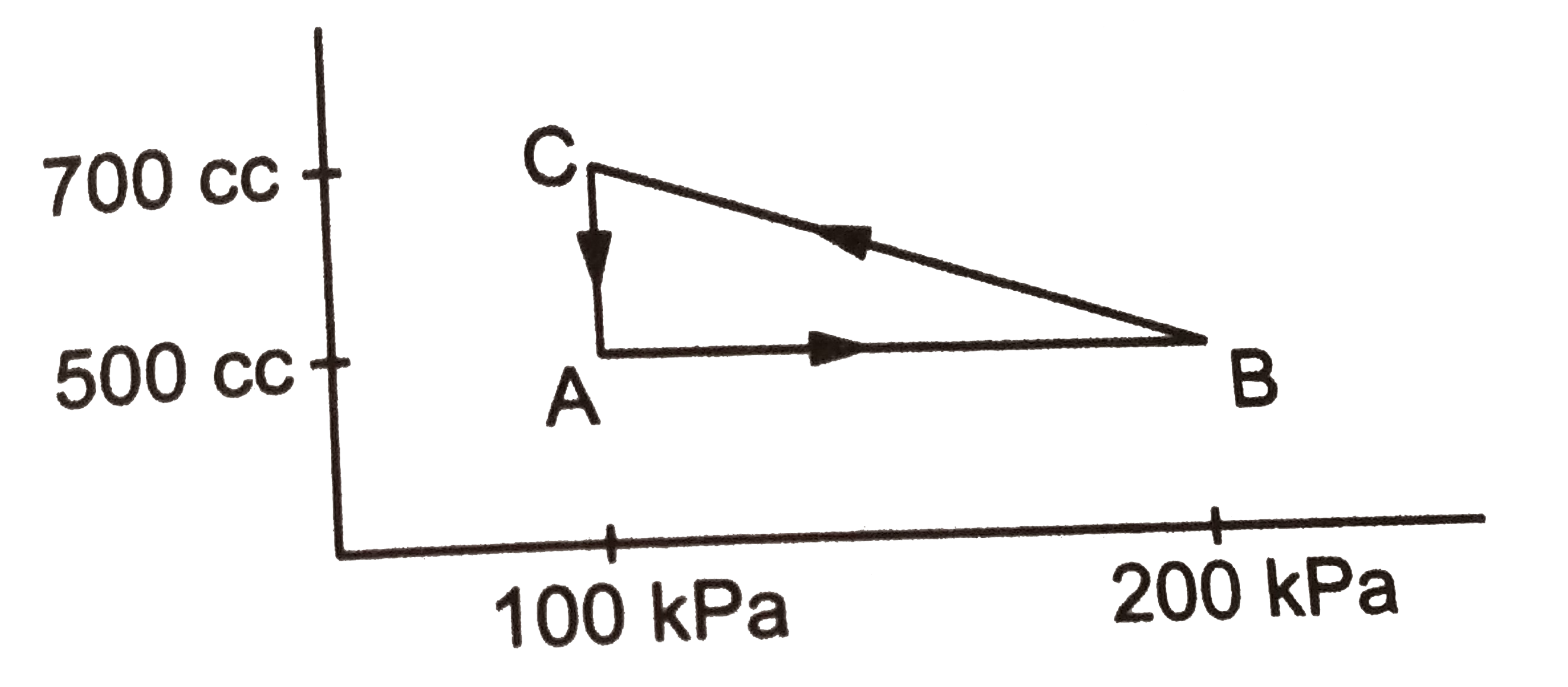
View SolutionA substance is taken through the process abc as shown in, if the internal energy of the substance increase by 5000 J and a heat of 2625 cal is given to the system, calculate the value of J.

View SolutionA gas is taken along the path AB as shown in , if 70 cal of heat is extracted from the gas in the process, calculate the change in the internal energy of the system.
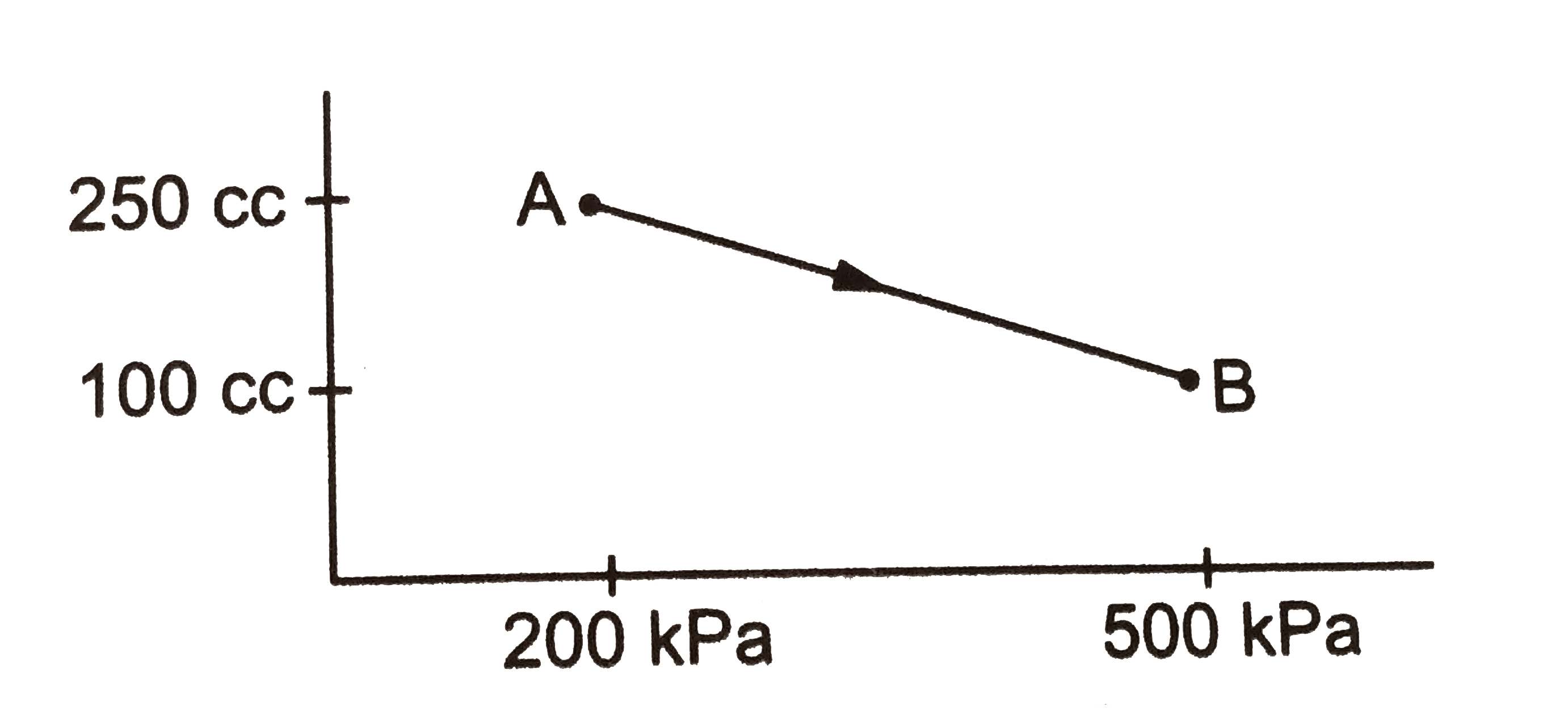
View SolutionThe internal energy of a gas is given by `U=1.5pV`. It expands from `100cm^2` to `200cm^3` against a constant pressure of `1.0xx10^5Pa`. Calculate the heat absorbed by the gas in the process.
View SolutionA gas is enclosed in a cylindrical vessel fitted with a frictionless piston.the gas is slowly heated for some time. During the process, 10 J of heat is suopplied and the piston is found to move out 10cm. Find the increase in the internal energy of the gas , the area of cross section of the gas, the area of cross section of the cylinder=4 cm^(4) and the atmospheric pressure `=100 kPa.`
View SolutionA gas is initinaly at a pressure of 100 kPa and its volume is `2.0 m^(3)`. Its pressure is kept constant and the volume is changed from `2.0 m^(3) to 2.5^(3)`. Its volume is now kept constant and the pressure is increased from 100 lPa to 200 kPa. The gas is brought back to its initial state, the pressure varying linearly with its volume. (a) whether the heat is supplied to or exterted from the gas in the complete cycle ? (b) how much heat was supplied or extracted ?
View SolutionConsider the cyclic process ABCA, shown in, performed on a sample of 2.0 mol if an ideal gas. A total of 1200 J if heat is withdrawn from the sample in the process. Find the work done by the gas during the part BC.
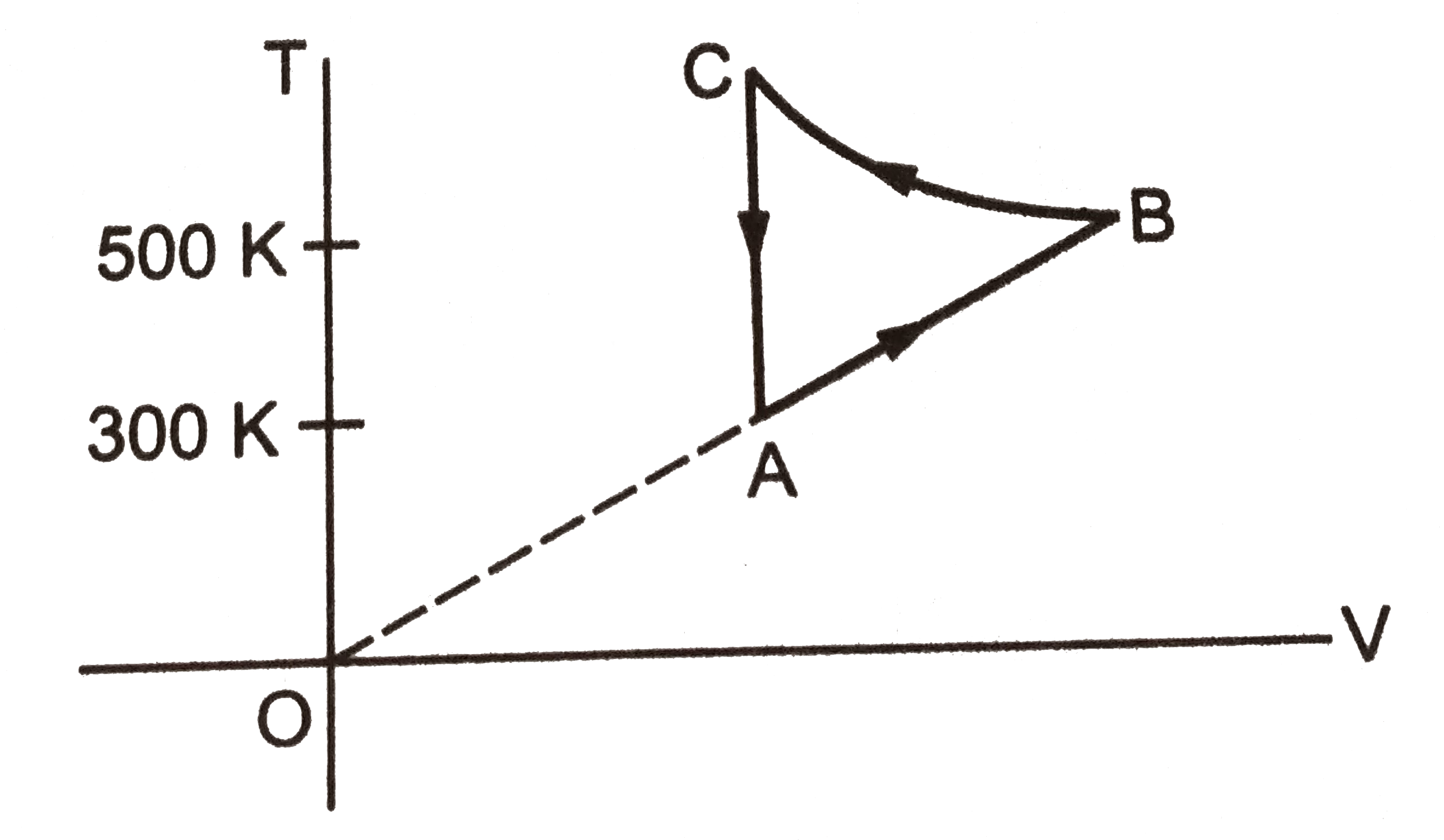
View Solutionshows the variation in the internal energy U with the volume V of 2.0 mol of an ideal gas in a cyclic process abcda. The temperatures of the gas at b and c are 500 K and 300 K respectively. Calculate the heat absorbed by the gas during the process.
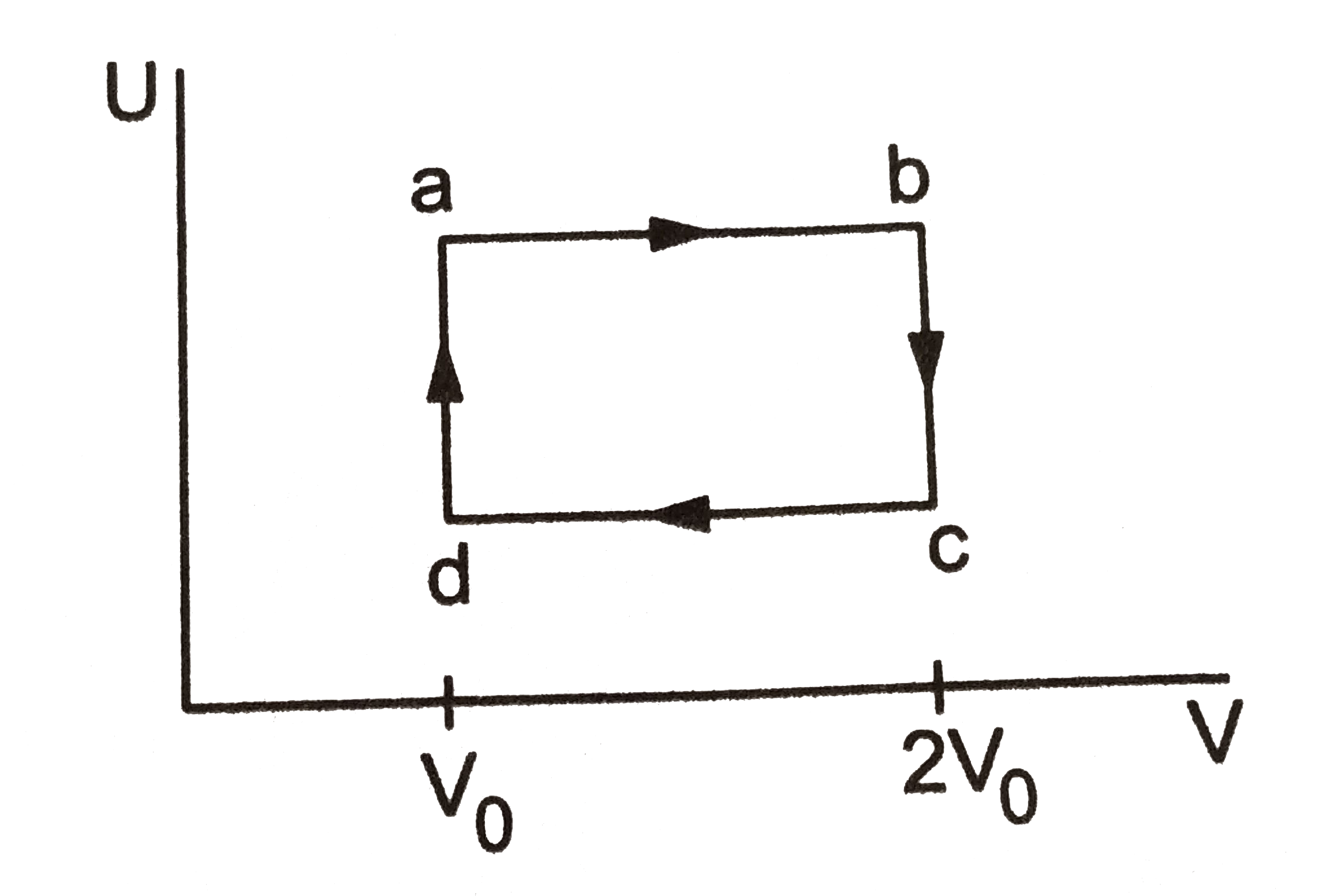
View SolutionFind the change in the internal energy of 2 kg of water as it heated from `0^(0)C to 4^(0)C`. The specific heat capacity of water is `4200 J kg^(-1) K^(-1)` and its densities at `0^(0)C` and `4^(0)C` are `999.9 kg m^(-3)` and `1000 kg m^(-3)` respectively. atmospheric pressure `=10^(5)` Pa.
View SolutionCalculate the increase in the internal energy of 10 g of water when it is heated from `0^(0)C to 100^(0)C` and converted into steam at 100 kPa. The density of steam `=0.6 kg m^(-3)` specific heat capacity of water `=4200 J kg^(-1 ^(0)C^(-3)` latent heat of vaporization of water `=2.25xx10 6 J kg^(-1)`
View Solutionshows a cylindrial tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the tempreture are `p_(1), T_(1)` on the left and `p_(2), T_(2)` on the right. the system is left for sufficient time so that the tempreture becomes equal on the two sides. (a) how much work has been done by the gas on the left part ? (b) find the final pressures on the two sides. (c ) find the final equilibrium tempreture. (d) how much heat has flown from the gas on the right to the gas on the left ?
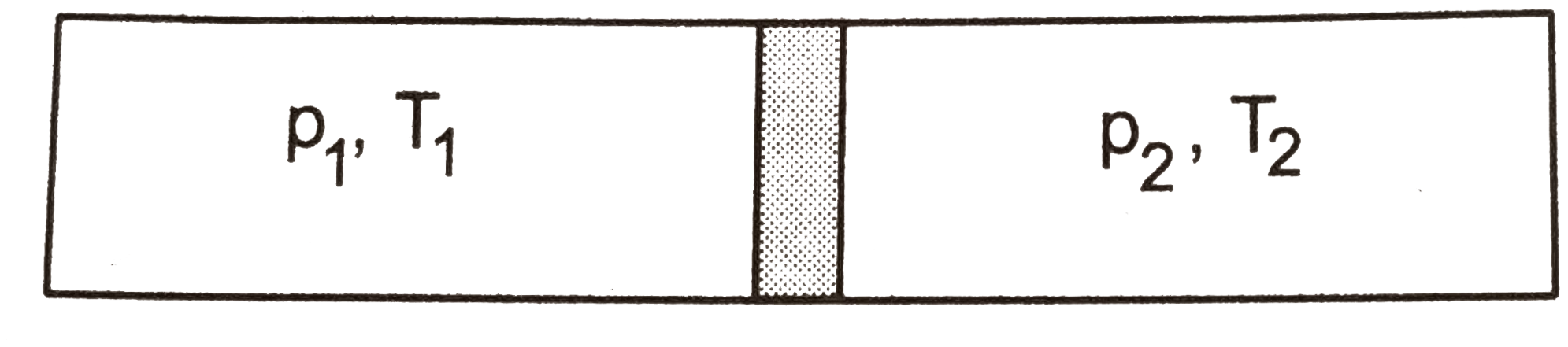
View SolutionAn adiabatic vessel of total volume V is divided into two equal parts by a conducting separtor. The is fixed in this position. The part on the left contains one mole of an ideal gas `(U=1.5 nRT)` and the part on the right contains two moles of the same gas. initially, the pressure on each side is p. the system is left for sufficiant time so that a steady state is reached. find (a) the work done by the gas in the left part during the process, (b) the temperature on the two sides in the beginning, (c ) the final common temperature reached by the gases, (d) the heat given to the gas in the right part and (e) the increase in the internal energy of the gas in the left part.
View Solution
Exercises questions and complete solutions for chapter LAWS OF THERMODYNAMICS of HC VERMA PHYSICS (HINGLISH) of Class 11