Complete Solutions to SECTION A of chapter SAMPLE QUESTION PAPER 01 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | SAMPLE QUESTION PAPER 01 |
| OSWAL | SECTION A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
The domain of the function f(x)= `sqrt(9-x^(2))` is :
View SolutionSolve `: tan ((pi)/(4)+ theta) + tan ((pi)/(4) - theta) = 4`
View SolutionIf `theta=4530^(@)`, then `"sin" 4530^(@)` is :
View SolutionThe third term of a G.P. is 2. Then product of the first five terms, is :
View SolutionIf `alpha and beta` are roots of the equation `x^(2)-2x+1=0`, then the value of `(alpha)/(beta)+(beta)/(alpha)` is
View SolutionIf the coefficients of `x^2 and x^3` in the expansion of `(3 + ax)^(9)` be same, then the value of `a` is
View SolutionIf `1 , omega, omega^(2)` are cube roots of unity then the value of `(3 + 5omega+3omega^(2))^(3)` is
View SolutionThe point diametrically opposite to the point (-3, -4) on the circle `x^(2)+y^(2)+2x+4y-3=0` is :
View SolutionA straight line that has equal intercepts on axes but opposite in magnitude and passing through the points (3, 2) is :
View Solution`lim_(xto(pi))(sinx)/(x-pi)` is equal to
View SolutionFind the numbers x and y if `(x+3, y-6)=(5, 5)`.
View SolutionHow many 4-digit numbers are there with no digit repeated?
View SolutionFind the middle term in the expansion of `((x)/(y)+(y)/(x))^(10)`.
View SolutionFind the derivative of f(x)= `2x^(2)+4x+5` at x = 2.
View SolutionA family has exactly 4 children. Assuming that each child born is equally likely to be boy or girl,then what is the sample space that the event to heaving exactly one girl child ?
View SolutionIf`A={1, 2, 3, 4, 5, 6} B={2, 4, 5, 6, 8, 9, 10}, "find" ADeltaB`.
View SolutionIn a class of 35 students, 24 like to play cricket and 16 like to play football. Also, each student likes to play at least one of the two games. How many students like to play both cricket and football ?
View SolutionProve that : `("cos" 2A)/(1+"sin" 2A)="tan"(pi/(4)-A)`
View SolutionIn any `DeltaABC`, prove that
`(sinA)/(sin(A+B))=a/c`
View SolutionIf `"tan" alpha=-2`, find the value of `"sin" alpha`(`alpha` lies in the II Quadrant).
View SolutionIf `(2 + 3i)/(3 - 4i) = a + ib`, find the values a and b.
View SolutionIf `alphaandbeta` are roots of the equation `x^(2)+kx+12=0andalpha-beta=1`, find k.
View SolutionFind the domain and range of : `2-|x-4|.`
View SolutionSolve : `"sin" 7x+"sin" 4x+"sin" x=0and 0ltxltpi/(2)`
View SolutionProve that `("cos"A+"cos"3A+"cos"5A+"cos"7A)/("sin"A+"sin"3A+"sin"5A+"sin"7A)="cot"4A`
View SolutionUsing Mathematical induction, prove that `10 ^(n)+3.4^(n+2)+5` is divisible by 9 for all `ninN`.
View SolutionDifferentiate the function `"sin"(2x-3)` by First Principle of differentiation.
View SolutionEvaluate : `lim_(xrarr0)((1-x)^(n)-1)/(x)`
View SolutionIf 'x' be real, find the maximum and minimum value of : `y=(x+2)/(2x^(2)+3x+6)`
View SolutionIf `alpha,beta` be the roots of the equation `x^(2)+lx+m=0`, then from an equation whose roots are : `(alpha+beta)^(2)and(alpha-beta)^(2)`
View SolutionThe sum of three consecutive numbers of a G.P is 56. If we sunbtract 1,7 and 21 from the these numbers in the order the resulting numbers from an A.P. Find the numbers.
View SolutionFind the equation of the circle which passes through the points `(2,3),(4,5)` and the centre lies on the straight line `y-4x+3=0.`
View SolutionFind the equation of acute angled bisector of lines :
`3x-4y+7=0and12x-5y-8=0`
View SolutionFind the mean and standard deviation of the following frequency distribution :
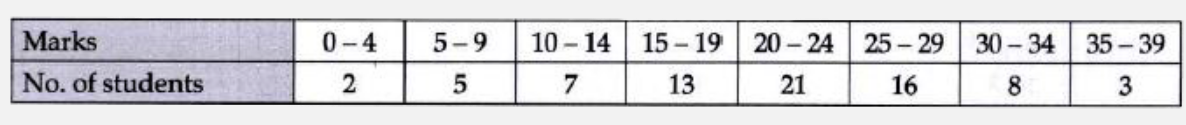
View Solution
SECTION A questions and complete solutions for chapter SAMPLE QUESTION PAPER 01 of S CHAND MATHS (ENGLISH) of Class 11