Complete Solutions to SECTION -A of chapter MODEL TEST PAPER -3 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL TEST PAPER -3 |
| VIVA PUBLICATION | SECTION -A |
| Number Of Questions | 33 |
| Academic Year | 2023-24 |
The value of `2 sin 15^(@).cos75^(@)`
View Solution`i^(57)+1/(i^(125))`, is equal to
View SolutionIf `A={1,3,5,6}`, the number of elements in `P{P(A)}` is
View SolutionThe range of the function `f(x)=(|x-4|)/(x-4)` is
View SolutionThe argument of the complex number `((3+i)/(2-i)+(3-i)/(2+i))` is equal to
View SolutionThe equation of circle having centre at (2,2) and passes through the point (4,5) is
View SolutionThe perpendicular distance from the point (1,-1) to the line `x+5y-9=0` is equal to
View Solution`lim_(ktooo)(1^(3)+2^(3)+……….+k^(3))/(k^(4))` is equal to
View SolutionValue of tan `130^(@). Tan 140^(@)`.
View SolutionWe wish to select 6 persons from 8 persons, but if the person A is chosen, then B must be chosen. Then the number of ways the selection can be made is
View SolutionIf a is the arithmetic mean of b and c, and two geometric means `G_(1)` and `G_(2)` are inserted between b and c such that `G_(1)^(3)+G_(2)^(3)=lamda abc,` then find the value of `lamda`.
View SolutionIf without repetition of the numbers, four digit numbers are formed with the number 0, 2,3, and 5, then find the probability of such number being divisible by 5.
View SolutionDifferentiate w.r.t `x:f(x)=(sinx+sin((pi)/2-x))^(2)`
View SolutionFind the coefficient of one middle term in the expansio of `(1+x)^(2n)`.
View SolutionIn how many ways can the letters of the word ARRANGE be arranged so that the two R's are never together.
View SolutionIf `A={1,2,3,5},B={4,6,9}` and `R={(a,b)| a in A,b in B, a-b` is odd}, then
a. Write R in roster form.
b. Represent R by an arrow diagram.
View SolutionIf the angles of a triangle are in the ratio 3:4:5, find the smallest angle in degrees and the greatest angle in radians.
View SolutionSolve `3 cos 2 theta-sin theta=2`
View SolutionIf `18x=pi`, then prove that `tan 2x.tan3x.tan4x.tan8x=1`
View SolutionFind Re `((z_(1)z_(2))/(z_(1))),` give `z_(1)=2-i` and `z_(2)=-2+i`
View SolutionRepresent to solution set of each of the following inequation graphically in two dimensional plane: `\ -3x+2ylt=6`
View SolutionShow that the function `f:NtoN` defined by `f(x)=2x-1` is one-one but not onto.
View SolutionProve that `cos12^(@)+cos84^(@)+cos132^(@)+cos156^(@)=-1/2`
View SolutionIf in a triangle ABC, `cos A +cos B+cos C=3/2`, prove that the triangle is equilateral.
View SolutionUsing mathematical induction prove that
`1/(n+1)+1/(n+2)+……+1/(2)gt13/24AAn in N` and `n gt1`
View SolutionIf `y=sqrt((1-x)/(1+x))` prove that `(1-x^2)(dy)/(dx)+y=0`
View SolutionGiven `f(x)={((x+|x|)/x,x!=0),(-2,x=0):}` show that `lim_(xto0)f(x)` does not exist.
View SolutionIf `alpha ` and `beta` are the roots of `x^(2)+ax+b=0`, then prove that `(alpha)/(beta)` is a root of the equation `bx^(2)+(2b-a^(2))x+b=0`
View SolutionFind the values of x for which the inequality `(8x^(2)+16x-51)/((2x-3)(x+4))gt3` holds.
View SolutionIf (p+q)th term of G.P is `m, (p-q)` th term is n, show that pth term is `sqrt(mn)` and qth term is `m(n/m)^(p/(2q))` .
View SolutionFind the equation of the line passing through the intersection of the lines `3x-4y+1=0` and `5x+y-1=0` which cuts off equal intercepts on the axes.
View SolutionFind the equation of the circle which has extremities of a diameter the origin and the point (2, - 4). Find also the equations of the tangents to the circle which are parallel to this diameter
View SolutionThere are 60 students in a class. The followng is the frequency distribution of marks obtained by the students in a test.
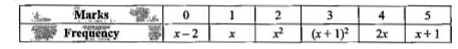
where x is positive integer. Determine the mean of the marks.
View Solution
SECTION -A questions and complete solutions for chapter MODEL TEST PAPER -3 of S CHAND MATHS (ENGLISH) of Class 11