Complete Solutions to SECTION A of chapter SELF ASSESSMENT PAPER 3 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | SELF ASSESSMENT PAPER 3 |
| OSWAL | SECTION A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
If A = {1,2,3,4}, then the number of subsets of A is:
View SolutionThe maximum value of sin x cos x is ……..
View SolutionThe value of `(1-tan^(2)15^(@))/(1+tan^(2)15^(@))` is
View SolutionThe tenth term of the AP: 1,3,5,7, ……. Is ……..
View SolutionIf `alpha and beta` are the roots of the equation `2x^(2) - 3x + 4 = 0`, then `alpha^(2) + beta^(2)` = ____
View SolutionFind the number of terms in the following expansions.
`(x+y)^(100) + (x-y)^(100)`
View SolutionThe value of `i + i^(2) + i^(3) + i^(4)` is ________
View SolutionThe radius of the circle `x^(2) + y^(2) - 2x + 4y - 4 = 0` is ______
View SolutionSlope of the line joining the points (2,3) and (k,5) is 2,then k = _______
View Solution`lim_(xto0) (sinx^(@))/(x)` = _________
View SolutionFind the range of (x) = |sinx|.
View SolutionHow many three digit numbers can be formed using the digits from 1 to 5 ?
View SolutionFind the middle term in the expansion of `(x + 2)^(6)`.
View SolutionFind the derivative of x + sin x with respect to x when x = 0.
View SolutionA = {1,3,5,7}, B = {1,2,3,4), find A `Delta` B.
View SolutionThe probability that atleast one of the events A and B occurs is 0.7. If A and B occurs simultaneously with probability 0.35 then find `P(bar(A)) + P(bar(B))`
View SolutionIf A = `{3n + 5 : n in N and n le 6}`, then represent set A I the roster form.
View SolutionIf tan `A = (a)/(a + 1) and tan B = (1)/(2a + 1)`, then find the value of A + B.
View SolutionEvaluate sin `(pi + x) sin (pi - x) cosec^(2)x`
View SolutionProve that `sin^(2) (pi/6) + cos^(2) (pi/3) - tan^(2) (pi/4) = - (1)/(2)`
View SolutionIf `(2 + 3i)/(3 - 4i) = a + ib`, find the values a and b.
View SolutionGiven the `alpha + beta` are the roots of the quadratic equation `px^(2) + qx + 1 = 0` find the value of `alpha^(3)beta^(2) + alpha^(2)beta^(3)`.
View SolutionIf `f(x) = (1 + x)/(1 - x)` Prove that `((f(x) f(x^(2)))/(1 + [f(x)]^(2))) = (1)/(2)`
View SolutionIf `tan - A tan B = x, cot B - cot A = y` prove that cot (A - B) = `(1)/(x) + (1)/(y)`
View SolutionProve that : `(sec 8theta - 1)/(sec 4theta - 1) = (tan 8theta)/(tan 2theta)`
View SolutionProve by the principle of mathematical induction `4^(n)+ 15n - 1` is divisible 9 for all `n in N`.
View SolutionUsing principle of mathematical induction, prove that
`1 + 3 + 3^(2) + … 3^(n-1) = (3^(n) - 1)/(2)`
View SolutionSolve the following inequality `(x + 8)/(x -2) ge 0`
View SolutionLet `f(x) =(kcosx)/(pi-2x)` if `x!=pi/2` and `f(x)=3` if `x=pi/2`then find the value of `k` if `lim_(x->pi/2) f(x)=f(pi/2)`
View SolutionDifferentiate the function `sqrt(sin x)` by first principal of differentiation.
View SolutionIn an A,P if the pth term is `(1)/(q) and q^(th)` terms is `(1)/(p)`. Prove that the sum of first pq term is `(1)/(2)` (pq+1) where, `(p!=q)`
View SolutionThe sum of three consecutive numbers of a G.P is 56. If we sunbtract 1,7 and 21 from the these numbers in the order the resulting numbers from an A.P. Find the numbers.
View SolutionFind the equation of one of the side of an isosecles right angled triangle whose hypotenuse is given by 3x + 4y = 4 and the opposite vertex of the hypotenuse is (2,2).
View SolutionFind the mean deviation about mean for the following data:
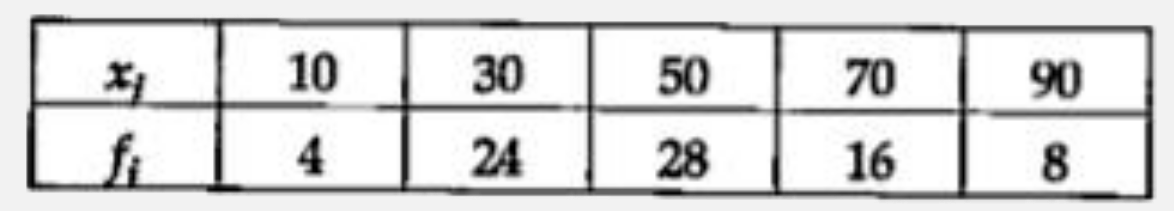
View Solution
SECTION A questions and complete solutions for chapter SELF ASSESSMENT PAPER 3 of S CHAND MATHS (ENGLISH) of Class 11