Complete Solutions to SECTION A of chapter SELF ASSESSMENT PAPER 5 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | SELF ASSESSMENT PAPER 5 |
| OSWAL | SECTION A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
A = {1,2,3), B = {2,3}, then A- B is ………
View SolutionThe maximum value of sin x cos x is ……..
View SolutionThe value of tan `(17 pi)/(4)`
View SolutionWhich term of the sequence 3, 10, 17, …… is 136?
View Solution`alpha and beta` are the roots of the equation `x^(2) - 3x + 5 = 0`, the equation whose roots are `(1)/(alpha) and (1)/(beta)` is:
View SolutionThe number of terms in `(x + y)^(100) (x - y)^(100)` is ______
View SolutionThe value of modulus of `(a + ib)/(a - ib)` is ________
View SolutionThe centre and radius of the circle `x^(2) + y^(2) + 6x + 8y - 11 = 0` is ………….
View SolutionEquation of line passing through (2,3) and parallel to x + 4y - 5 = 0 is ________
View Solution`lim_(x to 0) (1 - cos 4x)/(1 - cos x)` = _________
View SolutionFind the range of f(x) = `(1)/(2 sin x + 5)`.
View SolutionA coin is tossed five times. How many outcomes are possible ?
View SolutionFind the common difference of the A. P, the Sum of whose first n terms is `2n + 5n^(2)`.
View SolutionFind the common difference of the A. P, the Sum of firist n terms is `2n + 5n^(2)`.
View SolutionEvaluate `lim_(x to 2) (x^(5) - 32)/(x - 2)`
View SolutionLet A and B be two events such that, p(A B)=0.8 and p(A)=0.3, find p(B) if A and B are two independent events
View SolutionIf `P={x:(x-8)/(2)=4}`. Is P an empty set? Justify.
View SolutionProve that: (sin 3 x +sin x) sin x + (cos 3x - cos x) cos x = 0
View SolutionProve that : `(cos x - cos y)^(2) + (sin x - sin y)^(2) = 4 sin^(2) ((x - y)/(2))`
View SolutionEvaluate tan `15^(@)`.
View SolutionWrite the amplitude of `(1)/(i)`.
View SolutionIf `alpha and beta` are the roots of the equation `px^(2) + qx + 1`= , find `alpha^(2) beta + beta^(2)alpha`.
View SolutionFind the domain and range of the function f(x) = `(2x + 1)/(x- 2)`.
View SolutionIf A + B = `(pi)/(4)`, then prove that (1 + tan A) (1 + tan B) = 2
View SolutionProve that: `\ sqrt(2+sqrt(2+2cos4theta))=2costheta`
View SolutionUsing mathemtical induction prove that `3^(2n + 2)- 8n - 9` is divisible by 64 for all `n in N`.
View SolutionProve by the principal of mathematcal induction that for all `n in N`.
`1^(2) + 3^(2) + 5^(2) + …… + (2n - 1)^(2) = (n(2n - 1) (2n + 1))/(3)`
View SolutionDetermine the values of m for which the equations `3x^(2)+4mx+2=0 and 2x^(2)+3x-2=0` may have a common root.
View SolutionEvaluate `lim_(xto0)(tan2x-sin2x)/(x^(3))`
View SolutionFind using the first principal of differentiation the derivation of `sin^(2)x`.
View SolutionThe sum of the roots of the quadratic equation `ax^(2) + bx + c = 0` is equal to the sum of the squares of their recipocals, prove that `(c)/(a),(a)/(b),(b)/(c)` are in A.P.
View SolutionIf `a\ a n d\ b` are the roots of `x^2-3x+p=0\ a n d\ c ,\ d` are the roots `x^2-12 x+q=0` where `a , b , c , d` form a G.P. Prove that `(q+p):(q-p)=17 : 15.`
View SolutionWhat are the points on y-axis whose distance from the line `x/3+y/4=1\ i s\ 4\ u n i t s ?`
View SolutionCalculate the mean, variance and standard devation for the following data :
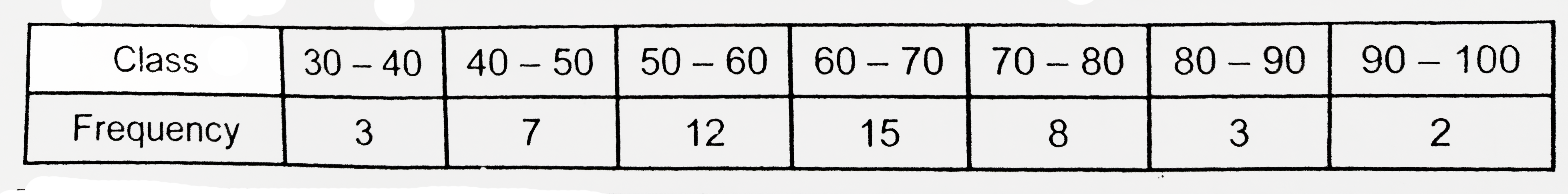
View Solution
SECTION A questions and complete solutions for chapter SELF ASSESSMENT PAPER 5 of S CHAND MATHS (ENGLISH) of Class 11