Complete Solutions to SECTION-A of chapter MODEL TEST PAPER-6 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL TEST PAPER-6 |
| VIVA PUBLICATION | SECTION-A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
The number of subsets of a set containing n elements is :
View SolutionIf f `Q to Q` is defined as `f(x) =x^(2), f^(-1)(9)` is equal to .
View Solution`"cosec" 10^(@) - sqrt(3) " sec" 10^(@)` =
View SolutionIf x is a real number and `|x | lt 5 ` , then
View SolutionThe number of a arrangement of the letter of the word BHARAT taking 3 at a time is
View SolutionIf x is real , then the minimum value of `(x^(2)-3x+4)/(x^(2)+3x+4) ` is :
View SolutionThe tw geometric means between the numbers 1 and 64 are
View SolutionA line passes through the point (2,2) and is perpendicular to the line `3x+ y ` Its y - Intercept is
View SolutionIf the circles `x^(2) +y^(2) = a and x^(2) + y^(2) - 6x - 8y + 9 = 0 ` touch externally then a =
View SolutionIf A,B,C are three mutually exclusive and exhaustive events of an experiment such 3P(A) = 2I = P(C ) , the P(A) is equal to .
View SolutionIf the perimeter of a certain sector of a circle is equal to the length of the arc of the semicircle having the same radius , find the angle of the sector in degrees .
View SolutionThere are 12 points in a plane of which 5 are collinear . Find the number of straight lines obtained by joining these points in pairs .
View SolutionFind the least positive value of n it `((1+i)/(1-i))^(n)=1 `
View SolutionIf f(x) = `alphax^(n)` prove that `alpha = (f'(1))/n`
View SolutionIf f(x) = `alphax^(n)` prove that `alpha = (f'(1))/n`
View SolutionProve that , U = `(U -A) + A, `Where U is the universal set .
View SolutionZ is the set of integers. Describe the following relation in set builder form, given its domain and range.
`{(0,-7),(2,-5),(4,-3),(-13,-20),.......}`
View SolutionThe angles of a triangle ABC are in A.P and b:c = `sqrt(3) : sqrt(2) ` find `angle A`
View SolutionFind the value of `theta, " if " m^(2) sin . Pi/2 - n^(2) sin . (3pi)/2 + 2 mn sec theta = (m-n)^(2) , 0 le theta le pi ` .
View SolutionIn any `DeltaABC`, prove that
`acos(A+B+C)-bcos(B+A)-c""cos(A+C)=0`
View SolutionFind the values of `lamda and mu` if both the roots of the equation `(3lamda+1)x^(2)=(2lamda+3mu)x-3` are infinite.
View SolutionProve the following
`(a + b omega + c omega^(2))/(c + a omega + b omega^(2)) + (a + b omega + c omega^(2))/(b + c omega + a omega^(2)) = -1`
View SolutionDefine modulus function . Write its domain and range . Draw the graph of it .
View SolutionSolve `2 cos^(2) theta = 3 sin theta `
View Solution`tan 6 ^(@) tan 42 ^(@) tan 66 ^(@) tan 78^(@) =1`
View SolutionUsing mathematical induction , to prove that
` 7^(2n)+2^(3n-3). 3^(n-1)` is divisible by 25 , for al `n in N `
View SolutionDifferentiate w.r.t `'x' f(x) = (sqrt(x^(2)+1)+sqrt(x^(2)-1))/(sqrt(x^(2)+1)-sqrt(x^(2)-1))`
View SolutionEvaluate : `lim_(x to oo) sqrt(x^(2)+x +1) - sqrt(x^(2)+1)`
View SolutionFind the equations of the bisectors of the angles between the lines `12x +5y - 4 = 0 and 3x + 4y + 7 = 0 ` .Prove that bisectors are at right angles to each other .
View SolutionFind the equations of the tangents to the circle `x^(2) + y^(2) = 25 ` which are parallel to the line `2x- u + 4 = 0 `
View SolutionIf the coefficient of 2nd, 3rd and 4th terms in the expansion of `(1+x)^(2n)` are in A.P. , show that `2n^2-9n+7=0.`
View SolutionThe sum of four numbers in G.P is 60 and the arithmetic mean of the first and the last numbers is 18 . Find the numbers .
View SolutionAn original frequency table with mean `10.5` and variance `9.9` was lost but the following table derived from it was found . Construct the original table .
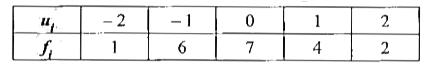
View SolutionAn original frequency table with mean `10.5` and variance `9.9` was lost but the following table derived from it was found . Construct the original table .
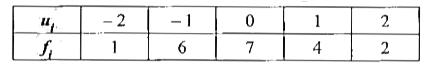
View Solution
SECTION-A questions and complete solutions for chapter MODEL TEST PAPER-6 of S CHAND MATHS (ENGLISH) of Class 11