Complete Solutions to SECTION -A of chapter MODEL TEST PAPER -13 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL TEST PAPER -13 |
| VIVA PUBLICATION | SECTION -A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
In set -builder form the null set is represented by
View SolutionEvaluate : ` tan ""(pi )/(12) .tan ""( pi )/(16) .tan ""(5pi )/(12) .tan ""( 7pi )/(16)`
View SolutionIf ` cos 18^(@) - sin 18^(@) = k sin 27^(@) , ` then k =
View SolutionIf` A_1 and A_2 ` are two AMs between a and b then ` (2A_1 -A_2) (2A_2 - A_1) = `
View SolutionIf ` ( 1+i) (1+ 2i) (1+ 3i) …(1 +ni) = x+ iy, `then ` 2,5,10 ….( 1+n^(2)) = x^(k) +y^(k) ` The value of k is :
View SolutionConstant term in the expansion of ` ( x- (1)/(x)) ^(10) ` is
View SolutionSolution of ` | 3x- 2 |le (1)/(2)` is
View SolutionThe angle between the lines ` 2x- y +3=0 and x+ 2y +3=0` is
View SolutionIf (x, 3) and ( 3,5) are the extremities of a diameter of a circle with centre at (2,y ) , then the values of x and y are
View SolutionIf ` underset( x to a ) lim (x^(5) - a^(5))/( x-a) = 405` , then possible values ( s ) of a:
View SolutionIf `f : R to R ` defined by `f ( x) = x^(2) + 1` , then find ` f^(-1) (-3) `
View SolutionA round table coference is to be held between 20 delegates. How many seating arrangements are possible if two particular delegates are always to sit together .
View SolutionIf the middle term in the expansion of `( (2)/(3) x^(2) - (3)/(2x)) ^(20) is^(20) C_10 x^(k)` , then find the value of k .
View SolutionIf `f(x) = log _(x^(2) ) x^(3) ` write the value of `f'(x)`
View Solutionabout to only mathematics
View SolutionIf ` A xx B ={ (p,q) ,(p,r) ,(m,q) , (m,r) } ` find A and B .
View SolutionTwo finite sets have m and n elements respectively . The total number of subsets of first set is 56 more than the total number of subsets of the second. Find the values of m and n.
View SolutionSolve : ` tan 3theta tan 2 theta = 1`
View SolutionIn any triangle ABC ,prove that ` (a-b cos C)/( c-b cos A ) = (sin C ) /( sin A)`
View SolutionFind the value of ` cos {npi +(-1) ^(n) (pi)/(3)} `
View SolutionFind the modulus and amplitude of ` ( 2+ i)/( 4i +(1+i) ^(2))`
View SolutionForm a quadratic equations defined over rational coefficients whose one root is sin ` 18^(@) `
View SolutionFind the domain of the functions ` f(x) = (1)/(sqrt( 4x^(2)-1)) +log _e (x(x^(2) -1))`
View SolutionIf ` cos theta = ( cos phi -e)/( 1- e cos phi ) ,` show that tan ` ""(theta)/( 2) = +- sqrt((1+e)/( 1-e)) tan ""(phi)/(2)`
View SolutionShow that ` ( sin 5A + 2 sin 8A + sin 11A ) /( sin 8 A +2sin 11A + sin 14A ) = (sin 8A)/( sin 11A) `
View SolutionUsing principle of mathematical induction , prove that `n^(3) - 7n +3` is divisible by 3 , for all n belongs to N .
View SolutionFind the co-ordinates of the points on the curve `y= (x)/( 1-x^(2)) " for which " (dy)/( dx)= 1 `
View SolutionEvaluate ` underset( x to pi ) lim ""( sin 3 x- 3sin x )/( (pi - x)^(3))`
View SolutionShow that if A and G .are A.M. and G.M. between two positive numbers , then the numbers are `A+- sqrt(A^(2) -G^(2))`
View SolutionIf the sum to infinity of the series
` 1- ( 1+d) (1)/(3) +(1+ 2d) (1)/(9) -( 1+3d) .(1)/( 27) + ………" is" (9)/(16) `, find d.
View SolutionFor what values of a is the inequality ` (x^(2) +ax-2)/( x^(2) -x+1) lt 2` satisfied for all real values of x?
View SolutionA line is drawn perpendicular to` 5x= y+7.` Find the equation of lines if the area of the triangle formed by this line with coordinate axes is 5sq. Units.
View SolutionFind the locus of the point of intersections of perpendicular tangents to the circle `x^(2) +y^(2) =a^(2)`
View SolutionThe number of faults on the surface of each of 1000 tiles were distribhuted as follows:
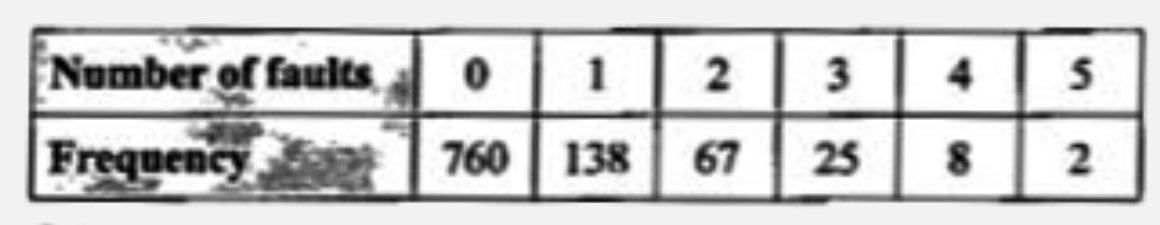
Calculate coefficient of variations:
View Solution
SECTION -A questions and complete solutions for chapter MODEL TEST PAPER -13 of S CHAND MATHS (ENGLISH) of Class 11