Complete Solutions to SECTION A of chapter MODEL TEST PAPER 14 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL TEST PAPER 14 |
| VIVA PUBLICATION | SECTION A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
The number of subsets of a set containing n elements is
View SolutionIf tan ` x= (3)/(4) " where " pi lt x lt (3pi)/( 2), ` value of tan ` ""(x)/(2)` is
View SolutionValue of sin `(-(11pi )/( 3)) ` is
View SolutionLet ` A_1 , A_2 …..,A_3 ` be n arithmetic means between a and b. Then the common difference of the AP is
View SolutionThe quadratic equation whose one root is ` - (i)/(4)` :
View SolutionThe distance between the complex numbers ` 2 +i and -3 + 5i ` is
View SolutionIf `f (x) = (x-1)/( x+1), ` then
View SolutionThe distance of the point (1,-1) from the line 12(x-6) = 5( y+2) is
View Solution` underset( x to 0 ) lim (sin ^(2) 2x)/( sin ^(2) 4x) ` is equal to
View SolutionThe area of the circle passing through (-2,6) and having its centre at (1,2) is
View SolutionFind the real numbers x,y such that `( iy+ x)(3+2i) = 1+ i`
View SolutionHow many 4-digit numbers can be formed with the digits 9,8,7,5,3 when a digit may be repeated any number of times in any arrangement.
View SolutionWrite the middle term in the expansion of `(x-(1)/(2y) ) ^(10)`
View SolutionIf `y = ( tan x)/( 1+ tan^2 x), `Prove that `(dy)/(dx) = cos 2x. `
View SolutionIf E and F are two events such that `P(E) =(1)/(4) ,P(F) = (1)/(2) and P(E nn F) = (1)/(8) , `then find `P( E' nn F')`
View SolutionLet f: ` R to R ` be a function defined as f (x) = 3x+ 7 , ` x in R ` . Show that f is an onto functions.
View SolutionIn a certain examination , the number of candidates who appeared in the examination is 1000 out of which 650 appeared in English and 200 appeared in both English and Hindi . Find
The number of candidates who offered paper in Hindi
(ii) The number of candidates who offered paper in English only.
View SolutionSketch the graph of cos 4x.
View Solutionshow that sin ` 55 ^(@) sin 25^(@) + cos 70^(@) .cos 10 ^(@) =( (sqrt3+1))/( 4)`
View SolutionProve that ` sqrt((2 sin 2x- sin 4x)/( 2 sin 2x+ sin 4x))= tan x .`
View SolutionIf `alpha and beta ` are the roots of the equation` 3x^(2) -4x+ 1 =0` ,find the equation whose roots are `(alpha^(2))/( beta) and ( beta^(2))/( alpha )`
View SolutionFind the square roots of : 4ab - `2i( a^(2) - b^(2)) `
View SolutionLet A =`( 1,2,3,4,……250)` and R be the relation " is cube of " in A. Find R as subset of `A xx A ` Also find the domain and range of R.
View SolutionProve that `tan 9^(@) - tan 27^(@) -tan 63^(@) + tan 81^(@) =4`
View SolutionIn any triangle , prove that ` ((b-c)/( b+c)) cot ""(A)/(2) +((b+c)/(b-c)) tan ""(A)/(2) = 2 cosec (B-C) `
View SolutionIf sum to infinity of the series ` 3- 5r +7r^(2) -9r^(3) + ….." is " (14)/(9) .` Find r.
View SolutionFind from first principles differential coefficients of ` sqrt( x^(2)+1) `
View SolutionEvaluate : ` underset( x to prop) lim (2x^(2) + 7x+ 5)/( 4x^(2) +3x- 1 ) `
View SolutionUsing mathematical induction, prove that
` (1)/(1.3.5) + (2)/(3.5.7) +….+(n)/((2n-1)( 2n+1) ( 2n+3)) =( n(n+1))/( 2(2n+1) (2n+3))`
View SolutionProve by using the principal of mathematical inductions
` 4+ 44 +444+ ….+ 444…4 ( n ` digits ) = `( 4)/(81) (10 ^(n+1) - 9n -10)`
View SolutionIf A be the sum of odd terms and B be the sum of even terms in the expansion of `(x+a)^(n) `, prove that `A^(2) -B^(2) = (x^(2) - a^(2)) ^(n)`
View SolutionThe slope of a straight line through A(3,2) is `(3)/(4) ` .Find the coordinates of the points on the line that are 5 units away from it.
View SolutionShow that the points ( 1,0),(2,-7) , (8,1) and (9,-6) are concyclic.
View SolutionThe batting scores of two cricket players A and B in 10 innings are as follows:
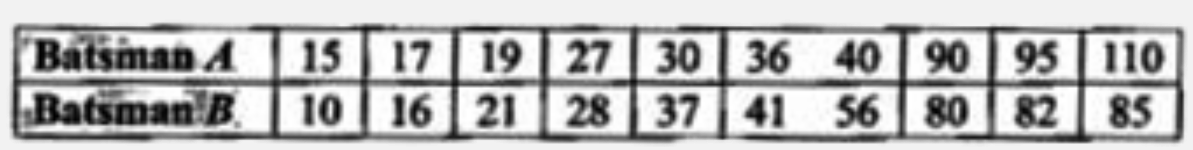
Which of the players is consistent.
View Solution
SECTION A questions and complete solutions for chapter MODEL TEST PAPER 14 of S CHAND MATHS (ENGLISH) of Class 11