Complete Solutions to SECTION-A of chapter MODEL TEST PAPER -16 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL TEST PAPER -16 |
| VIVA PUBLICATION | SECTION-A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
For any two sets A and B, A' – B'=
View SolutionIf a, b are positive real numbers, then `|x|le a hArr`
View SolutionIf the arcs of the same length in two circles subtend angles `65^(@)` and `110^(@)` at the centre then the ratio of the radii of the circles is
View SolutionThe number of values of `theta` in `[0, 2pi]` that satisfies the equation `sin^(2)theta - cos theta = (1)/(4)`
View SolutionIf the sum of n terms of an A.P. is `2n^(2)+5n`, then its `n^(th)` term
View SolutionValue of `i^(37) +(1)/(i^(67))`
View Solution`4^(th)` term from end in the expansion of `((3)/(x^(2)) -(x^(3))/(6))^(7)` is `k x^(6)`, then the value of k is
View SolutionThe least positive integer n such that `((2i)/(1+i))^(n)` is a positive integer is
View SolutionThe angle between the line x = a and `by+c= 0`, is
View SolutionThe coordinates of the centre of the circle inscribed in the square formed by the lines x=2, x=6, y=5 and y=9 is:
View SolutionEvaluate : `lim_(x to 1) (x^(m)-1)/(x^(n)-1)`
View SolutionGiven `f(x)=(1)/(secx - tan x)`, prove that `f'(x)= sec x (sec x +tan x)`
View SolutionThere are three events A, B, and C, one of which one and only one can happen. The odds are 7 to 4 against A and 3 to 5 favour of B. Find the odds against C.
View SolutionLet P(n) be the statement "7 divides `2^(3n)-1`." What is `P(n+1)`?
View SolutionFind the number of terms in the expansion of `(1+(x^(2))/(4)-x)^(5)` .
View SolutionProve that for a null set `phi, n{P(P(phi))}=2`.
View SolutionA function f is defined on the set of real numbers as follows:
`f(x)={(x+1, 1 le x lt 2),(2x-1, 2 le x lt 4), (3x-10, 4 le x lt 6):}`
(a) Find the domain of the function.
(b) Find the range of the function.
View SolutionThe angles of a triangle ABC are in A.P. and it is being given that `b:c= sqrt(3) : sqrt(2)`, find `angleA`.
View SolutionEvaluate : `"tan"(2pi)/(9)+"tan"(pi)/(9) + sqrt(3) "tan"(2pi)/(9) "tan"(pi)/(9)`
View SolutionIf `theta=(pi)/(7), ` show that `cos theta cos 2theta cos 3 theta = (1)/(8)`.
View SolutionExpress in polar form, hence find the amplitude: `z=-1-i.`
View SolutionFind k so that the roots of the equation `(x^(2)-qx)/(px-r) = (k-1)/(k+1)` may be equal in magnitude but opposite in sign.
View SolutionRedefine the function `f(x)= |1+x| +|1-x|, -2 le x le 2`.
View SolutionEvaluate : `cos^(2)76^(@) +cos^(2)16^(@) - cos76^(@).cos16^(@)`
View SolutionIf `sin alpha.sinbeta - cos alpha.cos beta+1=0`, then find the value of `cot alpha.tan beta` .
View SolutionIf `y sqrt(x^(2)+1)= log (sqrt(x^(2)+1)-x)`, prove that `(x^(2)+1)(dy)/(dx) +xy+1=0`.
View SolutionHow many words each of 3 vowels and 2 consonants can be formed from the letters of the word INVOLUTE so that consonants never together?
View Solution12 persons are invited for a party. In how many different ways can they and the host be seated at a circular table, if two particular persons are to be seated on either side of the host?
View SolutionProve that `(6x^(2)-22x+21)/(5x^(2)-18x +17)` for real values of x lies between 1 and `(5)/(4)`.
View SolutionGiven that `alpha and beta` are the roots of the equation `2x^(2)-3x+4=0`, find an equation whose roots are `alpha+(1)/(alpha) and beta+(1)/(beta)`.
View SolutionFind the `n^(th)` term of the series `2+(5)/(9)+(7)/(27) +`…… . Hence find sum to n terms.
View SolutionCalculate the co-ordinates of the foot of the perpendicular from the point(-4, 2) to the line 3x +2y=5. Also find the equation of the smallest circle passing through (-4, 2) and having its centre on the line 3x + 2y=5.
View SolutionA triangle is formed by the lines whose equations are `3x+4y-6=0,12x-5y-3 = 0 and 4x-3y + 12 = 0`. Find the internal bisector of the angle opposite to the side `3x + 4y -6=0.`
View SolutionA purchasing agent obtained samples of 60 watt bulbs from a standard company. He had the samples tested in his own laboratory for length of life with following results:
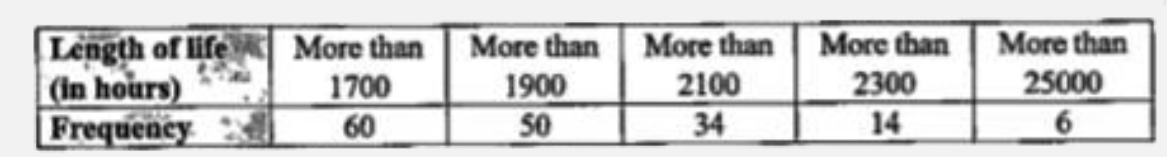
Find the standard deviation for these samples.
View Solution
SECTION-A questions and complete solutions for chapter MODEL TEST PAPER -16 of S CHAND MATHS (ENGLISH) of Class 11