Complete Solutions to EXAMPLE of chapter RELATIONS AND FUNCTIONS of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | RELATIONS AND FUNCTIONS |
| S CHAND | EXAMPLE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
If `A={a,b}`, then all possible ordered paris are (a,a) (b,b) (a,b) (b,a).
View SolutionIf `A={a,b,c}` and `B={1,2,3,4}`. Then all possible ordered pairs such that in each ordered pair the first component is an element of set A and second component is an element of set B are obtained by pairing each element from set A with each element form set B. This can be done by the following scheme:
View SolutionExpress `{(x,y):x^(2)+y^(2)=25, "where" x,y inW}` as a set of ordered pairs.
View SolutionThe number of elements in the set `{(a,b):2a^(2)+3b^(2)=35,a,b,inZ}`, where Z is set of all integers is
View SolutionIf `A={a,b}` and `B={1}`, then `AxxB={(a,1),(b,1)}` and `BxxA={(1,a),(1,b)}`
View SolutionIf `A={2,4,6}`, then `A^(2)=AxxA={(2,2)(2,4),(2,6),(4,2),(4,4),(4,6),(6,2),(6,4),(6,6)}`
View SolutionIf `A xxB = {(a , x), (a , y), (b , x), (b , y)}`. Find A and B.
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `AxxA`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `AxxB`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `BxxA`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `(BxxB)`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `n(AxxA)`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `n(AxxB)`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `n(BxxA)`
View SolutionLet `A={x inN:xle4}` and `B={y inN:3ltyle5}`. Find out `n(AxxB)`
View SolutionIf `A={1,2,3},B={4,5},C={1,2,3,4,5}` find `(i)AxxB (ii) CxxB (iii) BxxB` Hence prove that `(CxxB)-(AxxB)=BxxB`.
View SolutionIf each one of the two sets A and B has 3 elements, how many elements are there in `AxxB?` If three of the elements in `AxxB` be (2,3),(4,4) and (6,6), find the remaining elements.
View SolutionIs `Axxphi` the empty set or not, where `phi` denotes the empty set and A is any set?
View SolutionLet `A={1,2},\ B={1,2,3,4},\ C={5,6}a n d\ D={5,6,7,8}` . Verity that: `Axx(BnnC)=(AxxB)nn(AxxC)`
View SolutionLet `A={1,2),B={1,2,3,4},C={5,6}` and `D={5,6,7,8}` verify that
`AxxC` is a subset of `BxxD`.
View SolutionIf `A={1,4}B={2,3,6}` and `C={2,3,7}` verify that `Axx(B-C)=(AxxB)-(AxxC)`.
View SolutionIf `AsubeB ,` and `CsubeD ,` prove that `AxxCsubeBxxDdot`
View SolutionTaking `A={1,2,3,4},B={4,5}` in Ex.8, represent `AxxB` and `BxxB` pictorially, using arrows.
View SolutionTaking `A={1,3,5,7}` and `B={2,4,6}`, draw the graphs of (i) `AxxA` (ii) `BxxA`.
View SolutionIf `A={-1,1}`, find `AxxAxxA`.
View SolutionLet R be the set of real numbers. What does `(RxxRxxR)` represent?
View SolutionLet R be the relation from `A={1,2,3,4,5,6}` to `B={1,3,5}` which is defined by the open sentence ''x is less than y''.
(i) Find the solution set of R, that is write R as set of ordered pairs.
(ii) Plot R on a co-ordinate diagram of `AxxB`.
(iii) state the domaing, range and codomain of R.
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(a,y),(a,z),(c,x),(d,y)}`
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(a,x),(b,y),(c,x),(a,d)}`
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(a,x),(y,d),(x,c)}`
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(y,a),(z,a),(z,c),(y,d)}`
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(a,x),(x,a),(b,y),(y,b)}`
View SolutionLet `A={a,b,c,d}` and `B={x,y,z}`. Which of the following are relations from A to B? `{(a,x),(b,y),(c,z),z}`
View SolutionIf `A={x,y,z}` and `B={1,2}`, then the number of relations from A to B is
View SolutionDetermine the domain and range of the following relations : `{(-3,1),(-1,1),(1,0),(3,0)}`
View SolutionDetermine the domain and range of the following relations : `{(x,y):x" is a multiple of 3 and y is a multiple of 5"}`
View SolutionDetermine the domain and range of the following relations : `{(x,x^(2)):" x is a prime numbers less than 15"}`
View SolutionLet N be the set of nutural numbers. Deseribe the following relation in words giving its domain and the range.
`{(1,1),(16,2),(81,3),(256,4)}`
View SolutionI is the set of integers. Deseribe the following relations in words, giving its domain and range.
`{(0,0),(1,-1),(2,-2),(3,-3)......}`
View SolutionLet `A={1,2,3,4,6}`. Let R be the relation on A defined by `{(a,b)=ainA,binA," a divides b"}`. Find
(i) R (ii) domain of R (iii) range of R
View SolutionIf `A={1,2,3,4},B={5,6,7,8}`, then which of the following are relations from A to B? Give reasons for your answer. `R_(1)={(1,5),(2,7),(3,8)}`
View SolutionIf `A={1,2,3,4},B={5,6,7,8}`, then which of the following are relations from A to B? Give reasons for your answer. `R_(2)={(6,2),(3,7),(4,7)}`
View SolutionLet `A = {x , y , z} a n d B = {1, 2}`. Find the number of relations from A to B.
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
`{(1,-2),(3,7),(4,-6),(8,11)}`
View SolutionWhich of the following relation are functions? Give reasons. In cane of a function, determine its domain and range.
`{(1,0),(1,-1),(2,3),(4,10)}`
View SolutionWhich of the following relation are functions? Give reasons. If it is a function, determine its domain and range.
`{(a,b),(b,c),(c,d),(d,e)}`
View SolutionState whether or not each of the following diagrams defines a function
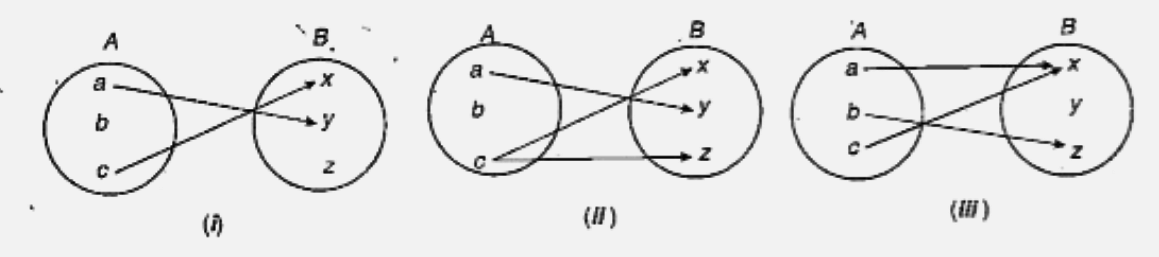
View SolutionWhich of the following relations are functions ? y=3x+2.
View SolutionWhich of the following relations are functions ?
a is the capital of b where `binB` and B is the set of all countries, `ainA` and A is the set of capital cities of countries.
View SolutionWhich of the following relations are functions ? `yltx+3`.
View SolutionWhich of the following relations are functions ?
y is the Maths teacher of x where x represents any pupll taking up Maths in a school.
View SolutionWhich of the following relations are functions ?
y is a Maths pupil of x, where x represents any Maths teacher in a school.
View SolutionState the domain of these functions :
`f:xto5x`
View SolutionState the domain of these functions :
`g:xto5x,x inZ`
View SolutionState the domain of these functions :
`h(x):(2)/(x-7)`
View SolutionState the domain of these functions :
`F:xto5x,x in{0,1,2}`
View SolutionState the domain of these functions :
`f:xto(x)/(5)`
View SolutionState the domain of these functions :
`F:xto(6)/(x)`
View SolutionState the domain of these functions :
`H:xtox^(2)+5x-6`
View SolutionState the domain of these functions :
`g: x to (x-4)/((x-3)(x+6))`
View SolutionState the domain of these functions :
`g:xto(x)/(1),x in{2,4,6}`
View SolutionState the domain of these functions :
`g:xto(1)/(x),x inR`
View SolutionDoes the relation `{(x,y)|y=|x|,x inR}` define a function? Write the range and draw the graph.
View SolutionDoes the relation 'a square root of' in the set of real numbers represent a function ?
View SolutionFind whether the following functions are one-one or not.
`f:RtoR`, defined by `f(x)=x^(3),x inR`
View SolutionFind whether the following functions are one-one or not.
`f:ZtoZ`, defined by `f(x)=x^(2)+5` for all `x inZ`
View SolutionFind whether the following functions are one-one or not.
`f:R-{3}toR`, defined by `f(x)=(5x+7)/(x-3),x inR-{3}`
View SolutionShow that the modulus function `f:RtoR`, given by `f(x)=|x|`, is not one-one function.
View SolutionProve that the greatest integer function `f:RtoR`, given by `f(x)=[x]` is a many-one function.
View SolutionFind whether the following is onto function (surjection) or not.
`f:RtoR` defined by `f(x)=x^(3)+5` for all `x inR`
View SolutionFind whether the following are into functions (surjections) or not.
`f:RtoR` defined by `f(x)=x^(2)+3` for all `x inR`
View SolutionFind whether the following are into functions (surjections) or not.
`f:ZtoZ` defined by `f(x)=5x-9` for all `x inZ`.
View SolutionIf `A=R-{3}` and `B=R-{1}` and `f:AtoB` is a mapping defined by `f(x)=(x-2)/(x-3)` show that f is one-one onto function.
View SolutionLet `f=NtoN` be defined by `f(x)=x^(2)+x+1,x inN`, then prove that f is one-one but not onto.
View SolutionLet `f:RtoR` be a function defined by `f(x)=(x-m)/(x-n)`, where `mnen`. Then show that f is one-one but not onto.
View SolutionThe function `f:RtoR` defined by `f(x)=e^(x)` is
View SolutionIf `f:RtoR` be defined as `f(x)=x^(4)`. Choose the corrent answer : (a) f is one-one onto (b)f is many-one onto (c)f is one-one but not onto (d)f is neither one-one nor onto
View SolutionLet `f:N to N` be defined by `f(n)={{:((n+1)/2, " if n is odd"),(n/2, "if n is even"):}` for all `n in N`.
Prove that f is many-one, onto function.
View SolutionIf `f(x)=(x-|x|)/(|x|)`, then find f(-1).
View SolutionIf `f(x)=(x)/(x-1)`, then prove that `(f(a))/(f(a+1))=f(a^(2))`
View SolutionIf `f(x)=(1)/(sqrt(x+2sqrt(2x-4)))+(1)/(sqrt(x-2sqrt(2x-4)))` for `xgt2` then find f(11).
View SolutionIf `f(x)=(1+x)/(1-x)`, show that `f|f(tantheta)]=-cottheta.`
View SolutionIf `f(x)=cos(logx)`, then `f(x^(2))f(y^(2))-(1)/(2)[f(x^(2)y^(2))+f((x^(2))/(y^(2)))]=`
View SolutionIf `f(x)={{:(2x-1,"when "xle0),(x^(2),"when "xgt0):},"then find "f((1)/(2)) and f((-1)/(2))`
View SolutionIf `f(x)={{:(1+x",",-1lexlt0),(x^(2)-1",",0ltxlt2),(2x",",2lex):}`
Find `f(3),f(-2),f(0),f((1)/(2))f(2-h),f(-1+h)`, where `hgt0` is very small.
View SolutionGraph the following piece wise-defined function :
`{{:(-x",",xlt0),(x^(2)",",0lexle1),(1",",xgt1):}`
View SolutionExamine whether `x((a^(x)+1)/(a^(x)-1))` is an odd or even function.
View SolutionThe function `f(x)=sin(log(x+ sqrt(x^2+1)))`
View SolutionDetermine whether the function is even, odd or niether even or odd.
(a) `f(x)=5-x^(2)` (b) `f(x)=|-x|` (c ) `f(x)=[x]` (d) `f(x)=|x-2|` (e) `f(x)=-x|x|`
View SolutionIf f is an odd function and f(0) is defined, must f(0)=?
View SolutionIf `f(x)=x^(2)+kx+1` for all x and f is an even function, find `k,kinR`.
View SolutionThe function `f(x)=sinx+cosx` will be
View SolutionIf f is a real function, find the domain of
`f(x)=sqrt((a^(2)-x^(2)))`
View SolutionIf f is a real function, find the domain of
`f(x)=(1)/(3x+2)`
View SolutionIf f is a real function, find the domain of
`f(x)=(1)/(log|x|)`
View SolutionIf f is a real function, find the domain of
`f(x)=10^(-x)`
View SolutionFind the domain of
`sqrtx+sqrt(2x-1)`
View SolutionFind the domain of
`log(x-2)-sqrt((3-x))`
View SolutionFind the domain of the following functions.
`f(x)=log_(3+x)(x^(2)-1)`
View SolutionFind the domain of the following functions.
`f(x)=(sin^(-1)(3-x))/(ln(|x|-2))`
View Solution
EXAMPLE questions and complete solutions for chapter RELATIONS AND FUNCTIONS of S CHAND MATHS (ENGLISH) of Class 11