Complete Solutions to EXERCISE of chapter MODEL PAPER (12) of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL PAPER (12) |
| JBD PUBLICATION | EXERCISE |
| Number Of Questions | 40 |
| Academic Year | 2023-24 |
`A cap (AcupB)'` is equal to:
View SolutionIf `f(x)=(x-1)/(x+1)`, then:
View SolutionThe value of `sinpi/14 sin(3pi)/14 sin(5pi)/14` is equal to:
View SolutionIf z is purely real number such that `Re(z)<0`, then argument (z) is equal to:
View SolutionThe number of ways in which n distinct objects can be put into two different boxes is:
View SolutionThe G.M. between -2i and 8i is:
View SolutionA line passes through the point (2,2) and is perpendicular to the line `3x+y=3` , then its y - intercept is
View SolutionThe eccentricity of the hyperbola whose latus rectum is half of its transverse axis is:
View Solution`underset(xrarr0)lim x/(tanx)` is equal to:
View SolutionIf A and B are mutually exclusive events then:
View SolutionProve that : `(tan (pi/4+x))/(tan (pi/4-x))= ((1+tanx)/(1-tanx))^2`.
View SolutionProve the following: `cos((3pi)/2+x)cos(2pi+x)[cot((3pi)/2-x)+cot(2pi+x)]=1`
View SolutionExpress: `(-i)(2i)(-1/8i)^3` in the form of a+ib.
View SolutionFind the fourth term from the end in the expansion of `[x^3/2-2/x^3]^9`.
View SolutionShow that `9^(n+1) - 8n -9` is divisible by 64, whenever n is a positive integer.
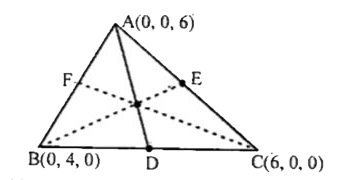
View SolutionFind the lenghts of the medians of the triangle with vertices A(0,0,6), B(0,4,0) and C(6,0,0)
View SolutionFind out which of the following sentences are statements and which are not justify your answer
A triangle has four sides.
View SolutionFind out which of the following sentences are statements and which are not justify your answer
Do you work.
View SolutionFind the component statement of the following and check whether they are true or not.
A person who has taken mathematics or computer science can go for MCA.
View SolutionLet A={a,b,c} and B={1,2,3}. Find the number of relations from A into B.
View SolutionShow tha:
`cos6A=32cos^6A-48cos^4A+18cos^2A-1`
View Solution`1^2+2^2+3^3+.....+n^2=(n(n+1)(2n+1))/(6)`
View SolutionIf `^nC_3=56` and `^nP_3=336`, find n .
View SolutionThe English alphabet has 5 vowels and 21 consonants. How many words with two different vowels and 2 different consonants can be formed from the alphabet ?
View SolutionThe sum of two numbers is 6 times their G.M. show that numbers are in the ratio `3+2sqrt2: 3-2sqrt2`.
View SolutionBetween 1 and 31, n A.M's have been inserted in sucha a way that the ratio of 7th and (m-1)th means is 5:9, find the value of m.
View SolutionIn which ratio is the line joining the points (1,3) and (2,7) is divided by the line 3x+y=9.
View SolutionFind the equation of hyperbola whose foic are `(0, pm12)` and length of latus rectum is 36.
View SolutionEvaluate:`underset(xrarr0)lim (1-cos2x)/(3tan^2x)`
View SolutionDifferentiate: `(acos x+bsinx+c)/(sinx)` w.r.t.x.
View SolutionThree coins are tossed once. Find the probability of getting
at least two heads.
View SolutionThree coins are tossed once. Find the probability of getting
at most two tails.
View SolutionIf `(x + iy)^3 = u + iv`, then show that `u/x +v/y = 4(x^2 - y^2)`
View SolutionFind the square root fo `1 - i`.
View SolutionFind all pairs of consecutive odd positive integers both of which are smaller than 10 such that their sum is more than 11.
View SolutionSolve the given inequality `-5 < (x - 2)/5 le 0`
View SolutionFind `underset(xrarr0)f(x)` where `f(x)={(2x+3, xle0),(3(x+1), x>0):}`
View SolutionFind the derivative of (sin x + cos x) from the first principle.
View SolutionFind the mean deviation from mean:

View SolutionFind the mean for the following data:
13,17,16,14,11,13,10,16,18,12,17
View Solution
EXERCISE questions and complete solutions for chapter MODEL PAPER (12) of JBD PUBLICATION of Class 11