Complete Solutions to SECTION-D of chapter MACHANICAL PROPERTIES OF SOLIDS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MACHANICAL PROPERTIES OF SOLIDS |
| KUMAR PRAKASHAN | SECTION-D |
| Number Of Questions | 29 |
| Academic Year | 2023-24 |
Modulus of rigidity of an ideal liquid is ......
View SolutionThe maximum load a wire can withstand without breaking when its length is reduced to half of its original length, will .......
View SolutionIf the temperature of a wire is doubled then Young's modulus of elasticity ......
View SolutionA spring is stretched by applying a load to its free end. The strain produced in the spring is ......
View SolutionA rigid bar of mass M is supported symmetrically by three wires each of length 1. Those at each end are of copper and the middle one is of iron. The ratio of their diameters, if each is to have the same tension, is equal to ......
View SolutionA mild steel wire of length 2L and crosssectional area A is stretched, well within elastic limit, horizontally between two pillars as shown in figure. A mass m is suspended from the mid point of the wire, the mid point lower by distance x. Then strain in the wire is ......

View SolutionA rectangular frame is to be suspended symmetrically by two strings of equal length on two supports as shown in figure. It can be done in one of the following three ways, The tension in the strings will be

View SolutionConsider two cylindrical rods of identical dimensions, one of rubber and the other of steel. Both the rods are fixed rigidly at one end to the roof. A mass M is attached to each of the free ends at the centre of the rods.
View SolutionThe stress-strain graphs for two materials are shown in figure. (Assume same scale).
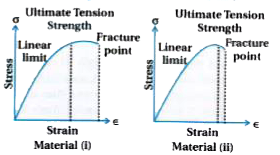
View SolutionA wire is suspended from the ceiling and stretched under the action of a weight F suspended from its other end. The force exerted by the ceiling on it is equal and opposite to the weight.
View SolutionA rod of length 1 and negligible mass is suspended at its two ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths as shown in figure. The cross-sectional areas of wires A and B are `1.0 mm^(2) and 2.0 mm^(2)` respectively. `Y_("steel”) = 200 xx 10 ^(9) Nm ^(-2) and Y_("auminium”) = 70 xx 10 ^(9) Nm ^(-2)`

View SolutionFor an ideal liquid
View SolutionA copper and a steel wire of the same diameter are connected end to end. A deforming force Fis applied to this composite wire which causes a total elongation of 1 cm. The two wires will have
View SolutionThe Young's modulus for steel is much more than that for rubber. For the same longitudinal strain, which one will have greater tensile stress ?
View SolutionThe Young's modulus for steel is much more than that for rubber. For the same longitudinal strain, which one will have greater tensile stress ?
View SolutionIdentical springs of steel and copper are equally stretched. On which more work will have to be done?
View SolutionWhat is the Young's modulus for a perfect rigid body?
View SolutionWhat is the bulk modulus of a perfectly rigid body?
View SolutionA wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force f its length increases by l. Another wire of the same material of length 2L and radius 2r is pulled by a force 2f. Then find the increase in length of this wire.
View SolutionA steel rod of length 1 m and area of cross section `1 cm^(2)` is heated from `0^(@)C` to` 200^(@)C` without being allowed to extend or bend. Find the tension produced in the rod `(Y=2.0 xx 10 ^(11) Nm ^(-1) , alpha = 10 ^(-5) ^(@)C ^(-1))`
View SolutionTo what depth must a rubber ball be taken in deep sea so that its volume is decreased by 0.1% (The bulk modulus of rubber is `9.8 xx 10 ^(8) N//m^(2)`and density of sea water is `10 ^(3) kgm^(-3))`
View SolutionA truck is pulling a car out of a ditch by means of a steel cable that is 9.1 m long and has a radius of 5 mm, when the car just begins to move the tension in the cable is 800 N. How much has the cable stretched ? (Young's modulus for steel is `2 xx 10 ^(11) Nm ^(-2))`
View SolutionTwo identical solid balls, one of ivory and the other of wet-clay are dropped from the same height on the floor. Which one will rise to a greater height after striking the floor and why?
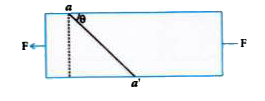
View SolutionConsider a long steel bar under a tensile stress due to force F acting at the edges along the length of the bar (as shown in figure). Consider a plane making an angle with the length. What are the tensile and shearing stresses on this plane?
(a) For what angle is the tensile stress a maximum ?
(b) For what angle is the shearing stress a maximum ?
View Solution(a) A steel wire of mass u per unit length with a circular cross section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming the wire to be uniform an lateral strains `lt lt` longitudinal strains find the extension in the length of the wire. The density of steel is 7860 `kgm ^(-3)` and Young's modulus `=2 xx 10 ^(11) Nm ^(-2)`
(b) If the yield strength of steel is `2.5 xx 10 ^(8) Nm ^(-2),`
what is the maximum weight that can be hung at the lower end of the wire ?
View SolutionA steel uniform rod of length 2L cross sectional area A and mass Mis set rotating in a horizontal plane about an axis passing through the centre. If Y is the Young's modulus for steel, find the extension in the length of the rod.
View SolutionAn equilateral triangle ABC is formed by two copper rods AB and BC and one is aluminium rod which heated in such a way that temperature of each rod increases by `DeltaT.` Find change in the angle `angleABC.` (Coefficient of linear expansion for copper is `alpha _(1).` and for aluminium is `alpha2`.).
View SolutionIn nature the failure of structural members usually result from large torque because of twisting or bending rather than due to tensile or compressive strains. This process of structural breakdown is called buckling and in cases of tall cylindrical structures like trees, the torque is caused by its own weight bending the structure. Thus, the vertical through the centre of gravity does not fall within the base. The elastic torque caused because of this bending about the central axis of the tree is given by `(Ypi r ^(2))/(4R) Y` is the Young's modulus, r is the radius 4R of the trunk and R is the radius of curvature of the bent surface along the height of the tree containing the centre of gravity (the neutral surface). Estimate the critical height of a tree for a given radius of the trunk.
View SolutionA stone is tied to an elastic string of negligible mass and spring constant k. The unstretched length of the string is L and has negligible mass. The other end of the string is fixed to a nail at a point P. Initially the stone is at the same level as the point P. The stone is dropped vertically from point P.
(a) Find the distance 'y' from the top when the mass comes to rest for an instant, for the first time.
(b) What is the maximum velocity attained by the stone in this drop ?
(c) What shall be the nature of the motion after the stone has reached its lowest point ?
View Solution
SECTION-D questions and complete solutions for chapter MACHANICAL PROPERTIES OF SOLIDS of KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) of Class 11