Complete Solutions to Section - B (Numericals) Numerical From Textual Exercise of chapter THERMAL PROPERTIES OF MATTER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | THERMAL PROPERTIES OF MATTER |
| KUMAR PRAKASHAN | Section - B (Numericals) Numerical From Textual Exercise |
| Number Of Questions | 26 |
| Academic Year | 2023-24 |
The triple points of neon and carbon dioxide are 24.57 K and 216.55 respectively. Express these temperatures on the Celsius and Fahrenheit scales.
View SolutionTwo absolute scales A and B have triple points of water defined to be 200 A and 350 B. What is the relation between `T_(A)` and `T_(B)` ?
View SolutionThe electrical resistance in ohms of a certain thermometer varies with temperature according to the approximate law :
`R=R_(0)[1+alpha(T-T_(0))]`
The resitance is `101.6" "Omega` at the triple-point of water 273.16 K, and `165.5" "Omega` at the normal melting point of lead (600.5 K). What is the temperature when the resistance is `123.4" "Omega` ?
View SolutionTwo ideal gas thermometers A and B use oxygen and hydrogen respectively. The following observations are made :
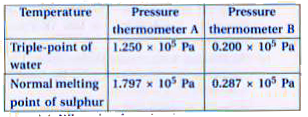
What is the absolute temperature of normal melting point sulphur as read by thermometers A and B ?
View SolutionTwo ideal gas thermometers A and B use oxygen and hydrogen respectively. The following observations are made :

What do you think is the reason behind the slight difference in answers of thermometers A and B ? (The thermometers are not faulty). What further procedure is needed in the experiment to reduce the discrepancy between the two readings ?
View SolutionA steel tape 1 m long is correctly calibrated for a temperature of `27.0^(@)C`. The length of a steel rod measured by this tape is found to be 63.0 cm on a hot day when the temperature is `45.0^(@)C`. What is the actual length of the steel rod on that day ? What is the length of the same steel rod on a day when the temperature is `27.0^(@)C` ? Coefficient of linear expansion of steel `=1.20xx10^(-5)K^(-1)`.
View SolutionA large steel wheel is to be fitted on to a shaft of the same material. At `27^(@)C`, the outer diameter of the shaft is 0.70 cm and the diameter of the central hole in the wheel is 8.69 cm. The shaft is cooled using 'dry ice'. At what temperature of the shaft does the wheel slip on the shaft ? Assume coefficient of linear expansion of the steel to be constant over the required temperature range :
`alpha_("steel")=1.20xx10^(-5)K^(-1)`.
View SolutionA hole is drilled in a copper sheet. The diameter of the hole is 4.24 cm at `27.0^(@)C`. What is the change in the diameter of the hole when the sheet is heated to `227^(@)C` ? Coefficient of linear expansion of copper `=1.70xx10^(-5)K^(-1)`.
View SolutionA steel wire `0.72` m long has a mass of `5.0 xx 10 ^(-3) kg.` If the wire is under a tension of 60 N, what is the speed of transvers waves on the wire ?
View SolutionA brass rod of length 50 cm and diameter 3.0 mm is joined to a steel rod of the same length and diameter. What is the change in length of the combined rod at `250^(@)C`, if the original lengths are at `40.0^(@)C` ? there a 'thermal stress' developed at the junction ? The ends of the rod are free to expand (Coefficient of linear expansion of brass `=2.0xx10^(-5)K^(-1)," steel "=1.2xx10^(-5)K^(-1)`).
View SolutionThe coefficient of volume expansion of glycerin is `49xx10^(-5)K^(-1)`. What is the fractional change in its density for a `30^(@)C` rise in temperature ?
View SolutionA 10 kW drilling machine is used to drill a bore in a small aluminium block of mass `8.0` kg. How much is the rise in temperature of the block in 2.5 minutes, assuming `50%` of power is used up in heating the machine itself or lost to the surroundings. Specific heat of aluminium `=0.91" Jg"^(-1)K^(-1)`.
View SolutionA copper block of mass 2.5 kg is heated in a furnace to a temperature of `500^(@)C` and then placed on a large ice block. What is the maximum amount of ice that can melt ? (Specific heat of copper `=0.39" Jg"^(-1)K"^(-1),` heat of fusion of water `=335" Jg"^(-1)`).
View SolutionIn an experiment on the specific heat of a metal, a 0.20 kg block of the metal at `150^(@)C` is dropped in a copper calorimeter (of water equivalent 0.025 kg) containing `150" cm"^(3)` of water at `27^(@)C`. The final temperature is `40^(@)C`. Compute the specific heat of the metal. If heat losses to the surroundings are not negligible, is your answer greater or smaller than the actual value for specific heat of the metal ?
View SolutionGiven below are observations on molar specific heats at room temperature of some common gases.

The measured molar specific heats of these gases are markedly different from those for monatomic gases. Typically, molar specific heat of a monatomic gas is 2.92 cal/mol K. Explain this difference. What can you infer from the somewhat larger (than the rest) value for chlorine ?
View SolutionAnswer the following questions based on the P-T phase diagram of carbon dioxide :
(a) At what temperature and pressure can the solid, liquid and vapour phases of `CO_(2)` coexist in equilibrium ?
(b) What is the effect of decrease of pressure on the fusion and boiling point of `CO_(2)` ?
(c) What are the critical temperature and pressure for `CO_(2)` ? What is their significance ?
(d) Is `CO_(2)` solid, liquid or gas at (a) `-70^(@)C` under 1 atm, (b) `-60^(@)C` under 10 atm, (c) `16^(@)C` under 56 atm ?

View SolutionAnswer the following questions based on the P - T phase diagram of `CO_(2)` :
(a) `CO_(2)` at 1 atm pressure and temperature `-60^(@)C` is compressed isothermally. Does it go through a liquid phase ?
(b) What happens when `CO_(2)` at 4 atm pressure is cooled from room temperature at constant pressure ?
(c) Describe qualitatively the changes in a given mass of solid `CO_(2)` at 10 atm pressure and temperature `-65^(@)C` as it is heated up to room temperature at constant pressure.
(d) `CO_(2)` is heated to a temperature `70^(@)C` and compressed isothermally. What changes in its properties do you expect to observe ?

View SolutionA child running a temperature if `101^(@)F` is given an antipyrine (i.e. a medicine that lowers fever) which causes an increase in the rate of evaporation of sweat from his body. If the fever is brought down to `98^(@)F` in 20 min, what is the average rate of extra evaporation caused, by the drug. Assume the evaporation mechanism to be the only way by which heat is lost The mass of the child is 30 kg. The specific heat of human body water, and latent heat of evaporation of water at that temperature is about `"580 cal g"^(-1)`.
View SolutionA 'thermacole' icebox is cheap and efficient method for storing small quantities of cooked food in summer in particular. A cubical icebox of side 30 cm has a thickness of 5.0 cam if 4.0 kg of ice is put in the box, estimate the amount of ice remaining after 6 h. The outside temperature is `45^(@)C`, and coefficient of thermal conductivity of thermacole is `0.01" Js"^(-1)m^(-1)K^(-1)`. [Heat of fusion of water `=335xx10^(3)" Jkg"^(-1)` ]
View SolutionA brass boiler has a base of `0.15m^(2)` and thickness 1.0 cm. It boils water at the rate of 6.0 kg/min when placed on a gas stove. Estimate the temperature of the part of the flame in contact with the boiler. Thermal conductivity of brass `=109" Js"^(-1)m^(-1)K^(-1),` Heat of vaporization of water `=2256xx10^(3)" Jkg"^(-1)`.
View SolutionExplain why,
a body with large reflectivity is a poor emitter.
View SolutionExplain why,
a brass tumbler feels much colder than a wooden tray on a chilly day.
View SolutionExplain why,
an optical pyrometer (for measuring high temperatures) calibrated for an ideal black body radiation gives too low a value for the temperature of a red hot iron piece in the open, but gives a correct value for the temperature when the same piece is in the temperature when the same piece is in the furnace.
View SolutionExplain why,
the earth without its atmosphere would be inhospitably cold.
View SolutionExplain why,
heating systems based on circulation of steam are more efficient in warming a building than those based on circulation of hot water.
View SolutionA body cools from `80^(@)C` to `50^(@)C` in 5 minutes Calculate the time it takes to cool from `60^(@)C` to `30^(@)C`. The temperature of the surroundings is `20^(@)C`.
View Solution
Section - B (Numericals) Numerical From Textual Exercise questions and complete solutions for chapter THERMAL PROPERTIES OF MATTER of KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) of Class 11
All Exercises from KUMAR PRAKASHAN Class 11 PHYSICS THERMAL PROPERTIES OF MATTER
- Information : Higher Order Thinking Skills (HOTS)
- Question Paper (Section - A) Answer following in one sentence : (Word limit of answer is 10) (Each question carries 1 mark)
- Question Paper (Section - B) Answer the following question in short : (Word limit of answer is 10) (Each carry 2 mark)
- Question Paper (Section - C) Do as directed : (Word limit of answer is 10) (Each carry 3 mark)
- Question Paper (Section - D) (Answer following in brief :) Each carry 4 marks
- Section - A Questions-Answer
- Section - B
- Section - B (Numericals) Numerical From Textual Exercise
- Section - B (Numericals) Numerical From Textual Exercise (Answer the following : )
- Section - B (Numericals) Numerical From Textual Illustration
- Section - B Numerical From .DARPAN. Based On Textbook
- Section - C Objective Questions (VSQs)
- Section - C Objective Questions (VSQs) Are the following Statements True or False ?
- Section - C Objective Questions (VSQs) Assertion and Reason Type Questions
- Section - C Objective Questions (VSQs) Fill in the Blank
- Section - C Objective Questions (VSQs) Match Type Questions
- Section - D NCERT Exemplar Solution (Long Answer Type Questions )
- Section - D NCERT Exemplar Solution (Multiple Choice Questions MCQs)
- Section - D NCERT Exemplar Solution (Multiple Choice Questions MCQs) More than one options
- Section - D NCERT Exemplar Solution (Short Answer Type Questions )
- Section - D NCERT Exemplar Solution (Very Short Answer Type Questions )
- Section - E Multiple Choice Questions (MCQs)
- Section - F Question from Module (Sample questions for preparation of competitive exams)
- Try Yourself (VSQs)