Complete Solutions to EXAMPLE of chapter PROBABILITY of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | PROBABILITY |
| MODERN PUBLICATION | EXAMPLE |
| Number Of Questions | 71 |
| Academic Year | 2023-24 |
In the following experiment, specify the appropriate sample space :
A person is noting down the number of accidents along a busy highway during a year.
View SolutionIn the following experiment, specify the appropriate sample space :
A boy has a 1 rupee coin, a 2 rupee coin and a 5 rupee coin in his pocket. He takes two coins out of his pocket, one after the other.
View SolutionThree coins are tossed simultaneously. Find the sample space.
View SolutionA coin is tossed and then a die is rolled only in case head is shown on the coin , describe the sample space for the experiment.
View SolutionAn experiment consists of recording boy-girl composition of families with 2 children.
What is the sample space if we are interested in knowing whether it is a boy or a girl in the order of their births ?
View SolutionAn experiment consists of recording boy-girl composition of families with 2 children.
What is the sample space if we are interested in the number of girls in a family ?
View SolutionA bag contains 4 identical red balls and 3 identical black balls. The experiment consists of drawing one ball, then putting it into the bag and again drawing a ball. What are the possible outcomes of the experiment ?
View SolutionThe numbers 1,2,3 and 4 are written separatley on four slips of paper. The slips are put in a box and mixed throughly. A person draws two slips from the box, one after the other without replacement. Describe the sample space for the experiment.
View SolutionA die is thrown repeatedly until a six comes up. What is the sample space for the this experiment?
View SolutionWrite the sample space of the following Random experiment :
A coin is tossed two times.
View SolutionThe number of elements in a sample space when a coin is tossed and a die is thrown are
View SolutionDescribe the sample space for the indicated experiment,
A coin is tossed three times.
View SolutionWrite the sample space of the following Random experiment :
A coin is tossed once.
View SolutionWrite the sample space of the following Random experiment :
A die is thrown once.
View SolutionAn experiment involves rolling a pair of dice and recording the numbers that come up. Describe the following events : A: the sum is greater than 8, B: 2 occurs on either die C: the sum is the least 7 and a multiple of 3. Also find `A nn B, B nnC` and `AnnC`.
View SolutionAn experiment involves rolling a pair of dice and recording the numbers that come up. Describe the following events : A: the sum is greater than 8, B: 2 occurs on either die C: the sum is the least 7 and a multiple of 3. Which pairs of three events are mutually exclusive?
View SolutionTwo dice are rolled. Let A, B and C be the events of getting a sum 2, a sum 3 and a sum 4 respectively.
Is event A simple ?
View SolutionTwo dice are rolled. Let A, B and C be the events of getting a sum 2, a sum 3 and a sum 4 respectively.
Is event B simple ?
View SolutionTwo dice are rolled. Let A, B and C be the events of getting a sum 2, a sum 3 and a sum 4 respectively.
Is event C compound ?
View SolutionTwo dice are rolled. Let A, B and C be the events of getting a sum 2, a sum 3 and a sum 4 respectively.
Are events A and B mutually exclusive ?
View SolutionA coin is tossed three times. Consider the following events : A : No head appears B : Exactly one head appears C : At least two heads appear. Do they form a set of mutually exclusive and exhaustive events ?
View SolutionLet a sample space be : `S={omega_(1),omega_(2),.........,omega_(n)}`. Which of the following assignments of probabilities to each outcome are valid ?
View SolutionA die is thrown, find the probability of following events:
A prime number will appear.
View SolutionA die is thrown, find the probability of following events:
A number greater than or equal to 3 will appear.
View SolutionA die is thrown, find the probability of following events:
A number less than or equal to one will appear.
View SolutionA die is thrown, find the probability of following events:
A number more than 6 will appear.
View SolutionA die is thrown, find the probability of following events:
A number less than 6 will appear.
View SolutionOne card is drawn from a well shuffled deck of 52 cards. If each outcome is equally likely, calculate the probability that the card will be: a diamond.
View SolutionOne card is drawn from a well shuffled deck of 52 cards. If each outcome is equally likely, calculate the probability that the card will be: not an ace.
View SolutionOne card is drawn from a well shuffled deck of 52 cards. If each outcome is equally likely, calculate the probability that the card will be: a black card (i.e. a club or a spade)
View SolutionOne card is drawn from a well shuffled deck of 52 cards. If each outcome is equally likely, calculate the probability that the card will be: not a black card.
View SolutionA bag contains 5 black and 3 white balls. Two balls are drawn at random. Find the probability of drawing
two black balls.
View SolutionA bag contains 5 black and 3 white balls. Two balls are drawn at random. Find the probability of drawing
two white balls.
View SolutionThe letters of the word ‘SOCIETY’ are placed at random in a row. What is the probability that the three vowels come together ?
View SolutionWhat is the chance that a non-leap year selected at random will contain 53 Sundays ?
View SolutionIn a toss of two coins, find the probability of getting both heads or both tails.
View SolutionFour coins are tossed simultaneously. Write the sample space and then complete the following table :

View SolutionA card is drawn at random from a well shuffled deck of 52 cards. If A is the event of getting a red card and the event B that a card is bearing a number greater than 2 but less than 9, find P (A) and P (B).
View SolutionTwo dice are thrown simultaneously. Find the probability of getting eight as the sum.
View SolutionTwo dice are thrown simultaneously. Find the probability of getting six as a product.
View SolutionIn a single throw of three dice, find the probability of not showing the same number on all the dice.
View SolutionA class consists of 10 boys and 8 girls. Three students are selected at random. Find the probability that the selected group has : all Boys.
View SolutionA class consists of 10 boys and 8 girls. Three students are selected at random. Find the probability that the selected group has : all girls.
View SolutionA class consists of 10 boys and 8 girls. Three students are selected at random. Find the probability that the selected group has : 2 boys and 1 girl.
View SolutionA certain team wins with probability 0.7, loses with probability 0.2 and ties with probability 0.1. The team plays three games. Find the probability that the team wins at least two of the games, but not lose.
View SolutionA bag contains 20 tickets numbered 1 to 20. Two tickets are drawn at random . Find the probability that both the numbers on the ticket are prime.
View SolutionA bag contains 8 red, 3 white and 9 blue balls. Three balls are drawn at random from the bag. Determine the probability that none of the balls is white .
View SolutionA bag contains 50 tickets numbered 1, 2, 3, .., 50 of which 5 are drawn at random and arranged in ascending order of magnitude `x_1 < x_2 < x_3 < x_4 < x_5` Find the probability that `x_3=30.`
a. `(.^20C_2times.^29C_2)/(.^50C_5)`
b. `(.^20C_2)/(.^50C_5)`
c.`(.^29C_2)/(.^50C_5)`
d. None of these
View SolutionFind the probability of 4 turning for at least once in two tosses of a fair die.
View SolutionA card is selected from a pack of 52 cards. How many points are there in the sample space ?
View SolutionA card is selected from a pack of 52 cards. Calculate the probability that the card is an ace of spades.
View SolutionOne card is drawn from a well shuffled deck of 52 cards. Calculate the probability that the card will be an ace.
View SolutionA card is selected from a pack of 52 cards. Calculate the probability that the card is : black card.
View SolutionA five digit number is formed by the digit 1,2,3,4 and 5 without repetition. Find the probability that the number formed is divisible by 4.
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have
no man?
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have
one man?
View SolutionA committee of two is selected from two men and two women. What is the probability that the committee will have : two men ?
View SolutionIf the odds in favour of an event are 4 : 5, find the probability that‘it will occur.
View SolutionThere are three events `E_1, E_2` and `E_3` one of which must, and only one can happen. The odds are 7 to 4 against `E_1` and 5 to 3 against `E_2`. Find odds against `E_3`.
View SolutionFill in the blanks in the following table :
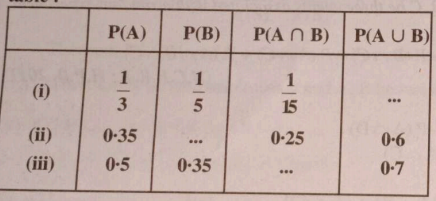
View SolutionA and B are two mutually exclusive events, for which P (A) = 0.3, P (B) = p and `P (AuuB)` = 0.5. Find the value of p.
View SolutionIn a class of 25 students with roll numbers 1 to 25, a student is picked up at random to answer a question. Find the probability that the roll number of the Selected student is either a multiple of 5 or of 7.
View SolutionTickets are numbered from 1 to 100. One ticket is picked up at random. Find the probability that the ticket picked up has a number, which is divisible by 5 or 8.
View SolutionTwo dice are toosed once. Find the probability of getting 'an even number on the first dice or a total of 8'
View SolutionIn a single throw of two dice, find the probability that neither a doublet nor a total of 10 will appear.
View SolutionIn class XI of a school 40% of the students study Mathematics and 30% study Biology 10% of the class study both Mathematics and Biology. If a student is selected at random from the class, find the probability that he will be studying Mathematics or Biology.
View SolutionA box contains 100 bolts and 50 nuts. It is given that 50% bolts and 50% nuts are rusted. Two objects are selected from the box at random. Find the probability that both are bolts or both are rusted.
View SolutionTwo students Anil and Ashima appeared in an examination. The probabilities that Anil will qualify the examination is 0.05 and that Ashima will qualify the examination is 0.10. The probabilities that both will qualify the examination is 0.02. Find the probability that
a) Both Anil and Ashima will not qualify the examination.
b) Atleast one of them will not qualify the examination.
View SolutionTwo students Anil and Ashima appeared in an examination. The probability that Anil will qualify the examination is 0.05 and that Ashima will qualify the examination is 0.10. The probability that both will qualify the examination is 0.02. Find the probability that : at least one of them will not qualify the examination.
View SolutionTwo students Anil and Ashima appeared in an examination. The probability that Anil will qualify the examination is 0.05 and that Ashima will qualify the examination is 0.10. The probability that both will qualify the examination is 0.02. Find the probability that : only one of them will qualify the examination.
View SolutionIn a given race, the odds in favour of horses A, B, C, D are 1:3, 1:4, 1:5 and 1:6 respectively. Find the probability that one of the them wins the race.
View Solution
EXAMPLE questions and complete solutions for chapter PROBABILITY of MODERN PUBLICATION of Class 11