Complete Solutions to Exercise of chapter Dynamics of Circular Motion of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | Dynamics of Circular Motion |
| MODERN PUBLICATION | Exercise |
| Number Of Questions | 81 |
| Academic Year | 2023-24 |
A bend in a level road has radius of 100 m.Find the maximum speed with which a car turning this bend may have without skidding ,if the coefficient of friction between the tyres and road is 0.8.
View SolutionThe radius of curvature of a railway track at a place where the train is moving at a speed of `72 km h^-1` is 625 m.The distance between the rails is 1.5 m.Find the angle and the elevation of the outer rail so that there may be no side pressure on the rails.`(g=9.8 m s^(-2))`.
View SolutionA cyclist riding at a speed of `14sqrt3 ms^(-1)` takes a turn around a circular road of radius `20sqrt3m`. What is the inclination to the vertical ?
View SolutionOne end of a string of length 1.5 m is tied to a stone of mass 0.4 kg and the other end to a small pivot on a smooth vertical board. What is the minimum speed of the stone required at its lower most point so that the string does not slack at any point in its motion along the vertical circle ?
View SolutionThe centripetal force of 45 N required to revolve a stone of mass 100 g along a circular path of radius 50 cm. Find the constant speed of the stone.
View SolutionA gramophone record is rotating at 90 r.p.m. and a coin of mass 20 g is placed at a distance of 10 cm from its centre. What is the centrifugal force acting on the coin ?
View SolutionA stone of mass 0.25 kg tied to the end of a string is whirled round in a circle of radius 1.5 m with a speed of `40 rev.//min` in a horizontal plane. What is the tension in the string ? What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N ?
View SolutionA body of heavy mass is suspended by a string of length 50 cm. the body revolves along a horizontal circle of radius 25 cm, keeping the string always taut. Find the angular speed of the body.
View SolutionTwo skaters of equal mass on a circular rink go around the rink in the same time. One skater is twice as far from the centre of the rink as the other. Compare the speed of the skaters.
View SolutionTwo particles of equal masses are revolving in circular paths of radii `r_1` and `r_2` respectively with the same time period .The ratio fo the centripetal force is :
View SolutionTwo particles of equal masses are revolving in circular paths of radii `r_1` and `r_2` respectively with the same time period .The ratio fo the centripetal force is :
View SolutionThe driver of a three-wheeler moving with a speed of `36 km//h` sees a child standing in the middle of the road and brings his vehicle to rest in 4.0 s just in time to save the child. What is the average retarding force on the vehicle ? The mass of the three-wheeler is 400 kg and the mass of the driver is 65 kg.
View SolutionA car of mass 1200 kg can take a turn on a circular level road of radius of 150 m with a maximum speed of `15ms^(-1)` without skidding. Find the force of friction and the coefficient of friction between the tyres of the car and road.
View SolutionA cyclist speeding at `18 km h^-1` on a level road makes a sharp circular turn of radius 3 m without reducing the speed. The coefficient of static friction between the tyres and the road is 0.1 will the cyclist slip while taking the turn?
View SolutionCalculate the maximum speed with which a car cen be driven safely along a curved road of radius 30 m and banked at `30^@` with the horizontal. Given, `g=9.8 ms^(-2)`.
View SolutionThe radius of curvature of a railway line at a place is 40000m.the train is running at `20 m s^-1` .the distance between the two rails is 1.5 m.Find the elevation of the outer rail over the inner one so that the train may be able to run safely.
View SolutionThe vertical section of a road over a bridge in the direction of its length in the form of an arc of a circle of radius 4.4 m. Find the greatest velocity at which a vehicle can cross the bridge without losing with the road at the highest point, if the centre of gravity of the vehicle is 0.5 m from the ground. Given, `g=9.8 ms^(-2)`.
View SolutionA body weighing 0.4 kf is whirled in a vertical circle making 2 revlutions per second. If the radius of the circle is 1.2 m, find the tension in the string, when body is at the bottom of the circle.
View SolutionA body weighing 0.4 kf is whirled in a vertical circle making 2 revlutions per second. If the radius of the circle is 1.2 m, find the tension in the string, when body is at the top of the circle.
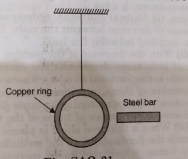
View SolutionA copper ring is suspended in a vertical plane by a thread. A steel bar is passed through the ring in a horizontal direction and then a magnet is similarly passed through. Will the motion of the bar and the magnet affect the position of the ring?
View SolutionA car of mass M moves with a constant speed `v` over a horizontal flat surface. What force is exerted by the car on the bridge in the case, as it passes the middle point of the bridge ?
View SolutionA car of mass M moves with a constant speed `v` over a convex bridge. What force is exerted by the car on the bridge in the case, as it passes the middle point of the bridge ? Take radius of curvature of the bridge in this case as r.
View SolutionA car of mass M moves with a constant speed `v` over a concave bridge. What force is exerted by the car on the bridge in the case, as it passes the middle point of the bridge ? Take radius of curvature of the bridge in this case as r.
View SolutionTwo blocks of mass `m_1=10 kg` and `m_2=5 kg` connected to each other by a massless inextensible string of length 0.3m are placed along a diameter of a turn table. The coefficient of friction between the table and`m_1` is 0.5 while there is no friction between `m_2` and the table. The table is rotating with an angular velocity of `10rad s^(-1)` about a vertical axis passing through its centre O. The masses are placed along the diameter of the table on either side of the centre O, such that `m_1` is at a distance of 0.124m from O. The masses are observed to be at rest with respect to an observer on the turn table. Calculate the frictional force on `m_1`.
View SolutionWhat provides the centripetal force to a satellite revolving around the earth?
View SolutionWhat provides the centripetal force to a satellite revolving around the earth?
View SolutionWhy are electrons revolving around the nucleus?
View SolutionFor uniform circular motion,does the direction of the centripetal force depend on th sense of rotation clockwise or anti-clockwise)?
View SolutionCan centripetal force produce rotation?
View SolutionWhat provides the centripetal force to a caar taking turn on a level road?
View SolutionIf, in Exercise 5.21, the speed of the stone is increased beyond the maximum permissible value, and the string breaks suddenly, which of the following correctly describes the trajectory of the stone after the string breaks :- the stone flies off tangentially from the instant the string breaks.
View SolutionWhat will be the maximum velocity with which a vehicle can negotiate a turn of radius `r` safely, when the coefficient of friction between the tyres and the road is `mu`.
View SolutionMoon is continuously revolving round the earth without falling towards it. Justify, why it does so ?
View SolutionWhat happens to a stone tied to the end of a string and whirled in a circle if the string suddenly breaks?
View SolutionOne often comes across the following kind of statement concerning circular motion : 'A particle moving uniformly along a circle experience a force directed towards the centre (centripetal force) and an equal and opposite force directed away from the centre (centrifugal force). The two forces together keep the particel in equilibrium'. Explain, what is wrong with this statement.
View SolutionWhy a person sitting inside a vehicle is thrown outwards, when vechile rounds a curve suddenly?
View SolutionWhy a person sitting inside a vehicle is thrown outwards, when vechile rounds a curve suddenly?
View SolutionWhy does a child in a merry - go - round press the side of his seat rdially outward?
View SolutionA car is taking a sudden turn to the left. A passenger in the front seat finds himself sliding towards the dorr. Explain, indicating the forces acting on the passenger and on the car at this instant.
View SolutionThe outer rail of a curved railway track is generally raised over the inner. Why ?
View SolutionWhy does a cyclist lean to one side while going along a curve? In which direction does he lean?
View SolutionWhy does a cyclist lean to one side while going along a curve? In which direction does he lean?
View SolutionA bucket containing water is rotated in a vertical circle. Explain why does not water fall down.
View SolutionExplain, why the pilot of the aeroplane does not fall down, while looping the loop ?
View SolutionWrite an expression for the centripetal force.
View SolutionExplain centipetal force and centrifugal force. Why centrifugal force is called a pseudo force ?
View SolutionDefine centrifugal force.
View SolutionExplain the need for banking of tracks.
View SolutionCalculate the maximum speed with which a vehicle can travel on a level circular road without skidding.
View SolutionDerive an expression for the angle of bending of a cyclist on a curved track.
View SolutionWhat is banking of a road ,why is it done?Find an expression for angle of banking.
View SolutionDerive an expression for the angle of bending of a cyclist on a curved track.
View SolutionWhat do you mean by banking of road?
View SolutionDefine centripetal and centrifugal forces. Explain with the help of neat diagram, how banking provides the centripetal force necessary for a car to go in circular track. (ignore friction between types and road).
View SolutionDefine angle of banking. Draw a neat labelled diagram showing different forces and their components acting on a vehicle moving on a banked road.
View SolutionWrite an expression for maximum velocity of a vehicle on an unbanked circular road.
View SolutionWhat will be the maximum velocity with which a vehicle can negotiate a turn of radius `r` safely, when the coefficient of friction between the tyres and the road is `mu`.
View SolutionWhy does a cyclist lean to one side while going along a curve? In which direction does he lean?
View SolutionDerive an expression for the angle of bending of a cyclist on a curved track.
View SolutionExplain why a centrifugal force is not a reaction of centripetal force.
View SolutionWhat provides the centripetal force to a satellite revolving around the earth?
View SolutionDerive an expression for the angle of bending of a cyclist on a curved track.
View SolutionDefine centripetal and centrifugal forces. Explain with the help of neat diagram, how banking provides the centripetal force necessary for a car to go in circular track. (ignore friction between types and road).
View SolutionWhat do you mean by banking of road?
View SolutionA stone of mass 50 g tied to one end of the string is rotated in a horizontal circle of radius 1 m with a speed of `5ms^(-1)`. Calculate the centripetal force.
View SolutionAn object of mass 0.4 kg is whirled in a horizontal circle of radius 2m. If ir performs 60 revolutions `mi n^(-1)`, calculate the centripetal force acting on it.
View SolutionFind the force required to revolve a body of mass 0.2 kg along a circular path of radius 2.5 m, so as to make 100 revolutions in one minute.
View SolutionA stone of mass 50 g tied to one end of the string is rotated in a horizontal circle of radius 1 m with a speed of `5ms^(-1)`. Calculate the centripetal force.
View SolutionA ball of mass 0.1 kg is revolved in a horizontal circular groove of radius 25 cm having vertical side walls. Find the contact force on the ball due to the wall of the groove, if it completes one round of the groove in 0.2s.
View SolutionA car of mass 800 kg is moving with a maximum speed of `10ms^(-1)` along a circular level track of 50 m radius without skidding. Find the force of friction and the coefficient of friction between the tyres and the road. Take, `g=10ms^(-2)`.
View SolutionFind the maximum speed at which a car can turn round a curve of 30 m radius on a level road, if the coefficient of friction between the tyres and the road is 0.4.
View SolutionThe radius of curvature of a railway line at a place is 40000m.the train is running at `20 m s^-1` .the distance between the two rails is 1.5 m.Find the elevation of the outer rail over the inner one so that the train may be able to run safely.
View SolutionFind the angle of banking of a curved railway track of radius 600 m, if the maximum safely speed limit of `54 km h^(-1)`. If the distance between the rails is 1.6 m, find the elevation of the outer track above the inner track. Given that `g=9.8 ms^(-2)`.
View SolutionA massless spring has a length 0.5 m and force constant `100 Nm^(-1)`. Its one end of is fixed and the other end is connected to a block of mass 0.5 kg. The system lies on a horizontal table and the block is made to rotate with an angular speed of `2 rad s^(-1)`. Find the elongation of the spring.
View SolutionA load is weighed on a spring balance in the carriage of a train,, which is moving along a curve of radius 400 m at a speed of `72 km h^(-1)`. If the weight of the load is 5 kgf, what will the reading of spring balance be ?
View SolutionA sphere of mass 0.1 kg is attached to an inextensible string of length 1.3 m, whose upper ens id fixed to the ceiling. The sphere is made to describe a horizontal circle of radius 0.5 m. Calculate the time period of one revolution..
View SolutionA sphere of mass 0.1 kg is attached to an inextensible string of length 1.3 m, whose upper ens id fixed to the ceiling. The sphere is made to describe a horizontal circle of radius 0.5 m. Calculate the tension in the string.
View SolutionA circular race track of radius 300 m is banked at an angle of `15^@`. If the coefficient of friction between the wheels of a race car and the road is 0.2, what is the optium speed of the race car to avoid wear and tear on its tyres.
View SolutionA circular race track of radius 300 m is banked at an angle of `15^@`. If the coefficient of friction between the wheels of a race car and the road is 0.2, what is the maximum permissible speed to avoid slipping ?
View SolutionThe centre of gravity of a loaded taxi is 1.5 m above the ground and the distance between its wheels is 2m. What is the maximum speed with which it can fo round an unbanked curve of radius 100 m without being turned upside down. What minimum value would the coefficient of friction is needed at this speed ?
View SolutionA ball rolls down an inclined plane from a height and then goes round a vertical circle of radius 1m. What should be the height of the inclined plane, so that the ball is able to go round thee circle without leaving the track ?
View Solution
Exercise questions and complete solutions for chapter Dynamics of Circular Motion of MODERN PUBLICATION of Class 11