Complete Solutions to EXERCISE of chapter MOTION IN A PLANE of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOTION IN A PLANE |
| NCERT | EXERCISE |
| Number Of Questions | 32 |
| Academic Year | 2023-24 |
State, for each of the following physical quantities, if it is a scalar or a vector. Volume, mass speed acceleration, density, number of moles, velocity, angular frequency, displacement, angular velocity.
View SolutionPick out the two scalar quantities in the following list: force, angular momentum, work, current, linear momentum, electric field, average force, angular momentum, work, current , linear momentum, electric field, average velocity, magnetic moment, relative velocity.
View SolutionPick out the only vector quantity in the following list:
Temperature, pressure, impulse, time, power , total path length, energy, gravitational potential , coefficient of friction, charge.
View SolutionState with reasons, whether the following algebraic operations with scalar and vector physical quantities are meaningful:
(i) Adding any two scalars (ii) Adding a scalar to a vector of the same dimensions
(iii) Multiplying any vector by any scalar (iv) Multiplying any two scalars
(v) Adding any two vectors
View SolutionRead each statement below carefully and state with reasons, with it is true or false :
(a) The magnitude of vector is always a scalar.
(b) Each component of a vector is always a scalar.
(c) The total path length is always equal to the magnitude of the displacement vector of a particle.
(d) The average speed of a particle (defined as total path length divided by the time taken to cover the path) is greater or equal to the magnitude of average velocity of the particle over the same interval of time.
(e) three vectors not lying in a plane can never add up to give a null vector.
View SolutionEstablish the following inequalities geometrically or otherwise ,
(a) ` | vec A + vec B| lt= | vec A | + |vec B|` ,
(b) ` | vec A + vec B| gt= ||vec A|- | vec B||`
(C )` | vec A- vec B| lt= | vec A| + |vec B|`
(d) `| vec A- vec B| gt= || vec A| - | vec B||`
When does the equality sign above apply ?
View SolutionGiven ` vec A + vec B+ vec C + vec D =0`, which of the following statements are correct ?
(a) ` vec A, vec B ,vec C ` abd ` vec D` each must be a null vector.
(b) The magnitude of ` ( vec A + vec C)` equals the magnitude of ` ( vec B + vec D)`.
(c) The magnitude of ` vec A` can never be greater than the sum of the magnitude of ` vec B , vec C ` and `vec D`.
(d) ` vec B + vec C` must lie in the plane of ` vec A + vec D`. if `vec A` and `vec D` are not collinear and in the line of ` vec A` and `vec D`, if they are collinear.
View SolutionThree girls skating on a circular ice ground of radius ` 200 m` start from a point (P) on the edge of the ground and reach a point ` Q` diametrically opposite to (P) following different paths as shown in Fig. What is the magnitude of the displacement vector for each ? which girl's displacement is equal to the actual length of path skate ?
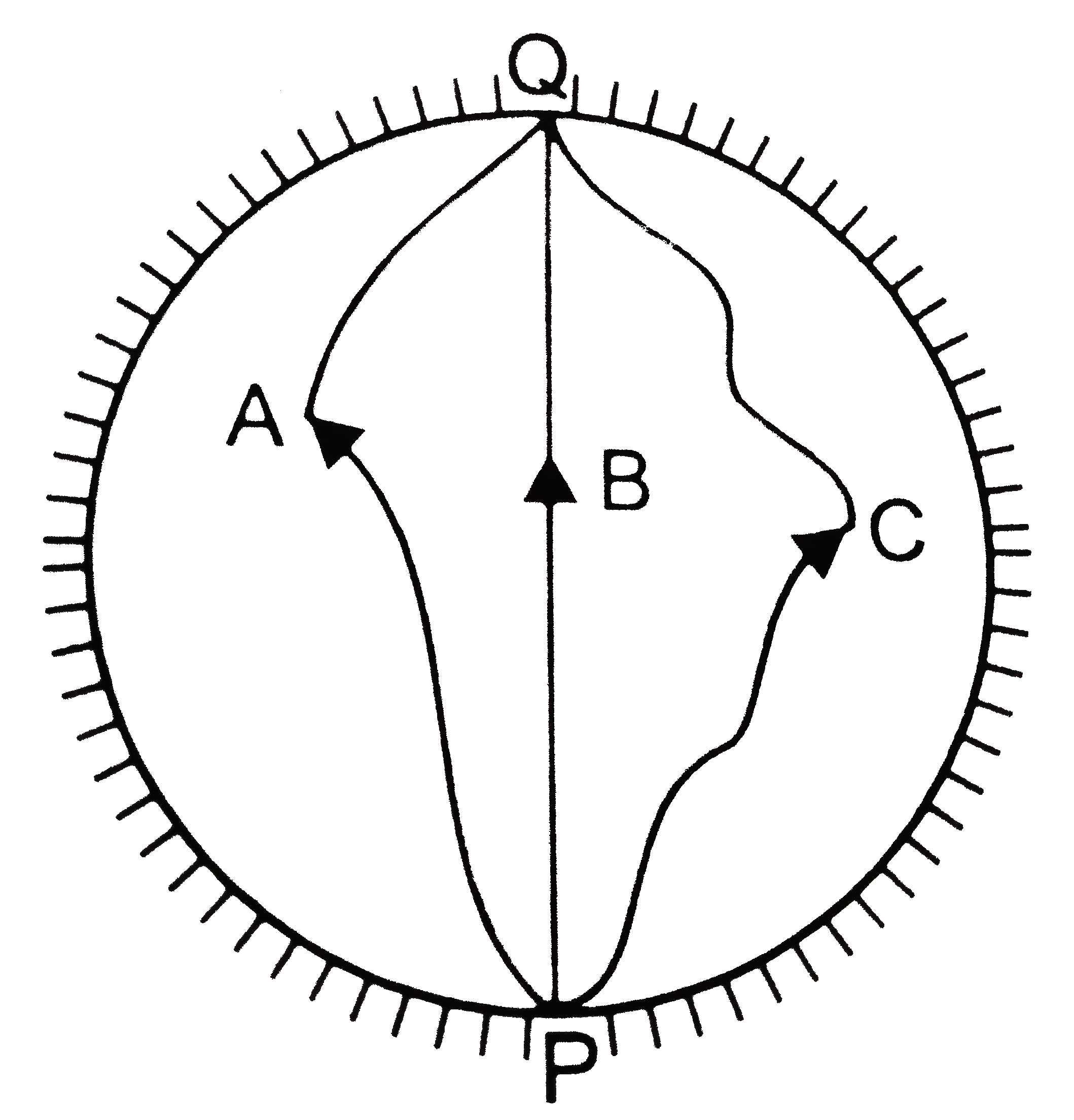 .
.
View SolutionA cyclist starts from the center O of a circular park of radius 1km, reaches the edge P of the park, then cycles along the PQ circumference and returns to the center along OQ as shown in fig. If the round trip taken ten minute, the (a) net displacement, (b) average velocity and (c) average speed of the cyclists (in kilometer per hour) is
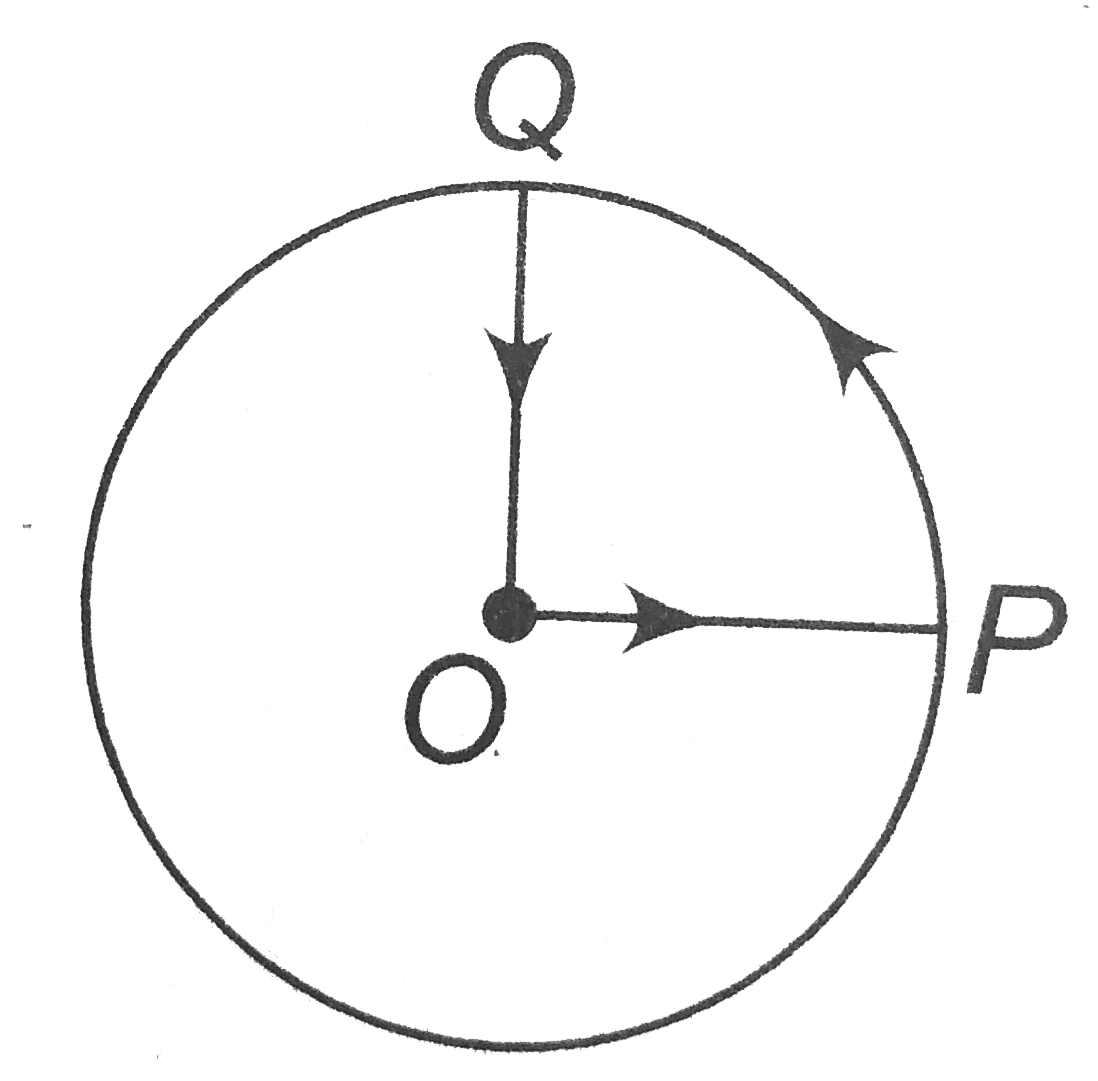
View SolutionOn an open ground, a motorist follows a track that turns to his left by an angle of `60^(@)` after every `500 m`. Starting from a given turn, The path followed by the motorist is a regular hexagon with side `500 m`, as shown in the given figure specify the displacement of the motorist
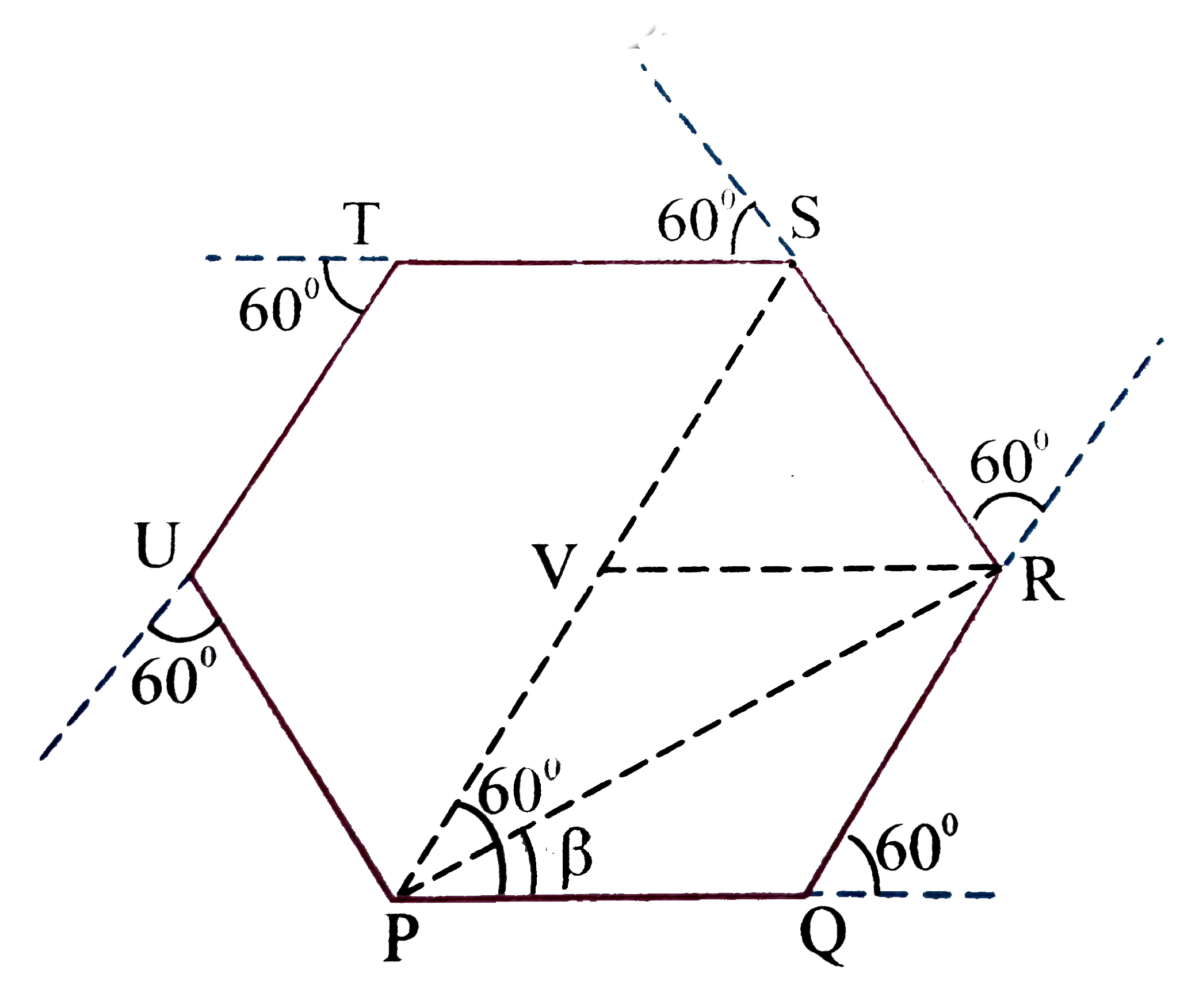
at the third, sixth and eighth turn. Compare the magnitude of the displacement with the total path length covered by the motorist in each case.
View SolutionA passenger arriving in a new town wishes to go from the station to a hotel located ` 10 km` away on a straight road from the station. A dishonest cabman takes him along a circuitous path ` 23 km` long and reaches the hotel in ` 28` minutes . What is (a) the average speed of the taxi, (b) the magnitude of average velocity ? Are the two equal ?
View SolutionRain is falling vertically with a speed of ` 30 ms^(-1)` . A woman rides a bicycle with a speed of ` 10 ms^(-1)` in the North to South direction. What is the direction in which she should hold her umbrella ?
View SolutionA man can swim with a speed of `4kmh^(-1)` in still water. He crosses a river 1km wide that flows steadly at `3kmh^(-1)`. If he makes his strokes normal to the river current, how far down the river does he go when he reaches the other bank?
View SolutionIn a harbour, wind is blowing at the speed of ` 72 km //h` and the flag on the mast of a boat anchored in the harbour flutters along the N-E` direction`. If the boat starts moving at a speed of ` 51 km// h` to the North, what is the direction of flag on the mast of the bat ?
View SolutionThe ceiling of a long hall is ` 25 m` high. What is the maximum horizontal distance if a ball thrown with a speed of ` 40 ms^(-1)` can go without hitting the ceiling of the hall ?
View SolutionA cricketer can throw a ball to a maximum horizontal distance of 100m. With the same speed how much high above the ground can the cricketer throw the same ball?
View SolutionA stone tied to end of a string ` 80 cm` long is whirled in a horizontal plane with a constant speed. If the stone makes ` 14` revolutions in ` 25` seconds, what is the magnitude and direction of acceleration of the stone ?
View SolutionAn aircraft executes a horizontal loop of radius 1 km with a steady speed of `900 km h^(-1)`. Compare its centripetal acceleration with the acceleration due to gravity.
View SolutionRead each statement below carefully and state, with reasons, if it is true or false :
(a) The net acceleration of a particle in circular motion is always along the radius of the circle towards the center.
(b) The velocity vector of a particle at a point is always along the tangent to the path of the particle at that point.
(c) The acceleration vector of a particle in uniform circular motion averaged over one cycle is a null vector.
View SolutionThe position of a particle is given by ` vec r= 3.0 t hat i - 2.0 t^2 hat j + 4.0 hat k m`, where (t) in seconds and the coefficients have the proper units for `vec r` to be in metres. (a) Find the ` vec v` and ` vec a` of the particle ? (b) What is the magnitude and direction of velocity of the particle at ` t= 2 s`?
View SolutionA particle starts from the origin at ` t=0` with a velocity of ` 10.0 hat j m//s` and moves in the x-y plane with a constant acceleration of ` ( 8.0 hat i + 2. 0 hat j ) ms^(-2)` . (a) At what time is the x-coordinate of the particle ` 16 m` ? What is the y-coordinate of the particle at that time ? (b) What is the speed of the particle at that time ?
View Solution`hat(i)` and `hat(j)` are unit vectors along x-and y-axis respectively. What is the magnitude and the direction of the vectors `hat(i)+hat(j)` and `hat(i)-hat(j)`? What are the components of a vector `vec(A)=2hat(i)+3hat(j)` along the direction `hat(i)+hat(j)`and `hat(i)-hat(j)`?
View SolutionFor any arbitrary motion in space, which of the following relations are true?
(a) `v_("average") = (1//2)(v(t_(1)) + v(t_(2)))`
(b) `v_("average") = [r(t_(2))-r(t_(1)]//(t_(2)-t_(1))`
(c) `v(t) = v(0) + at`
(d) `a_("average") = [v(t_(2))-v(t_(1))]//(t_(2)-t_(1))`
The average stands for average of the quantity over time interval `t_(1)` to `t_(2)`
View SolutionRead each statement below carefully and state with reason and examples, if it is true or false.
A scalar quantity is one that
(a) is conserved in a process
(b) can never take negative values
(c) must be dimensionless
(d) does not vary from one point to another in space
(e) has the same value for observers with different orientations of axes.
View SolutionAn aircraft is flying at a height of 3400 m above the ground. If the angle subtended at a ground observation point by the aircraft positions 10 s apart is `30^(@)` , what is the speed of the aircraft ?
View SolutionA vector has magnitude and direction (i) Does it have a location in the space ? (ii) Can it vary with time ? (iii) Will two equal vectors ` vec a` and `vec b` different locations in space necessarily have identical physical effects ? Give examples in support of your answer.
View SolutionA vector has both magnitude and direction. Does that mean anything that has magnitude and direction is necessarily a vector ? The rotation of a body can specified by the direction of the axis of rotation and the angle of rotation about the axis. Does the make any rotation a vector ?
View SolutionCan you associate vectors with (a) the length of a wire bent into a loop (b) a plane area (c) a sphere ? Explain.
View SolutionA bullet fired at an angle of `30^@` with the horizontal hits the ground 3.0 km away. By adjusting its angle of projection, can one hope to hit a target 5.0 km away? Assume the muzzle speed to be fixed and neglect air resistance.
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km `h^(-1)` passes diretly overhead an anti-aircraft gun. At what angle from the vertical should the gun he fired for the shell with muzzle speed 600 m `s^(-1)` to hit the plane. (Take g = 10 m `s^(-1)`)
View SolutionA cyclist is riding with a speed of `27 km h^(-1)`. As he approaches a circular turn on the road of radius 80 m, he applies brakes and reduces his speed at the constant rate `0.5 ms^(-1)`. What is the magnitude and direction of the net acceleration of the cyclist on the circular turn ?
View SolutionShow that for a projectile the angle between the velocity and the x-axis as function of time is given
by ` theta_(t) = tan ^(-1)( ( v_(0 y)-g t)/(v_(o x)))`
(b) Shows that the projection angle `theta_0` for a projectile launched from the origin is given by ` theta_(t) = tan ^(-1)( ( 4 h_m)/R)`
where the symbols have their usual meanings.
View Solution
EXERCISE questions and complete solutions for chapter MOTION IN A PLANE of NCERT PHYSICS(ENGLISH) of Class 11