Complete Solutions to EXERCISE of chapter SOLVED PAPER 2017-1 of Class 11 book with complete answers and questions

Given that the number of subsets of a set . A is 16. Find th number of elements in A.

If (x - 1, y + 3 ) = (2, x + 4 ) Find the values of x and y .

Convert `(7pi)/6` radians in degree measure ?

Find the multiplicative inverse of ` sqrt 5 + 3i`

Find 20th term of G.P. `5/2,5/4,5/8-----.`

Find 'n' if `'^(n)C_9=^nC_8`.

Find the slope of the line `x-y+3=0`

Write the negation of the statement " `sqrt(7)` is rational".

Evaluate`underset(x rarr 0) lim [((x+1)^5-1)/x]`.

A letter is chosen at random from the word "ASSASSINATION" . Find the probability that letter is vowel.

If X and Y are two sets such that `X ∪ Y` has 18 elements, X has 8 elements and Y has 15 elements , how many elements does `X ∩ Y` have?

If A={-1,1}, find `A xx A xx A`

Let ` f(x) = sqrt(x) and g(x) = x ` find (i) (f + g) x (ii) (fg) x

Let ` f(x) = sqrt(x) and g(x) = x ` find (i) (f + g) x (ii) (fg) x

The minute hand of a clock is 2.1cm long. How far does its tip move is 20 minute. `("use" pi=22/7)`

Find the general solutions of ` 2 cos ^(2) x - 3 sin x = 0 `

Evaluate `underset (x rarr 1)lim(x^15-1)/(x^10-1)`.

Find the median for the following data. 3,9,5,10,18,4,7,19,21.

Write the converse and contrapositive of 'If a parallelogram is a square,then it is a rhombus'.

On her vacations Veena visits cities A,B C and D in random order . What is the probability that she visits A before B ?

In the triangle ABC with vertices `A (2, 3) , B (4, -1) " and " C (1, 2)`, find the equation and length of altitude from the vertex A.

Find the distance between two parallel lines `3x+4y+5=0` and `6x+8y+2=0`

Solve `4x+3 lt 6x+7`.

Show that the points P(- 2 , 3, 5) , Q (1, 2, 3) and R(7, 0, -1) are collinear.

Express `1+isqrt3` in polar form

In a survey of 600 students in a school, 150 students were found to be taking tea and 225 taking coffee, 100 were taking both tea and coffee. Find how many students were taking neither tea nor coffee?

Define a signum function. Write range, also draw the graph of the function.

`tan4x=(4tan x(1-tan^(2)x))/(1-6tan^(2)x+tan^(4)x)`

If ` x + iy = sqrt((a + ib)/(c + id)) ` Prove that ` x^(2) + y^(2) = sqrt(( a^(2) + b^(2))/(c^(2) + d^(2)))`

Convert the complex number `-16/(1+isqrt(3))` into polar form.

Find `(a+b)^4 -(a-b)^4`.Hence evaluate `(sqrt(3)+sqrt(2))^4-(sqrt(3)-sqrt(2))^4`.

How many words, with or without meaning can be made from the letters of the word MONDAY, assuming that no letter is repeated, if.
(i) 4 leters are used at a time,
(ii) all letters are used at a time
(iii) all letters are used but first letter is a vowel ?

How many words, with or without meaning can be made from the letters of the word MONDAY, assuming that no letter is repeated, if.
(i) 4 leters are used at a time,
(ii) all letters are used at a time
(iii) all letters are used but first letter is a vowel ?

How many words, with or without meaning can be made from the letters of the word MONDAY, assuming that no letter is repeated, if.
(i) 4 leters are used at a time,
(ii) all letters are used at a time
(iii) all letters are used but first letter is a vowel ?

Find the foci and eccentricity of ellipse ` (x^(2))/(16) + (y^(2))/(9) = 1 `

How many terms of AP - 6 ` -11//2,-5 . . . ` are needed to give the sum - 25 ?

In an A.P if `m^(th)` term is n and `n^(th)` term is m, where `mnen,` find the `p^(th)` term .

Compute the derivative of sinx using first principal method ?

Verify by the method of contradiction that `sqrt(2)` is irrational .

A committee of two persons is selected from two men and two women.What is the probability that the committee will have (i) no men (ii)two men

A committee of two persons is selected from two men and two women.What is the probability that the committee will have (i) no men (ii)two men

If E and F are two events such that `P(E)=1/4, P(E)=1/2 and P(E and F)=1/8`. Find P (not E and not F).

Prove that `underset(xrarr0)lim(sinx/x=1)` ?

`1^(2)+2^(2)+3^(2)+............+n^(2)=(n(n+1)(2n+1))/6 forall n in N.`

Define a modulus function . Draw its graph. Also write down its domain and range.

A group consists of 7 boys and 5 girls . Find the number of ways in which a team of 5 members can be selected so as to have atleast one boy and girl.

State and prove Bionomial theorem for any positive integer n.

If p is the length of perpendicular from origin to the line whose intercepts on the axes are 'a' and 'b' then prove that `(1)/(p^(2)) = (1)/(a^(2)) + (1)/(b^(2))` .

`(cos 4x+cos3x+cos2x)/(sin 4x+sin3x+sin2x)=cot3x`

Solve the following system of inequalities graphivally `2x+y>+4,x+yle3,2x-3yle6`,

Find the mean deviation about median for the following data:

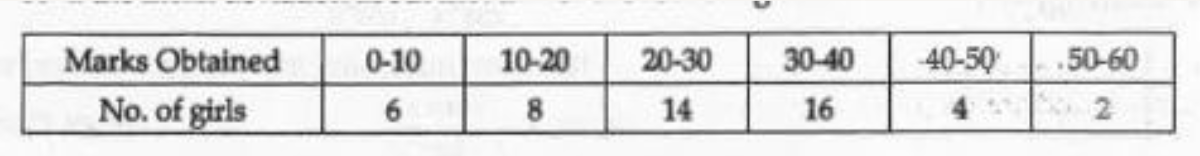
To `cos(A+B)=cosx.cosy - sin x sin "and hence find "cos75^(@)`

Find the sum to n terms of the series `1^(2)+(1^(2)+2^(2))+(1^(2)+2^(2)+3^(2))+.....`

Derive the equation of the ellipse in the form `(x^(2))/(a^(2))+(y^(2))/(b^(2))=1`.

(b) Find the derivative of `(x^(5) - cos x)/(sin x)` with respect to x.