Complete Solutions to EXERCISE of chapter SOLVED PAPER 2020-1 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | SOLVED PAPER 2020-1 |
| OSWAAL PUBLICATION | EXERCISE |
| Number Of Questions | 54 |
| Academic Year | 2023-24 |
Given that the number of subsets of a set . A is 16. Find th number of elements in A.
View SolutionIf A={7,8} and B={5,4,2}.Find `A xx B`
View SolutionFind the modulus and the argument of the complex number `-sqrt3 + i`
View SolutionWrite the first five terms of the sequence defined by ` a_n = ( n)/( n+1) ` where n `in ` N
View SolutionFind the slope of the line `x/3+y/2=1`
View SolutionWrite the negation of the statement `sqrt 2` is a complex number.
View SolutionIf `2/11`is the probability of an event 'A'.What is the probability of the event 'not A'?
View SolutionIf X and Y are two sets such that `X ∪ Y` has 18 elements, X has 8 elements and Y has 15 elements , how many elements does `X ∩ Y` have?
View SolutionIf A={1,2,3},B={3,4} and C={4,5,6},Find `A xx (BnnC)`
View SolutionThe range of the function `f(x) = sqrt(9-x^(2))`
View SolutionFind the radius of the circle in which a central angle of `60^(@)` intercepts an arc of length 37.4 cm (use `pi=22/7`)
View SolutionProve that `sin 2x=(2 tan x)/(1+tan^(2)x)`
View SolutionSolve each of the following equations.
1. Solve `x^(2) +x+1=0`
View SolutionThe marks obtained by a student of class XI in the first and second terminal examination are 62 and 48 respectively. Find the minimum marks he should get in the annual examination to have an average of at least 60 marks .
View SolutionFind the distance of the point (3,-5) from the line `3x -4y -26 =0`
View SolutionFind the equation of the line parallel to the line `3x -4y + 2=0` and passing through the point `( -2,3) `
View SolutionFind the co-ordinates of the point P which divides the line segment joining the points A ( 1, - 2 ,3 ) and B ( 3 , 4 - 5 ) internally in the ratio 2 : 3
View SolutionWrite the contrapositive and converse of the following statements.
If x is a prime number, then x is odd.
View SolutionWrite the mean of the given data : 6,7,10,12,13,4,8,12 ?
View SolutionA letter is chosen at random from the word "ASSASSINATION" . Find the probability that letter is vowel.
View SolutionA letter is chosen at random from the word 'ASSASSINATION' Find the probability that latter is a vowel (ii) a consonant
View SolutionIn a survey of 400 students in a school, 100 were listed as taking apple juice, 150 as taking orange juice and 75 were listed as taking both apple as well as orange juice. Find how many students were taking neither apple juice nor orange juice.
View SolutionLet `f(x)=x^2` and `g(x)=2x+1`be two real valued functions,Find (i)`(f+g)(x)`
View SolutionLet `f(x)=x^2` and `g(x)=2x+1`be two real valued functions,Find(ii) `(f-g)(x)`
View SolutionLet `f(x)=x^2" and "g(x)=2x+1` be two real values functions, find
`(fg)(x)`.
View SolutionExpress the complex number `(-1+isqrt3)` in polar form ?
View Solutionfind general solution `sin x + sin 3x + sin 5x =0`
View SolutionFind r, if `5""^(4)P_(r)=6""^(5)P_(r-1)`
View SolutionFind the middle term in the expansions of `(3 - x^3/6)^7`
View SolutionInsert five numbers between 8 and 26 such that the resulting sequence is in AP.
View SolutionThe sum of first three terms of a G.P. is `13/12` and their product is – 1. Find the common ratio and the terms.
View SolutionFind the centre and radius of the circle `x^(2)+y^(2)-4x-8y-45=0` ?
View SolutionDifferentiate of ` sin x` w.r.t. x from first principles
View SolutionVerify by the method of contradiction that ` sqrt7` is irrational number
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have (a) no man? (b) one man? (c) two men?
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have (a) no man? (b) one man? (c) two men?
View SolutionA committee of two persons is selected from two men and two women.What is the probability that the committee will have (c) two men?
View SolutionIf E and F are two events such that `P(E)=1/4, P(E)=1/2 and P(E and F)=1/8`. Find P (not E and not F).
View SolutionIf E and F are two events such that `P(E)=1/4, P(E)=1/2 and P(E and F)=1/8`. Find P (not E and not F).
View SolutionDefine a modulus function . Draw its graph. Also write down its domain and range.
View Solution`1^(2)+2^(2)+3^(2)+..........+n^(2)=(n(n+1)(2n+1))/(6)`
View SolutionProve that `cos^(2)+x+cos^(2) (x+pi/3)+cos^(2)(x-pi/3)=3/2` and hence find the values of `sin^(2)x +sin^(2) (x+pi/3)+sin^(2) (x-pi/3)`
View SolutionSolve the following system of inequalities graphically `x+2yle8,2x+yle8,xge0,yge0`.
View SolutionA group consists of 5 girls and 7 boys.In how many ways can a team of 5 members be selected if the team has atleast one boy and one girl.
View SolutionA group consists of 4 girls and 7 boys. In how many ways can a team of 5 members be selected, if the team has.
At least three girls?
View SolutionState and prove Bionomial theorem for any positive integer n.
View SolutionDerive the equation of a plane in normal form both in the vector and Cartesian form .
View SolutionProve that `lim_(thetararr0)(sin theta)/(theta)=1`.
View SolutionDerive the formula to find the co-ordinates of a point which divide the line joining the points `A(x_1,y_1,z_1)` and `B(x_2,y_2,z_2)`internally in the ratio `m:n`.
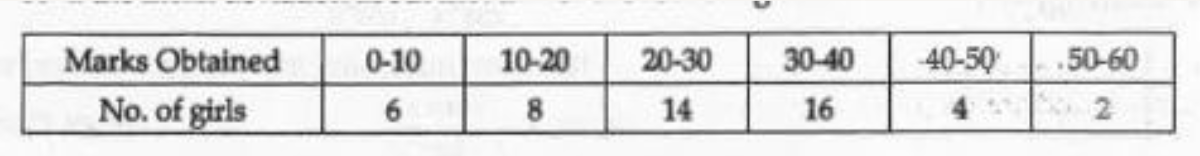
View SolutionFind the mean deviation about median for the following data:
View Solution(a)Derive geometrically that `cos(x+y)=cos x cos y-sin x sin y`.Hence deduce the valueof `cos 75^@`
View Solution(b) Find the sum to n terms of the series `1 xx 2 xx 3 + 2 xx 3 xx 4 + 3 xx 4 xx 5 + ..`.
View SolutionDerive the equation of the ellipse in the form `(x^(2))/(a^(2))+(y^(2))/(b^(2))=1`.
View Solution(b) Find the derivative of `(x^(5) - cos x)/(sin x)` with respect to x.
View Solution
EXERCISE questions and complete solutions for chapter SOLVED PAPER 2020-1 of OSWAAL PUBLICATION of Class 11