Complete Solutions to Exercies 7 of chapter RADIO ACTIVITY of Class 11 book with complete answers and questions
| Class 11 | CHEMISTRY |
| Chapter | RADIO ACTIVITY |
| P BAHADUR | Exercies 7 |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
The stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
Loss in mass during the change.
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
`underset((E.S.))( ._(8)^(19))O rarr underset((G.S.))( ._(8)^(19))O + 1.06xx10^(8) "kcal"//mol`. The two oxygen atoms differ by a mass per mol is:
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
`._(90)^(228)Th overset(-alpha)(rarr) A`,If `Th` belongs to `III gp`of periodic table, then `'A'` belongs to:
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
An element having `n//p` ratio greater than 1 will show:
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
An element having `n//p` ratio lesser than 1 and lying below the belt of stabiiliy shows:
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
Durting `K-` electron capture, the emission is always in the region of:
View SolutionThe stabiliy of nuclies has been explained in terms of binding energy. Higher is binding energy, more is stabliliy to nucleus. The binding energy is written as `B.E = 931.478 xx Delta m' MeV`m wehre `Delta m'` is mass decayed in amu. If `B.E.//"nucleon"` lies below the belt of stablilty, the nucleus undergoes `alpha-` emission in order to lower down the energy level of nucleus but its `n//p` ratio increases. To lower down the energy level of nucleus but is `n//p` ratio increases. To lower down level of nucleus loses `beta-` particles and if stability is not gained, `gamma-` emission is noticed. A radioactive element on losing on `alpha-` particles shows a loss in its mass number by `4` units and atomic number by 2 units whereas `beta-` emission leads to a gain in at.no by one units and mass no. remains same.
Total number of `alpha-` and `beta-` particles emitted during radioactive emssion of `._(92)^(235)U` to attain stabilty is:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
The percentage of atoms decayed in average life of a radioactive element is:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
The completion of radioactive emission from a species takes place in:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
Which one cause more damage to humain tissue if exposed to radioacitve emission out of `alpha` or `beta-` paricles?
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
Report the wrong realation:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
The number of `beta-` particles emitted during the change, `._(a)^(c)X rarr ._(d)^(b) Y` is:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
The half-life of `3g` of sample of a radioactive species `.^(14)CO_(2)` is `2` minute. If `10g` sample of `.^(14)CO_(2)` is taken, the half-life would be:
View SolutionRadioactive disintergation always follow `I` order kinetics and is independent of all external factors and is represented by the relation `N = N_(0) e^(-lambda t)` where `lambda` is decay constant and `N` atoms are left at time `t`. The radioactive nature of element is expressed in terms of average life numerically equal to decay constant `(1//lambda)` however all the radioactive do not lose their radioactive nature in thier average life. The radioactive emission involves `alpha, beta` particles as well as `gamma-` rays.The penertrating power order is `alpha lt beta lt gamma`. The emissions can perntrate even thick steel walls but are however unable to penttrate `Pb` blocks. The `S` unit fo rate of decay is `dps`.
The mass of `.^(14)C` with `t_(1//2) = 5730` year having activity equal to `1` curies si:
View SolutionRutherford studied the first nuclear reaction `[._(7)^(14)N(alpha,p) ._(8)^(17)O]` which take place with a change in energy equivalent to `1.193 MeV`. Later on various types of nuclear reactions such as artifical radioactivity, artifical transmutatoon , nuclear fission, nuclear fussion, spallation reactions etc. were studied.
The reaction `._(97)^(14)N + ._(2)^(4)He + ._(8)^(17)O + ._(1)^(1)H` may be carried out by bombaring `N` atoms with `alpha-` particles of energy:
View SolutionRutherford studied the first nuclear reaction `[._(7)^(14)N(alpha,p) ._(8)^(17)O]` which take place with a change in energy equivalent to `1.193 MeV`. Later on various types of nuclear reactions such as artifical radioactivity, artifical transmutatoon , nuclear fission, nuclear fussion, spallation reactions etc. were studied.
The reaction, `._(33)^(75) As + ._(1)^(2)H rarr ._(25)^(56)Mn + 9 ._(1)^(1)H + 12 ._(0)^(1)n` is called nuclear reaction or:
View SolutionRutherford studied the first nuclear reaction `[._(7)^(14)N(alpha,p) ._(8)^(17)O]` which take place with a change in energy equivalent to `1.193 MeV`. Later on various types of nuclear reactions such as artifical radioactivity, artifical transmutatoon , nuclear fission, nuclear fussion, spallation reactions etc. were studied.
Nuclear fusion reactions is not:
View SolutionRutherford studied the first nuclear reaction `[._(7)^(14)N(alpha,p) ._(8)^(17)O]` which take place with a change in energy equivalent to `1.193 MeV`. Later on various types of nuclear reactions such as artifical radioactivity, artifical transmutatoon , nuclear fission, nuclear fussion, spallation reactions etc. were studied.
Which one is correct?
View SolutionCarbon 14 is used to determine the age of organic material. The procerdure is based on the formation of `.^(14)C` by neutron capture in the upper atmosphere.
`._(7)^(14)N + ._(0)^(1)n rarr ._(6)^(14)C + ._(1)n^(1)`
`.^(14)C` is abosorbed by living organisms during phostosythesis. The `.^(14)C` content is constant in living organisms once the plant or animal dies, the uptake of carbon dioxide by it ceases and the level of `.^(14)C` in the dead being, falls due to the decay which `.^(14)C` undergoes.
`._(6)^(14)C rarr ._(7)^(14)C + beta^(-)`
The half-life period of `.^(14)C` is 5770 years. The decay constant `(lambda)` can be calculated by using the following formula `lambda = (0.693)/(t_(1//2))`
The comparison fo the `beta^(-)` activity fo the dead matter with that of the carbon still in circulation enables measurement of the period of the isolation the materail form the living cycle. The method however, ceases to be accurate ever periods longer than `30,000` years. The proportaion of `.^(14)C` to `.^(12)C` living matter is `1:10^(12)`.
Which fo the following option is correct?
View SolutionCarbon 14 is used to determine the age of organic material. The procerdure is based on the formation of `.^(14)C` by neutron capture in the upper atmosphere.
`._(7)^(14)N + ._(0)^(1)n rarr ._(6)^(14)C + ._(1)n^(1)`
`.^(14)C` is abosorbed by living organisms during phostosythesis. The `.^(14)C` content is constant in living organisms once the plant or animal dies, the uptake of carbon dioxide by it ceases and the level of `.^(14)C` in the dead being, falls due to the decay which `.^(14)C` undergoes.
`._(6)^(14)C rarr ._(7)^(14)C + beta^(-)`
The half-life period of `.^(14)C` is 5770 years. The decay constant `(lambda)` can be calculated by using the following formula `lambda = (0.693)/(t_(1//2))`
The comparison fo the `beta^(-)` activity fo the dead matter with that of the carbon still in circulation enables measurement of the period of the isolation the materail form the living cycle. The method however, ceases to be accurate ever periods longer than `30,000` years. The proportaion of `.^(14)C` to `.^(12)C` living matter is `1:10^(12)`.
What should be the age of fossil for meainingful determination of its age?
View SolutionCarbon 14 is used to determine the age of organic material. The procerdure is based on the formation of `.^(14)C` by neutron capture in the upper atmosphere.
`._(7)^(14)N + ._(0)^(1)n rarr ._(6)^(14)C + ._(1)n^(1)`
`.^(14)C` is abosorbed by living organisms during phostosythesis. The `.^(14)C` content is constant in living organisms once the plant or animal dies, the uptake of carbon dioxide by it ceases and the level of `.^(14)C` in the dead being, falls due to the decay which `.^(14)C` undergoes.
`._(6)^(14)C rarr ._(7)^(14)C + beta^(-)`
The half-life period of `.^(14)C` is 5770 years. The decay constant `(lambda)` can be calculated by using the following formula `lambda = (0.693)/(t_(1//2))`
The comparison fo the `beta^(-)` activity fo the dead matter with that of the carbon still in circulation enables measurement of the period of the isolation the materail form the living cycle. The method however, ceases to be accurate ever periods longer than `30,000` years. The proportaion of `.^(14)C` to `.^(12)C` living matter is `1:10^(12)`.
A nulcear explosion has taken place leading to increases in conventration of `^(14)C` in nearly areas. `^(14)C` concentration is `C_(1)` in nearby areas and `C_(2)` in areas far away. If the age of the fossil is detemined to be `T_(1)` and `T_(2)` at the places respectively, then:
View SolutionSome times a reacant undergoes chemical/radioactive changes following two or more different paths to yield two or more different produces respectively. Such reactions are called parallel path reactions. If `K_(1)` and `K_(2)` are rate constans for the reaction of `A` follwing two parallel paths, then
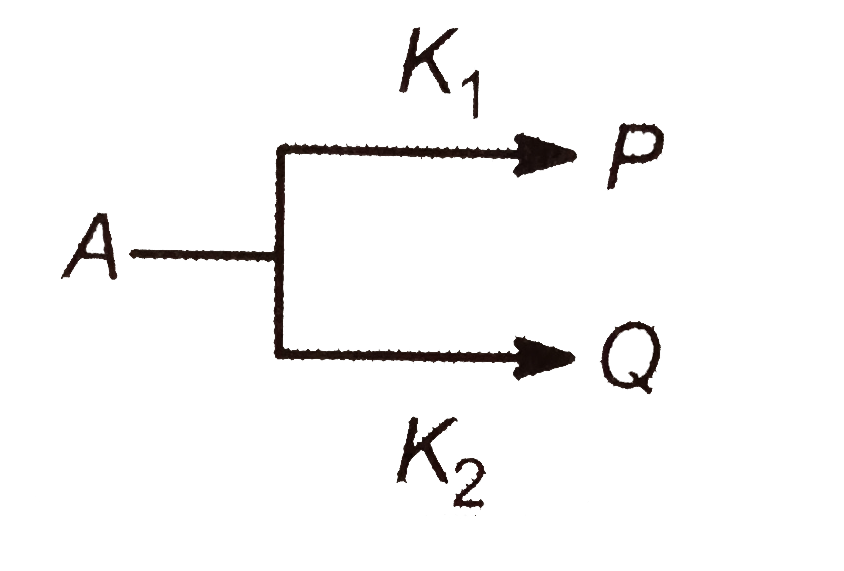
Then `K_(av) = K_(1) + K_(2)`
For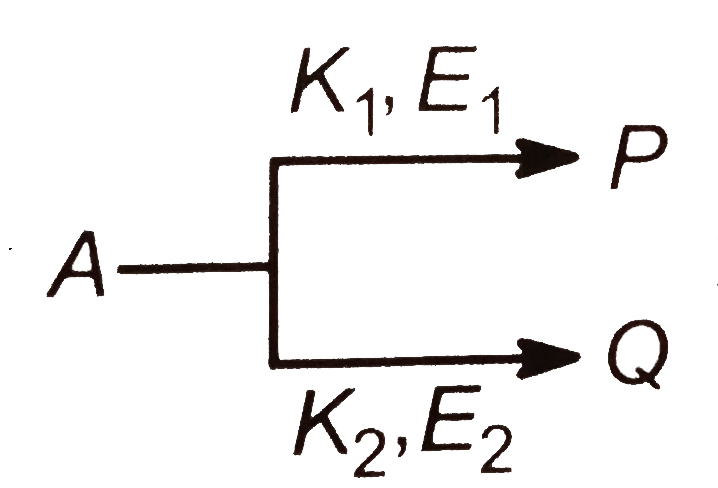 if `E_(1)` and `E_(2)` are energy fo activations, then
if `E_(1)` and `E_(2)` are energy fo activations, then
View SolutionSome times a reacant undergoes chemical/radioactive changes following two or more different paths to yield two or more different produces respectively. Such reactions are called parallel path reactions. If `K_(1)` and `K_(2)` are rate constans for the reaction of `A` follwing two parallel paths, then
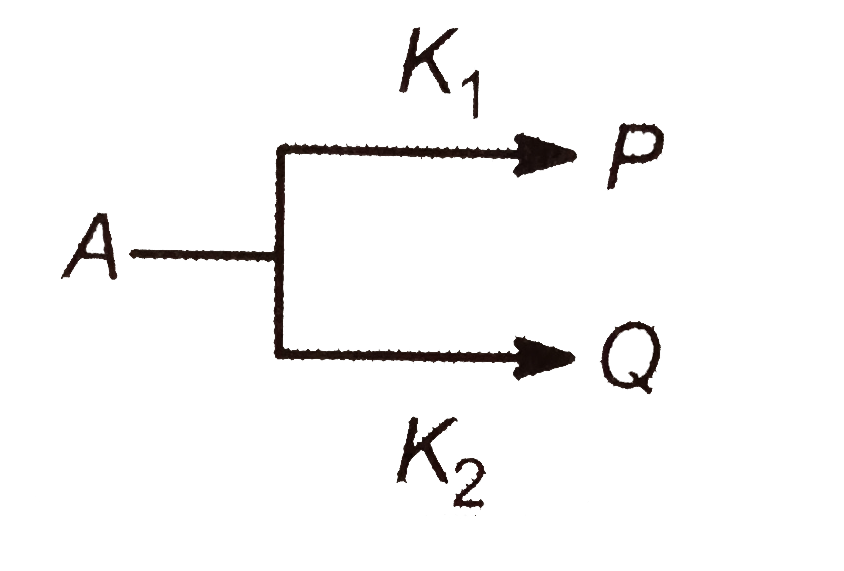
Then `K_(av) = K_(1) + K_(2)`
If average life of `A` for `p` is `T_(1)` and for `Q` is `T_(2)` then:
View SolutionSome times a reacant undergoes chemical/radioactive changes following two or more different paths to yield two or more different produces respectively. Such reactions are called parallel path reactions. If `K_(1)` and `K_(2)` are rate constans for the reaction of `A` follwing two parallel paths, then
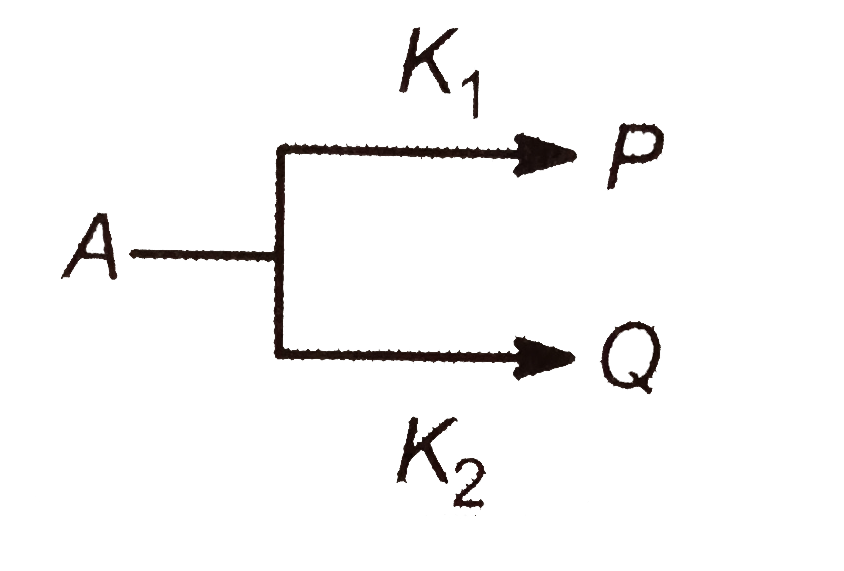
Then `K_(av) = K_(1) + K_(2)`
Which one of the following is correct for the above reaction:
View Solution
Exercies 7 questions and complete solutions for chapter RADIO ACTIVITY of P BAHADUR CHEMISTRY (HINGLISH) of Class 11