Complete Solutions to Exercise of chapter Mathematical tools of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | Mathematical tools |
| SL ARORA | Exercise |
| Number Of Questions | 20 |
| Academic Year | 2023-24 |
Find `dy/dx` for the following functions:`y= x^3- 3x^2 + 3x - (2/5)`.
View SolutionFind `dy/dx` for the following functions:`y= ((x-1)(x-2))/sqrtx`.
View SolutionFind `dy/dx` for the following functions: `y= (sqrt x + (1/sqrt x))^2`.
View SolutionDifferentiate the following functions: `(x^2 -4x +5)(x^3 -2)`.
View SolutionDifferentiate the following functions:`((2x +3)/(x^2-5))`.
View SolutionDifferentiate the following functions: `((sin x+ cosx)/(sin x - cos x)`.
View SolutionDifferentiate the following functions: `(4x^3 - 5x^2 + 1)^4.
View SolutionIf the motion of aparticle is governed by the equation, `s= 2t^3- 3t^2+ 2t +2`, find the poswition, velocity and acceleration of the particle at time `t=2s`.
View SolutionThe angular displacement of a particle performing circular motion is
`theta=(t^(3))/(60)-(t)/(4)`
where ` theta` is in radian and 't' is in second .Then the angular velocity and angular acceleraion of a particle at the end of 5 s will be
View SolutionShow that force can be expressed as the product of mass and acceleration. [Hint `F=(dp/dt)-(d/dt)mv- m(dv/dt)-ma`]
View SolutionIntegrate the following: `6x + 5x^2 - 2x^3 + (1/x^2)`
View SolutionIntegrate the following: `ax^2 +bx+c`
View SolutionIntegrate the following:`(x+(1/x))^3`
View SolutionIntegrate the following:` 3 cosec^2 x- 5x+ sinx)`
View SolutionIntegrate the following: `3cosec^2 x +2sin 3x)`
View SolutionEvaluate the following integrals:
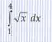
View SolutionEvaluate the following integrals:
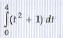
View SolutionEvaluate the following integrals:
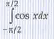
View SolutionEvaluate the following integrals:
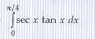
View SolutionEvaluate the following integrals:
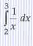
View Solution
Exercise questions and complete solutions for chapter Mathematical tools of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11