Complete Solutions to Problem of chapter FLUIDS AT REST of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | FLUIDS AT REST |
| SL ARORA | Problem |
| Number Of Questions | 19 |
| Academic Year | 2023-24 |
The height to which a cylindrical vessel be filled with a homogenous liquid, to make the average force with which the liquid presses the side of the vessel equal to the force exerted by the liquid on the bottom of the vessel, is equal to.
View SolutionA U-tube of uniform cross-section shown in figure is partially filled with liquid Igt Another liquid II which does not mix with I is poured into one side. The liquid levels of the two sides is found the same, while the level of liquid I has risen by 2 cm. If the specific gravity of liquid I is 1.1, then specific gravity of liquid II must be
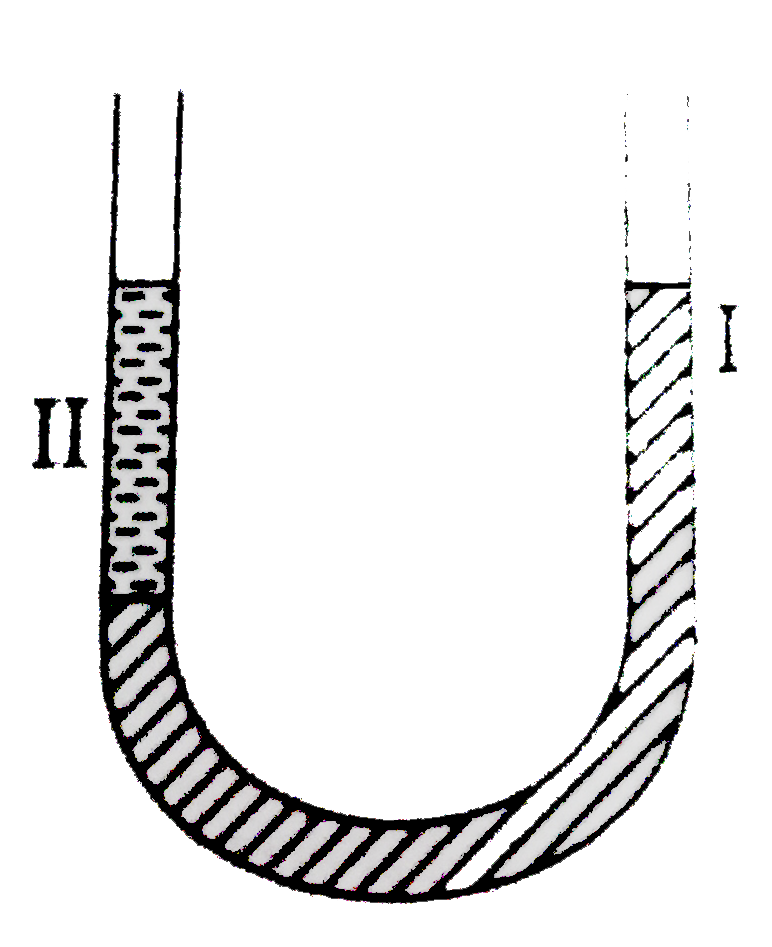
View SolutionA boat floating in a water tank is carrying a number of large stones. If the stones are unloaded into water, what will happen to the water level?
View SolutionVessel contains oil `(density 0.8 g//c c)` over mercury (density `13.6 g//c c)` A homogeneous sphere floats with half its volume immersed in mercury and the other half in oil. The density of the sphere in g/c c is
View SolutionAn iceberg weighs 400 tonnes. The specific gravity of iceberg is 0.92 and the specific gravity of water is 1.02. what percentage of iceberg is below the water surface?
View SolutionA balloon filled with hydrogen has a volume of 1000 litres and has mass of 1kg. What would be the volume of the block of very light material which it can just lift? One litre of the material has a mass 91.3g and density of air is `1.3 g" ""litre"^(-1).`
View SolutionA cubical block of iron 5 cm on each side is floating on mercury in a vessel. (i) what is the height of the block above mercury level ?
(ii) Water is poured in the vessel until it just covers the iron block. What is the height of water column . density of mercury `13.6 gm//cm^(3)`. Density of iron 7.2 `gm//cm^(3)`
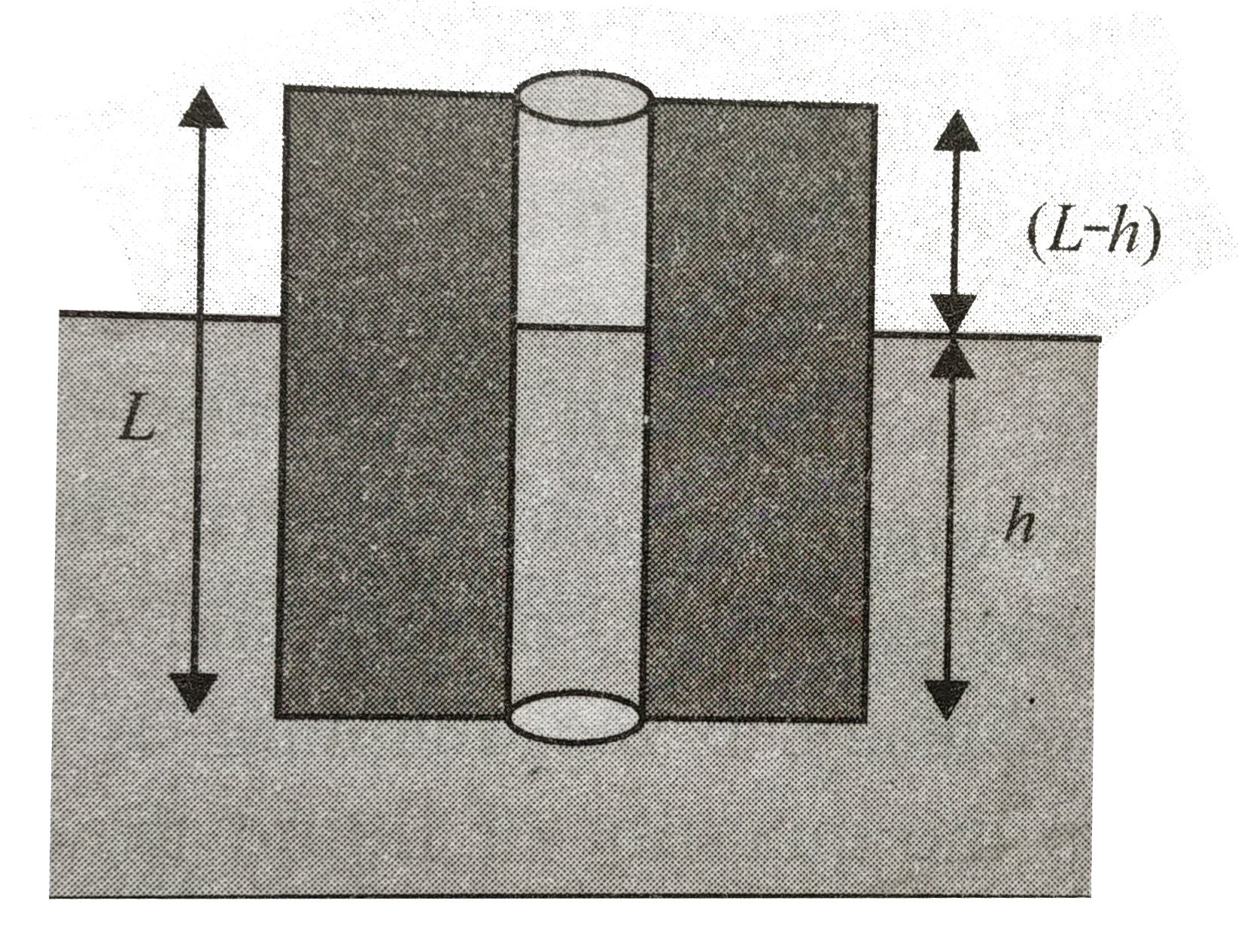
View SolutionA large block of ice `5 m` thick has a vertical hole drilled through it and is floating in the middle of a lake. What is the minimum length of a rope required to scoop up a bucket full of water through the hole? Relative density of ice `=0.9`.
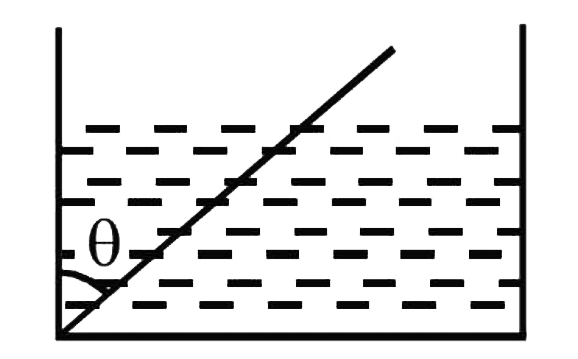
View SolutionA wooden plank of length 1m and uniform cross-section is hinged at one end to the bottom of a tank as shown in fig. The tank is filled with water upto a hight 0.5m. The specific gravity of the plank is 0.5. Find the angle `theta` that the plank makes with the vertical in the equilibrium position. (Exclude the case `theta=theta^@`)
View SolutionA film of water is formed between two straight parallel wires each `10 cm` long and at a seperation of `0.5 cm`. Calculate the work required to increase `1mm` distance between the wires. Surface tension of water `=72xx10^(-3)N//m`.
View SolutionWater rises in a capillary tube upto a certain height such that the upward force of surface tension balances the force of `75 xx 10^(-5)N` due to weight of the liquid. If surface tension of water is ` 6 xx 10^(-2)Nm^(-1)`, what must be the internal circumference of the capillary tube?
View SolutionA ring is cut from a platinum tube `8.5 cm` internal and `8.7 cm` external diameter. It is supported horizontally from the pan of a balance, so that it comes in contact with the water in a glass vessel. If an extra `3.103 g.f.` is required to pull it away from water, the surface tension of water is
View SolutionThere is a soap bubble of radius `2.4 xx 10^(-4)m` in air cylinder at a pressure of `10^(5) N//m^(2)`. The air in the cylinder is compressed isothermal until the radius of the bubble is halved. Calculate the new pressure of air in the cylinder. Surface tension of soap solution is `0.08 Nm^(-1)`.
View SolutionTwo separate air bubbles (radii `0.002 cm` and `0.004`) formed of the same liquid (surface tension `0.07 N//m`) come together to form a double bubble. Find the radius and the sense of curvature of the internal film surface common to both the bubbles.
View SolutionTwo soap bubble of radii `r_(1)` and `r_(2)` combime to form a single bubble of radius r under isothermal conditions . If the external pressure is P, prove that surface tension of soap solution is given by `S=(P(r^(3)-r_(1)^(3)-r_(2)^(3)))/(4(r_(1)^(2)+r_(2)^(2)-r^(2)))`.
View SolutionA long capillary tube of radius 1 mm, open at both ends is filled with water and placed vertically. What will be the height of water column left in the capillary ? (Surface tension of water is `73.5xx10^(-3)Nm^(-1))`
View SolutionA glass capillary sealed at the upper end is of length `0.11 m` and internal diameter `2xx10^(5)`m. The tube is immersed vertically into a liquid of surface tension `5.06xx10^(-2)N//m`. To what length the capillary has to be immersed so that the liquid level inside and outside the capillary becomes the same. What will happen to water level inside the capillary if the seal is now broken?
View SolutionA tapering glass capillary tube A of length `0.1 m` has diameters `10^(-3)` m and `5 × 10^(4)` m at the ends. When it is just immersed in a liquid at `0^(@)`C with larger radius in contact with liquid surface, the liquid rises `8 × 10^(-2)` m in the tube. In another experiment, in a cylindrical glass capillary tube B, when immersed in the same liquid at `0^(@)`C, the liquid rises to `6 × 10^(-2)` m height. The rise of liquid in tube B is only `5.5 × 10^(-2)` m when the liquid is at `50^(@)`C. Find the rate at which the surface tension changes with temperature considering the change to be linear. The density of liquid is `(1//14) × 10^(4) kg//m^(3)` and the angle of contact is zero. Effect of temperature on the density of liquid and glass is negligible.
View SolutionTwo spherical soap bubble coalesce. If `V` is the consequent change in volume of the contained air and `S` the change in total surface area, show that
`3PV+4ST=0`
where `T` is the surface tension of soap bubble and `P` is
Atmospheric pressure
View Solution
Problem questions and complete solutions for chapter FLUIDS AT REST of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11