Complete Solutions to PROBLEM of chapter IIT SUPPLEMENT of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | IIT SUPPLEMENT |
| SL ARORA | PROBLEM |
| Number Of Questions | 38 |
| Academic Year | 2023-24 |
A cube has a side of length `1.2xx10^(-2)m`. Calculate is volume:
View SolutionThe nth division of main scale coincides with (n +1) th division of vernier scale. Given one main division is equal to 'a' units. Find the least count of the vernier.
View SolutionOn a frictionless horizontal surface , assumed to be the ` x-y` plane , a small trolley `A` is moving along a straight line parallel to the `y-axis `( see figure) with a constant velocity of `(sqrt(3)-1) m//s ` . At a particular instant , when the line `OA` makes an angle of `45(@)` with the `x - axis ` , a ball is thrown along the surface from the origin `O`. Its velocity makes an angle `phi` with the `x -axis and it hits the trolley .
(a) The motion of the ball is observed from the frame of the trolley . Calculate the angle `theta` made by the velocity vector of the ball with the ` x-axis in this frame .
(b) Find the speed of the ball with respect to the surface , if ` phi = (4 theta )//(4)`.

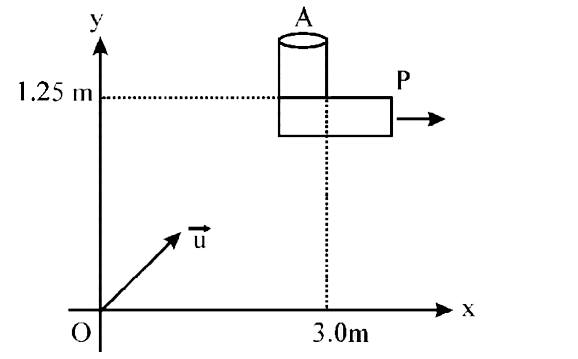
View SolutionAn object `A` is kept fixed at the point ` x= 3 m` and `y = 1.25 m` on a plank `p` raised above the ground . At time ` t = 0 ` the plank starts moving along the `+x` direction with an acceleration ` 1.5 m//s^(2) `. At the same instant a stone is projected from the origin with a velocity `vec(u)` as shown . A stationary person on the ground observes the stone hitting the object during its downward motion at an angle ` 45(@)` to the horizontal . All the motions are in the ` X -Y `plane . Find ` vec(u)` and the time after which the stone hits the object . Take ` g = 10 m//s`
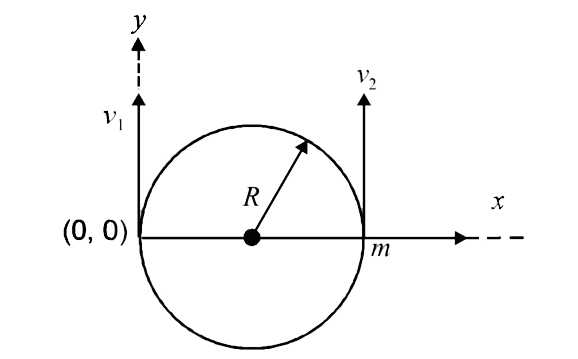
View SolutionA particle of mass m, moving in a cicular path of radius R with a constant speed `v_2` is located at point (2R,0) at time `t=0` and a man starts moving with a velocity `v_1` along the +ve y-axis from origin at time `t=0`. Calculate the linear momentum of the particle w.r.t. the man as a function of time.
View SolutionTwo particles of masses `m_(1)` and `m_(2)` in projectile motion have velocities `vec(v)_(1)` and `vec(v)_(2)` , respectively , at time `t = 0`. They collide at time `t_(0)`. Their velocities become `vec(v')_(1)` and `vec(v')_(2)` at time ` 2 t_(0)` while still moving in air. The value of `|(m_(1) vec(v')_(1) + m_(2) vec(v')_(2)) - (m_(1) vec(v)_(1) + m_(2) vec(v)_(2))|`
View SolutionThe pulleys and strings shown in the figure are smooth and of negligible mass. For the system to remain in equilibrium, the angle `theta` should be :
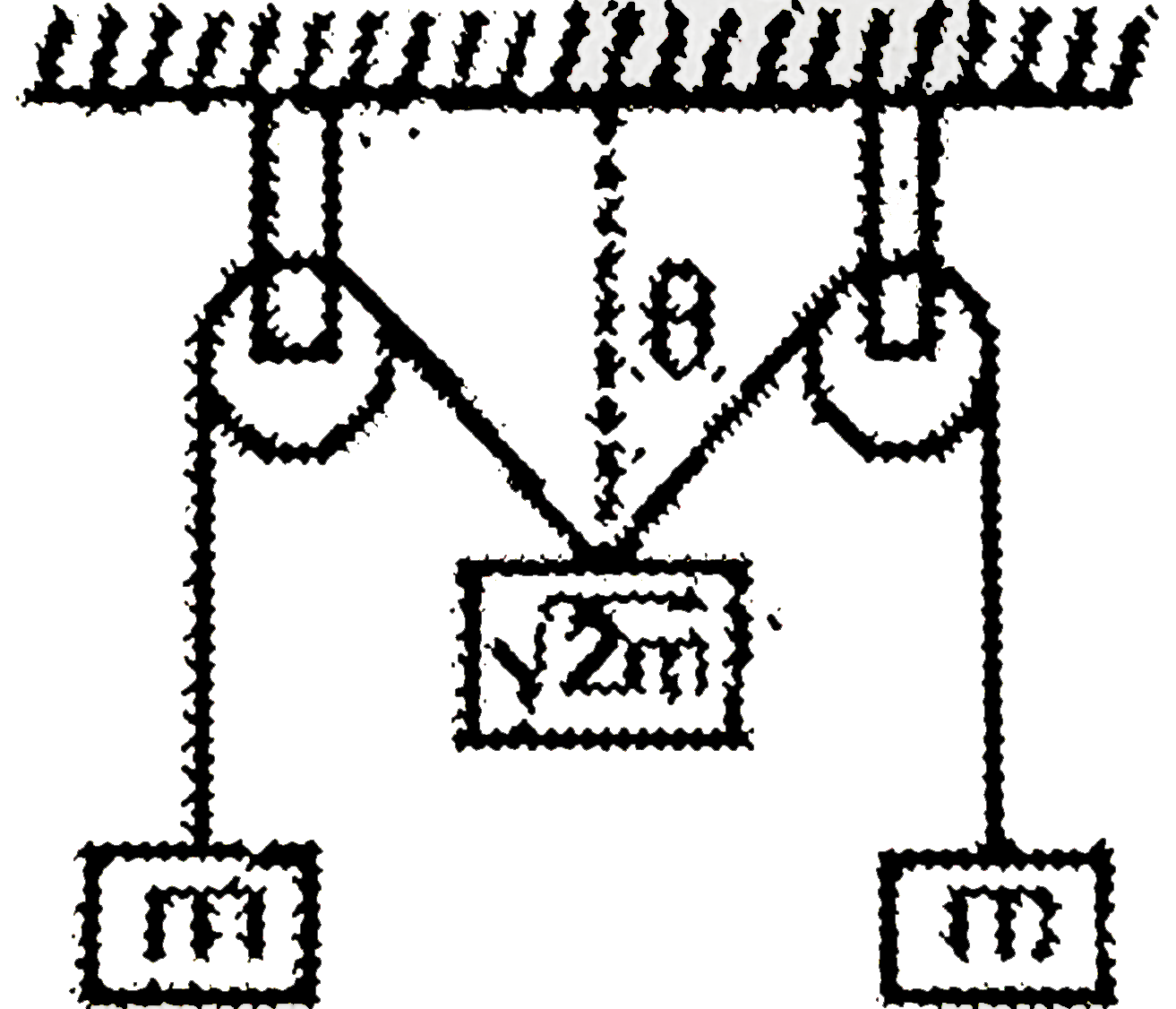
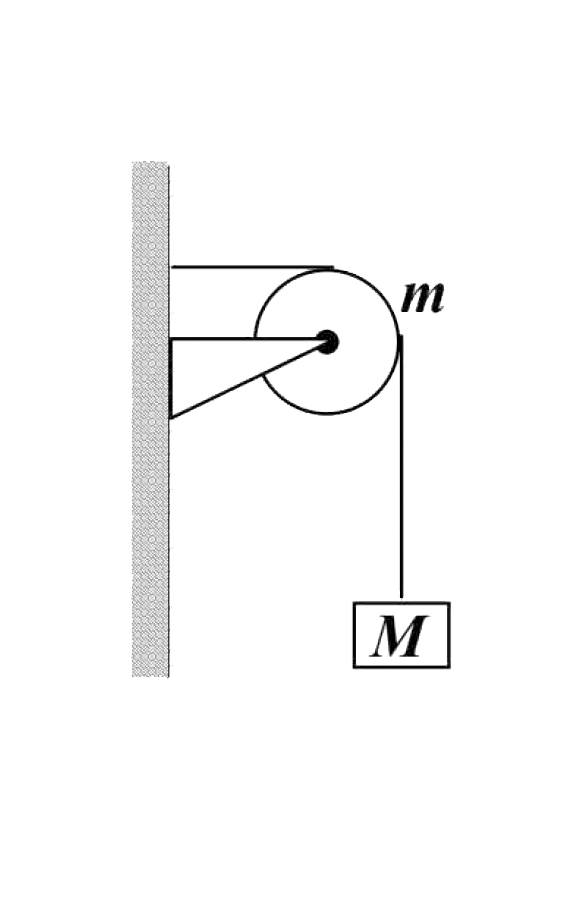
View SolutionA string of negligible mass going over a clamped pulley of mass m supports a block of mass M as shown in the figure. The force on the pulley by the clamp is given by
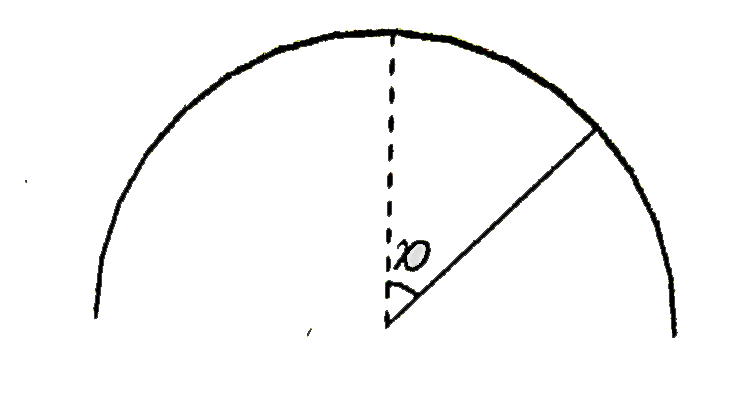
View SolutionAn insect crawls up a hemispherical surface very slowly (see the figure). The coefficient of friction between the insect and the surface is 1/3. If the line joining the centre of the hemispherical surface to the insect makes an angle `alpha` with the vertical, the maximum possible value of `alpha` is given by
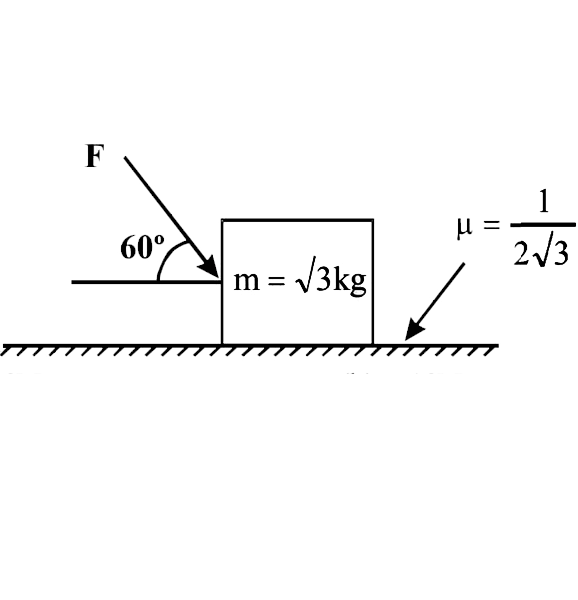
View SolutionWhat is the maximum value of the force F such that the block shown in the arrangement, does not move?
View SolutionIn the figure masses `m_1`, `m_2` and M are 20 kg, 5kg and 50kg respectively. The coefficient of friction between M and ground is zero. The coefficient of friction between `m_1` and M and that between `m_2` and ground is 0.3. The pulleys and the strings are massless. The string is perfectly horizontal between `P_1` and `m_1` and also between `P_2` and `m_2`. The string is perfectly vertical between `P_1` and `P_2`. An external horizontal force F is applied to the mass M. Take `g=10m//s^2`.
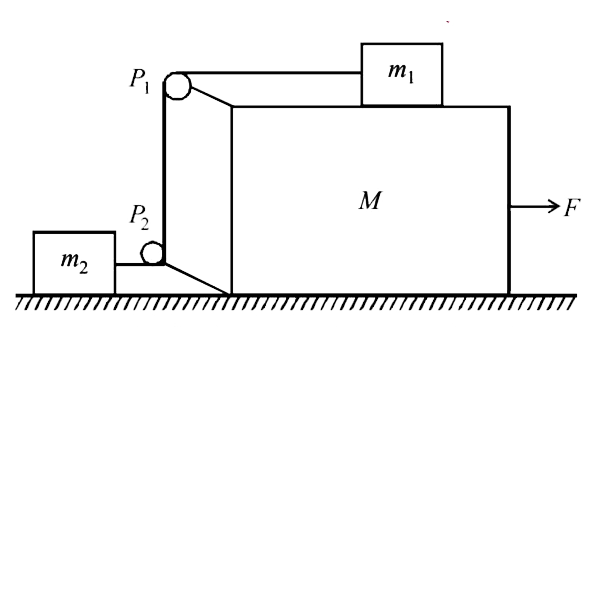
(a) Draw a free body diagram for mass M, clearly showing all the forces.
(b) Let the magnitude of the force of friction between `m_1` and M be `f_1` and that between `m_2` and ground be `f_2`. For a particular F it is found that `f_1=2f_2`. Find `f_1` and `f_2`. Write equations of motion of all the masses. Find F, tension in the spring and acceleration of masses.
View SolutionA wind - powered generator convets and energy into electrical energy . Assume that the generator convents a fixed fraction of the wind energy intercepited by to blades into electrical energy for wind speed `V` , the electrical power output will be propertional to
View SolutionAn ideal spring with spring constant k is hung from the ceiling and a block of mass M is attached to its lower end. The mass is released with the spring initially unstretched. Then the maximum extension in the spring is
View SolutionA car P is moving with a uniform speed `5sqrt3 m//s` towards a carriage of mass 9 kg at rest kept on the rails at a point B as shown in figure. The height AC is 120 m. Cannon balls of 1 kg are fired from the car with an initial velocity `100m//s` at an angle `30^@` with the horizontal. The first cannon hall hits the stationary carriage after a time`t_0` and sticks to it. Determine `t_0`.
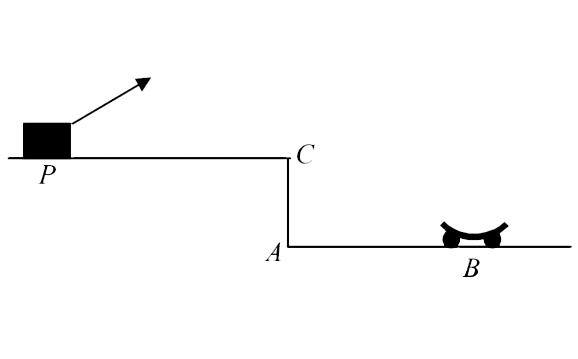
At `t_0`, the second cannon ball is fired. Assume that the resistive force between the rails and the carriage is constant and ignore the vertical motion of the carriage throughout. If the second ball also hits and sticks to the carriage, what will be the horizontal velocity of the carriage just after the second impact?
View SolutionTwo blocks of masses 10 kg and 4 kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse gives a velocity of `14 m//s` to the heavier block in the direction of the lighter block. The velocity of the centre of mass is
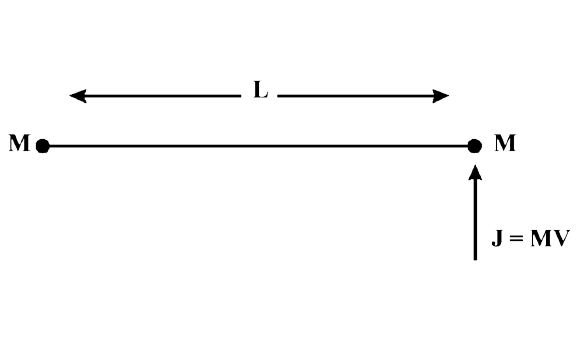
View SolutionConsider a body, shown in figure, consisting of two identical balls, each of mass M connected by a light rigid rod. If an impulse J = MV is imparted to the body at one of its ends what would be it angular velocity?
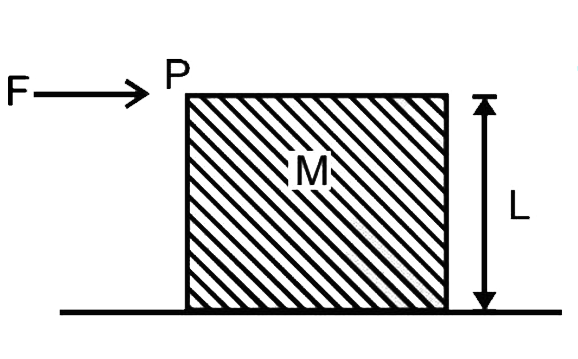
View SolutionA cubical block of side L rests on a rough horizonta surface with coefficient of friction `mu`. A horizontal force F is applied on the block as shown. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, the minimum force required to topple the block is
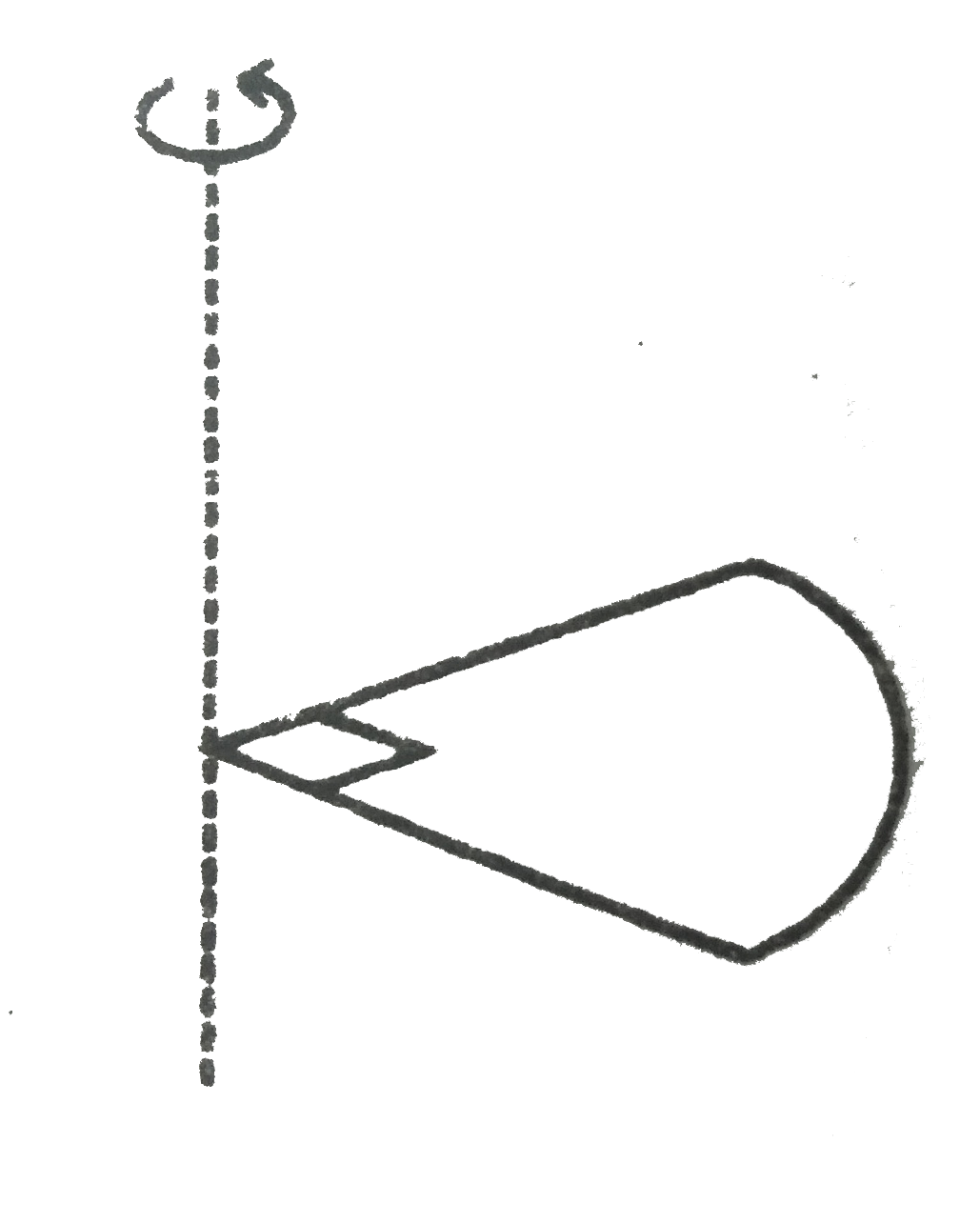
View SolutionOne quarter sector is cut from a uniform circular disc of radius `R`. This sector has mass `M`. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. It moment of inertia about the axis of rotation is.
 .
.
View SolutionA thin wire of length `L` and uniform linear mass density `rho` is bent into a circular loop with centre at `O` as shown. The moment of inertia of the loop about the axis `XX'` is :
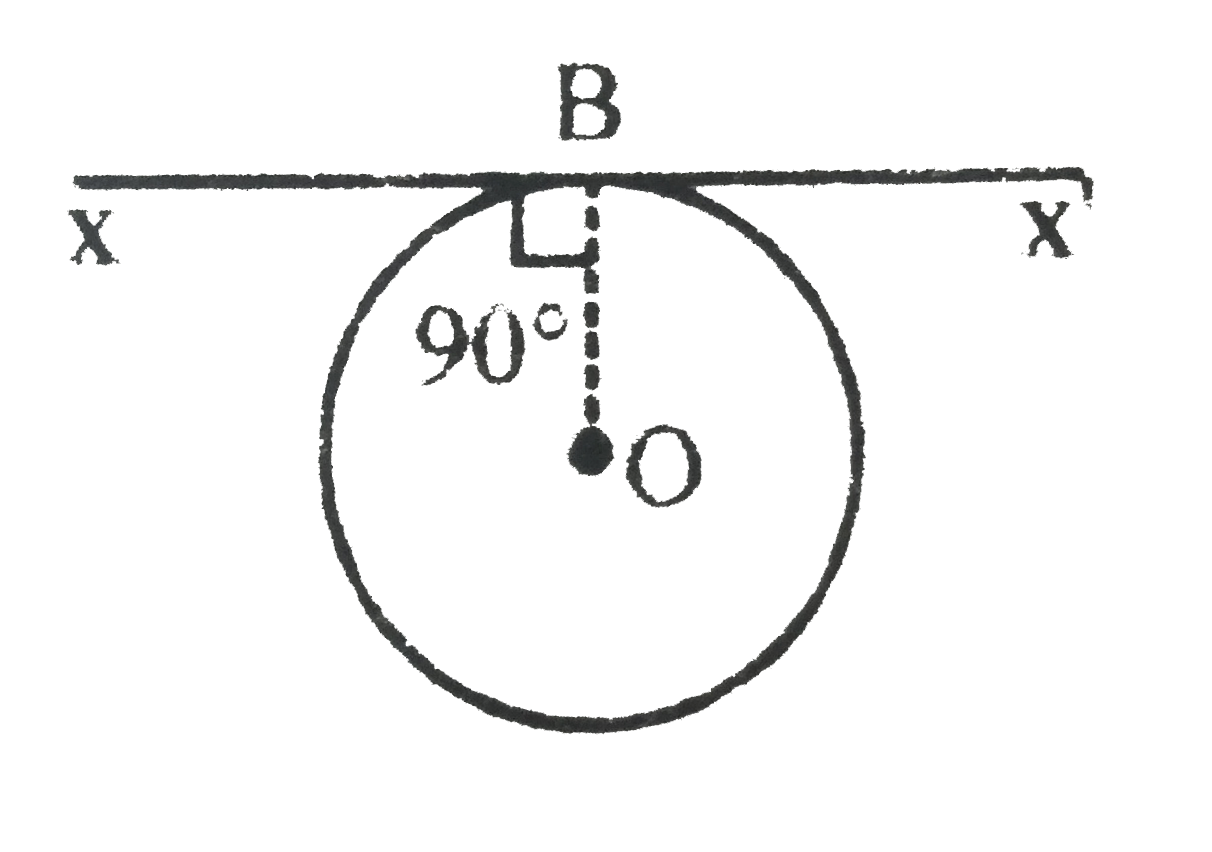 .
.
View SolutionA long horizontal rod has a bead which can slide along its length and initially placed at a

distance L from one end A of the rod. The rod is set in angular motion about A with constant angular acceleration `alpha.` if the coefficient of friction between the rod and the bead is `mu`, and gravity is neglected, then the time after which the bead starts slipping is
View SolutionA spherical balll of mass `m` is the highest point in the space between two fixed , concentic sphere `A` and `B` the smaller the two sphere `A` has a radius `R` and the space between the two spheres has a width `d` . The bell has a disneter very dightly less then d . All surface are frictionless . The bell is a given a gentle push (owards the right in the figure ) The upward vertical is denoted by `theta` (shown ijn the figure)
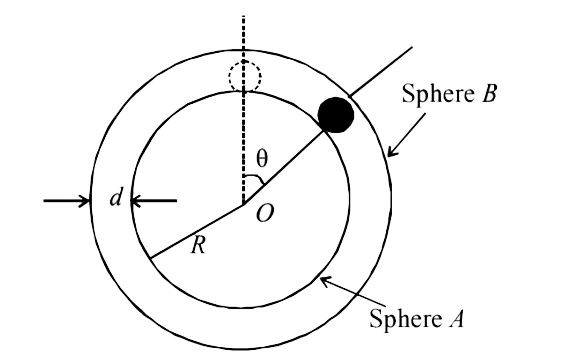
(a)Express the total normal reaction force exerted by the sphore on the as a finction of angle `theta`
(b) Let N_(A)` and N_(B)`denote in the magnitubes of the normal reaction force on the bell evered by the sphare `A` and `B` repectively Skech the variation of `N_(A) and`N_(B)` as functions of `cos theta ` in the range `0 le theta le pi ` by drawing two separate graph in your answer book taking `cos theta ` an the horizental axas.
View SolutionThree particles A, B and C each of mass m, are connected to each other by three massless rigid rods to form a rigid, equilateral triangular body of side l. This body is placed on a horizonta frictionsess table (x-y plane) and is hinged to it at the point A so that it can move without friction about the vertical axis through A . the body is set into rotational motion on the table about A with a constant angular velocity `omega`.
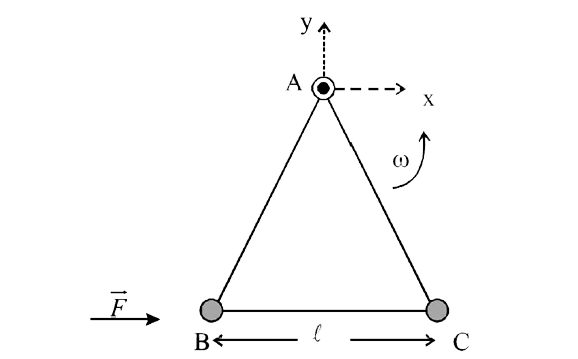
(a) Find the magnitude of the horizontal force exerted by the hinge on the body.
(b) At time T, when the side BC is parallel to the x-axis, a force F is applied on B along BC (as shown). Obtain the x-component and the y-component of the force exerted by the hinge on the body, immediately after time T.
View SolutionA rod AB of mass `M` and length L is lying on a horizontal frictionless surface. A particle of mass `m` travelling along the surface hits the end A of the rod with a velocity `v_(0)` in a direction perpendicular to AB. The collision in elastic. After the collision the particle comes to rest
(a). Find the ratio `m//M`
(b). A point P on the rod is at rest immediately after collision find the distance AP.
(c). Fid the linear speed of the point P a time `piL//3v_(0)` after the collision.
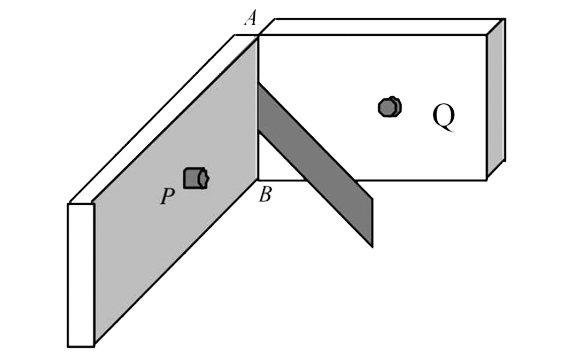
View SolutionTwo heavy metallic plates are joined together at `90^@` to each other. A laminar sheet of mass 30kg is hinged at the line AB joining the two heavy metallic plates. The hinges are frictionless. The moment of inertia of the laminar sheet about an axis parallel to AB and passing through its center of mass is 1.2 kg `m^2.` Two rubber obstacles P and Q are fixed, one on each metallic plate at a distance 0.5m from the line AB. This distance is chosen so that the reaction due to the hinges on the laminar sheet is zero during the impact. Initially the laminar sheet hits one of the obstacles with an angular velocity 1 rad/s and turns back. If the impulse on the sheet due to each obstacal is 6 N-s,
(a) Find the location of the center of mass of the laminar sheet from AB.
(b) At what angular velocity does the laminar sheet come back after the first impact?
(c) After how many impacts, does the laminar sheet come to rest?
View SolutionAn insulated container containing monoatomic gas of molar mass s is moving with a velocity `v_0`. If the container is suddenly stopped, find the change in temperature.
View SolutionA cubical box of side `1 m` contains helium gas (atomic weight 4) at a pressure of `100 N//m^2`. During an observation time of `1 second`, an atom travelling with the root - mean - square speed parallel to one of the edges of the cube, was found to make `500 hits` with a particular wall, without any collision with other atoms . Take `R = (25)/3 j //mol - K and k = 1.38 xx 10^-23 J//K`.
(a) Evaluate the temperature of the gas.
(b) Evaluate the average kinetic energy per atom.
( c) Evaluate the total mass of helium gas in the box.
View Solution2kg of ice at `20^@C` is mixed with 5kg of water at `20^@C` in an insulating vessel having a negligible heat capacity. Calculate the final mass of water remaining in the container. It is given that the specific heats of water & ice are `1kcal//kg//^@C` and `0.5`
`kcal//kg//^@C` while the latent heat of fusion of ice is `80kcal//kg`
View SolutionTwo rods, one of aluminium and other made of steel, having initial lengths `l_(1) and l_(2)` are connected together to form a single rod of length `(l_(1)+l_(2))`. The coefficient of linear expansions for aluminium and steel are `alpha_(a)` and `alpha_(s)` respectively. If length of each rod increases by same amount when their tempertures are raised by `t^(@)C`, then find the ratio `l_(1) (l_(1)+l_(2))`.
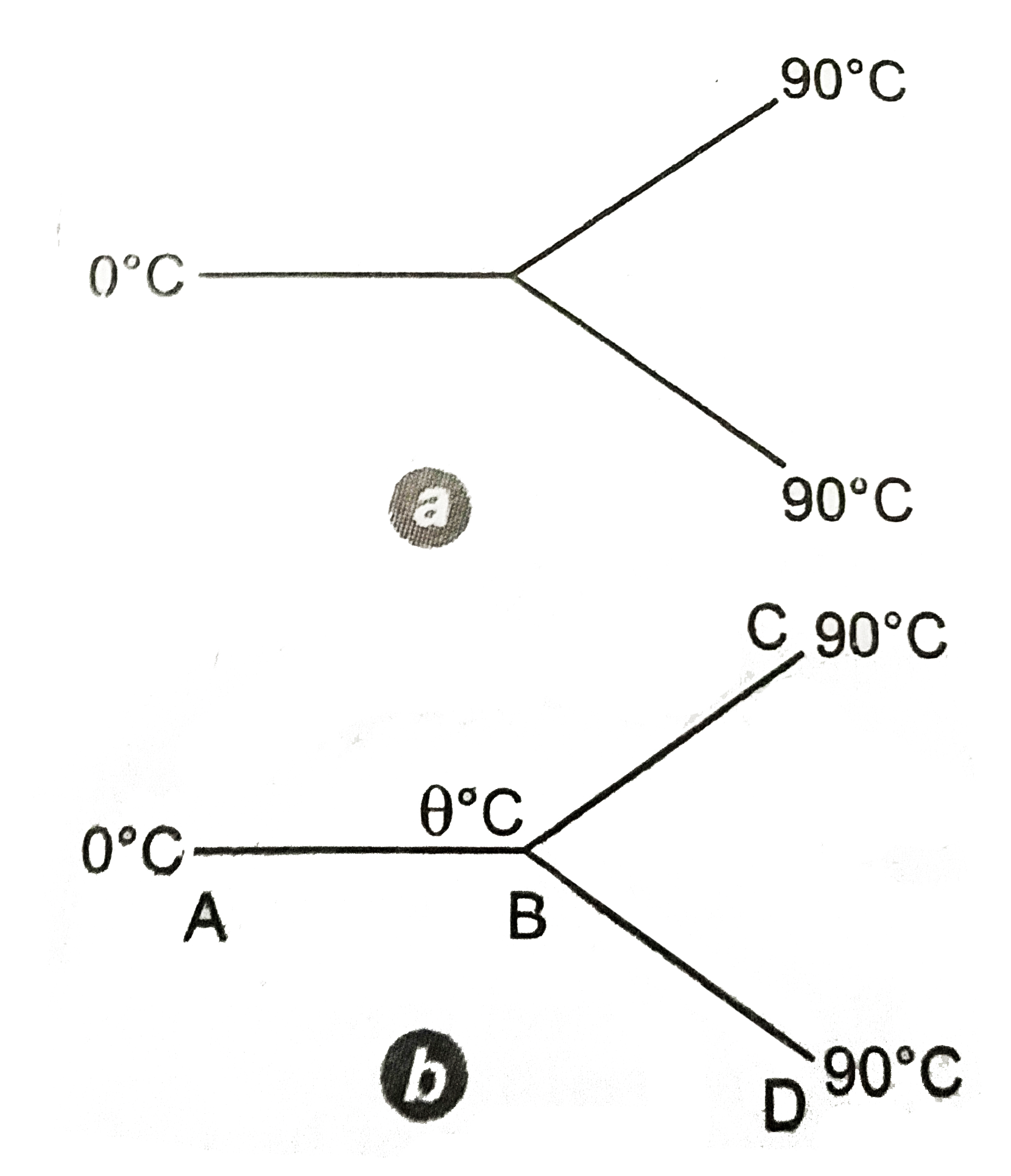
View SolutionThree rods made of same material and having the same cross-section have been joined as shown in fig. each rod is of the same length. The left and the right ends are kept at `0^(@)C` and `90^(@)C` respectively. What will be the temperature of the junction of the three rods?
View SolutionWhen a block of iron in mercury at `0^@C,` fraction `K_1`of its volume is submerged, while at the temperature `60^@C,` a fraction `K_2` is seen to be submerged. If the coefficient of volume expansion of iron is `gamma_(Fe)` and that of mercury is `gamma_(Hg),` then the ratio `(K_1)//(K_2)` can be expressed as
View SolutionIn a given process on an ideal gas, `dW=0 and dQlt0.` Then for the gas
View SolutionA gas is taken through the cycle `A rarrB rarr C rarr A`, as shown in figure, what is the net work done by the gas?
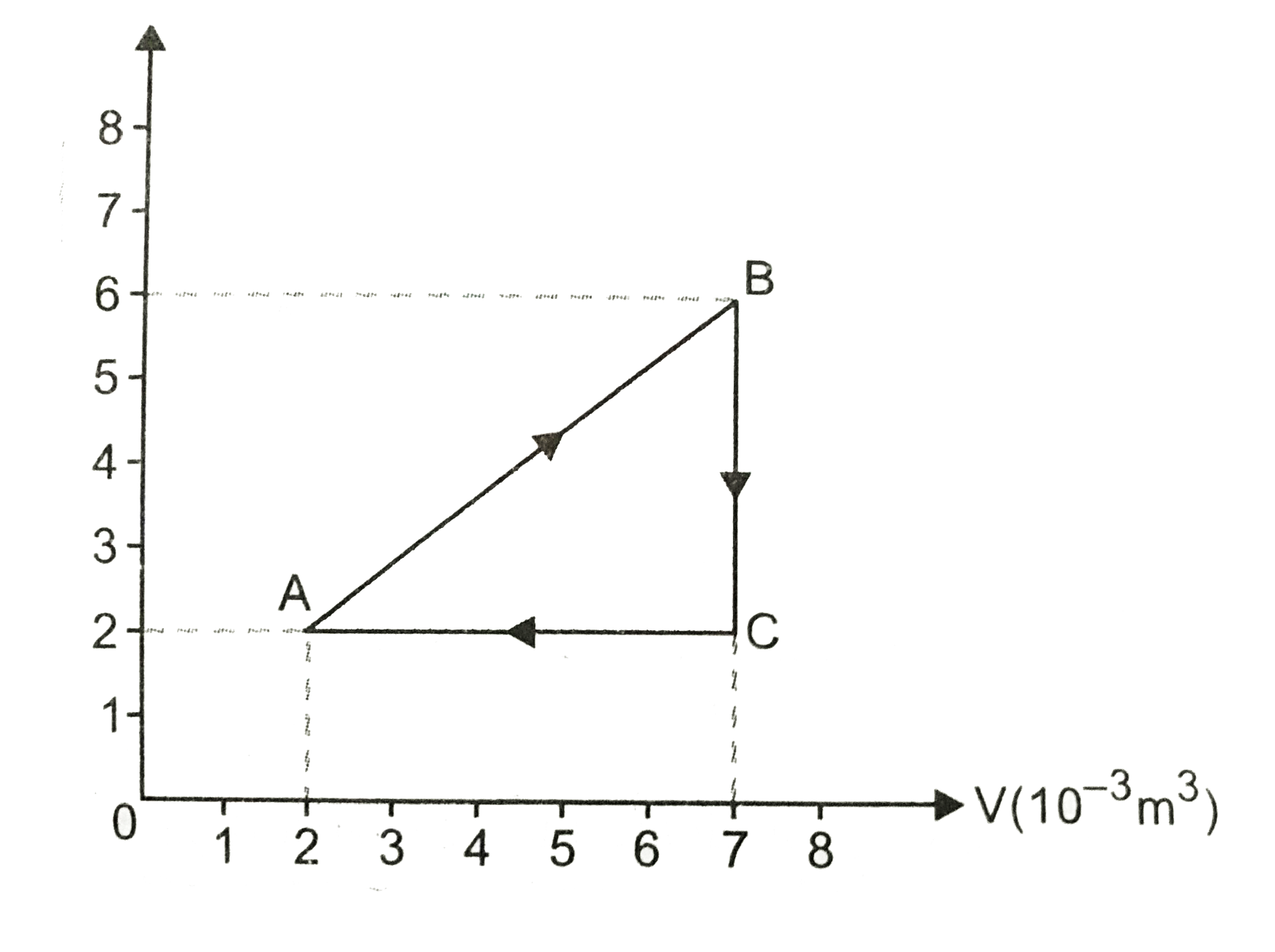
View SolutionA monoatomic ideal gas, initially at temperature `T_1,` is enclosed in a cylinder fitted with a friction less piston. The gas is allowed to expand adiabatically to a temperature `T_2` by releasing the piston suddenly. If `L_1 and L_2` are the length of the gas column before expansion respectively, then `(T_1)/(T_2)` is given by
View SolutionAn ice cube of mass 0.1 kg at `0^@C` is placed in an isolated container which is at `227^@C`. The specific heat s of the container varies with temperature T according to the empirical relation `s=A+BT`, where `A= 100 cal//kg.K and B = 2xx 10^-2 cal//kg.K^2`. If the final temperature of the container is `27^@C`, determine the mass of the container.
(Latent heat of fusion for water = `8xx 10^4 cal//kg`, specific heat of water `=10^3 cal//kg.K`).
View SolutionHot oil is circulated thorugh an insulated container with a wooden lid at the top whose conductivity `K=0.149J//(m-^@C-sec)`, thickness `t=5mm, emissivity=0.6` Temperature of the top of the lid is maintaining at `T_l=127^@C`. If the ambient temperature `T_a=27^@C`.
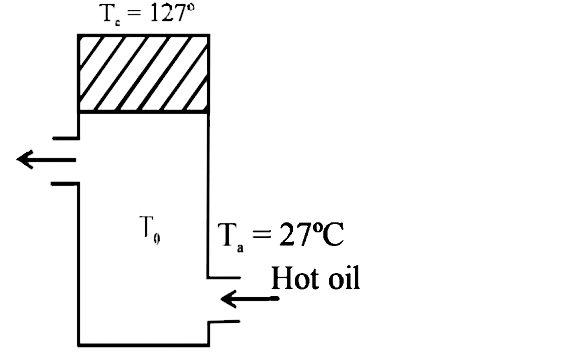
Calculate:
(a) rate of heat loss per unit area due to radiation from the lid.
(b) temperature of the oil. `(Given sigma=17/3xx10^-8 Wm^-2K^-4)`
View SolutionTwo moles of an ideal monoatomic gas is taken through a cycle ABCA as shown in the P-T diagram. During the process AB , pressure and temperature of the gas very such that `PT=Constant`. It `T_1=300K`, calculate
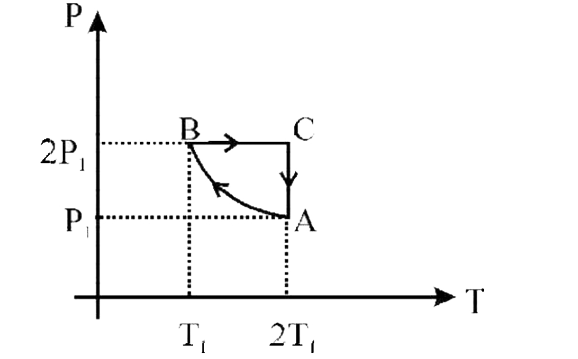
(a) the work done on the gas in the process AB and
(b) the heat absorbed or released by the gas in each of the processes. Give answer in terms of the gas constant R.
View SolutionA monoatomic ideal gas of two moles is taken through a cyclic process starting from A as shown in figure. The volume ratios are `(V_B)/(V_A)=2 and (V_D)/(V_A)=4`. If the temperature `T_A` at A is `27^@C`.
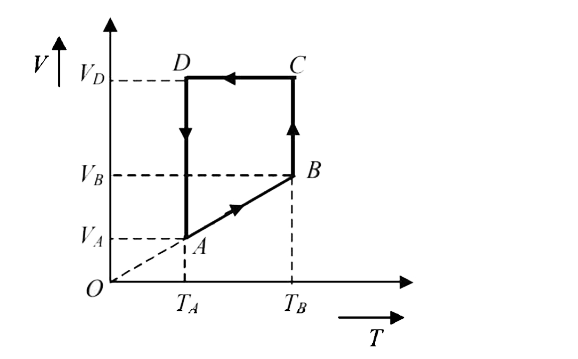
Calculate,
(a) the temperature of the gas at point B,
(b) heat absorbed or released by the gas in each process,
(c) the total work done by the gas during the complete cycle. Express your answer in terms of the gas constant R.
View SolutionA particle executes simple harmonic motion between `x = -A and x = + A`. The time taken for it to go from `0 to A//2 is T_1 and to go from A//2 to (A) is (T_2)`. Then.
View Solution
PROBLEM questions and complete solutions for chapter IIT SUPPLEMENT of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11