Complete Solutions to Part-D of chapter I PUC ANNUAL EXAMINATION QUESTION PAPER -2019 ( NORTH )-9 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | I PUC ANNUAL EXAMINATION QUESTION PAPER -2019 ( NORTH )-9 |
| SUBHASH PUBLICATION | Part-D |
| Number Of Questions | 10 |
| Academic Year | 2023-24 |
Define modulus function, draw the graph of it, write its domain and range.
View SolutionProve that ` cos ^(2) x+ cos ^(2) (x+ ( pi )/( 3)) +cos ^(2) (x- (pi)/(3)) =(3)/(2) `
View Solution`1^(2)+2^(2)+3^(2)+............+n^(2)=(n(n+1)(2n+1))/6 forall n in N.`
View SolutionSolve the following system of inequalities graphically :
`5x + 4y le 20, x ge 1, y ge 2 `
View SolutionA group consists of 4 girls and 7 boys. In how ways can a team of 5 members be selected, if he team has.
At least one boy and one girl?
View SolutionProve binomial theorem for positive integers
View SolutionDerive the equation of a straight line having the intercepts 'a' & 'b' on the X ane Y-axes respectively. Hence find the equation of the line intercepts -3 and 2 on the X and Y-axes respectively.
View SolutionDerive the formula for the distance between two points`(x_1,y_1,z_1) and (x_2,y_2,z_2)` and hence find the distance between P(1,-3,4) and Q(-4,1,2).
View SolutionProve that `Lim _( xto 0) ( sin x) /( x ) =1` ( x being measured in radians )
View SolutionFind the mean deviation about the mean for the following data.
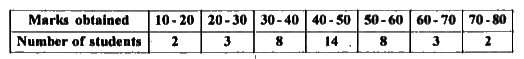
View Solution
Part-D questions and complete solutions for chapter I PUC ANNUAL EXAMINATION QUESTION PAPER -2019 ( NORTH )-9 of JEEVITH PUBLICATIONS MATHS (KANNADA ENGLISH) of Class 11