Complete Solutions to EXERCISE of chapter ANNUAL EXAMINATION QUESTION PAPERS 2016 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | ANNUAL EXAMINATION QUESTION PAPERS 2016 |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 51 |
| Academic Year | 2023-24 |
If f(x)=`log_3x` and `phi(x)=x^2`,then the value of `f{varphi(3)}`will be
View SolutionIf `tan theta=-4/3`,then the value of `sin theta` is
View SolutionIf the ratio of the sum of first three terms to the sum of next three terms of a geometric series be 125:27,then the common ratio of the series be
View SolutionIf the point `(lambda,1+lambda)`be lying inside the circle `x^2+y^2`=1 then
View SolutionThe distance between the points A(5,1,2) and B(4,6,-1)is
View SolutionIf the points `A(2,beta,3)`,`B(alpha,-5,1)`and C(-1,11,9) are collinear,then, find the values of alpha and beta.
View SolutionThe value of `lim_(xrarr0)(sin5x)/(tan 3x)` is
View SolutionIf `y=sqrt((1-cos2x)/(1+cos2x))`,then the value of dy/dx will be
View SolutionFor two mutually exclusive events A and B,P(A)=1/2and `P(AcupB)=2/3`,then the value of P(B) will be
View SolutionIf `i^2=-1`,then the value of modulus of `(3i-1)^2`will be
View SolutionFind the domain of the function `f(x)=(x^(2)+3x+5)/(x^(2)-5x+4)`
View SolutionIf `f(x)=e^(px+q)`,then shoq that `f(a).f(b).f(c)=f(a+b+c).e^(2q)`
View Solutionif `13theta=pi`,then show that `cos3theta+cos5theta+2costheta.cos9theta=0`
View SolutionShow that `secalpha-tanalpha=cot(pi/4+alpha/2)`
View SolutionFind the square root of i
View SolutionFind the (r+1)th term from the end in the expansion of `(1-3x)^n`.
View SolutionProve that by using the principle of mathematical induction for all `n in N`:
`10^(2n-1)+1` is divisible by 11
View SolutionLet the sum of n, 2n, 3n terms of an A.P. be `S_1, S_2 and S_3` , respectively, show that `S_3 =3(S_2-S_1)`
View SolutionFind the locus of the mid-point of the portion of the line `x cosalpha+ysinalpha=4`intercepted between the axes of coordinates.
View SolutionIf the coordinates of a point lies on the ellipse `9x^2+16y^2=144` be `(2,(3sqrt3)/2)`,find the eccentric angle of that point.
View SolutionIf `y=e^x/(1+x^2)`,determin dy/dx.
View SolutionEvaluate :`lim_(xrarrpi/4)(1-tanx)/(x-pi/4)`
View Solutioncan `7/2`be the probability of an event? Explain.
View SolutionTwo variables x and y are related by y=10-3x.If the standard deviation of x be 4,find the standard deviation of y.
View SolutionFor any three sets A,B and C prove that `Acup(BcapC)=(AcupB)cap(AcupC)`
View SolutionFind the value of `16cos(pi/15).cos((2pi)/15).cos((4pi)/15).cos((8pi)/15)`
View SolutionShow that `sin^3alpha+sin^3(120^@+alpha)+sin^3(240^@+alpha)=-3/4sin3alpha`
View SolutionProve that by using the principle of mathematical induction for all `n in N`:
`1.2+ 2.3+ 3.4+ .....+n(n+1)= [(n(n+1)(n+2))/(3)]`
View SolutionIf the `P^(th)`and `q^(th)`terms of an A.Pare a and b respectively,then show that the sum of first (p+q) terms of thath A.P is 1/2(p+q)`(a+b+(a-b)/(p-q))`
View SolutionFind the probability of drawing 4 cards from a pack of 52 cards such that at least two cardswill be aces.
View SolutionFind the sum of n-terms of the following series:`(x+1/x)^2+(x^2+1/x^2)^2+(x^3+1/x^3)^2+(x^4+1/x^4)^2+...`
View SolutionThe equations of two sides of a square are 5x+12y-10=0 and 5x+12y+29=0 and the third side passes through (3,5):find equations of all other possible sides of the square.
View SolutionFind the equation of the circle passes through the points (4,3) and (-2,5) and whose centre lies on the lin 2x-3y=4
View SolutionShow that the area of the triangle formed by the lines `y = m_(1) x + c_(1) , y = m_(2) x + c_(2) " and " x = 0 " is " ((c_(1) - c_(2))^(2))/(2|m_(1) - m_(2)|)`
View SolutionFind the value of cos B for the triangle formed by joining the points A(6,11,2),B(1,-1,2)and C(1,2,6).
View SolutionEvlauate `lim_(xrarr0)(cot2x-cosec2x)/x`
View SolutionFind from the first principle,the derivative of `f(x)=e^(x^2)`at x=1.
View SolutionProve the following by contradiciton ."The sum of a rational and an irrational number is an irrational number?".
View SolutionCheck the validity of the following compound propostions :"72 is a multiple of both 4 and 9"
View SolutionCheck the validity of the following compound propostions :"120 is a multiple of both 15 and 9"
View SolutionTwo students Anil and Ashima appeared is an examination. The probability that Anil will qualify the examination is 0.05 and that Ashima will qualify the examination is 0.10. The probability qualify the examination is 0.02. Find the probability that
(a) Bothe Anil and Ashima will not qualify the examination.
(b) Atleast one of them will qualify the examination and (c) Only one of them will qualify the examination.
View SolutionFind the coefficient of variation of the following data:
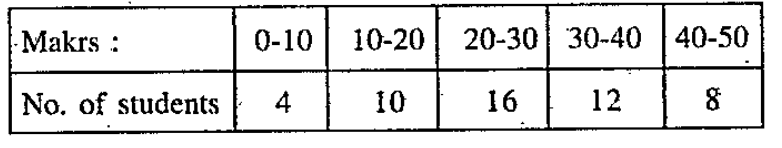
View SolutionFind the general solution of x and y satisfying the equation 5sinx.cosy=1 and 4 tanx=tany.
View SolutionFind the domain of definition and range of the function `f(x)=x/(1+x^2)`.
View SolutionIf `f(x)=ax^2+bx+c`and f(2)=1,f(3)=6,f(-1)=10,then find the value of f(1).
View SolutionIf z=x+iy and |z-1|+|z+1|=4,then show that `3x^2+4y^2=12`,where `i=sqrt-1`
View SolutionIn how many ways can 6 boys and 4 girls be seated in a round table so that two girls never be seated together.
View SolutionIf `a_1,a_2,a3,...,a_n`are in A.P then show that `1/(a_1a_2)+1/(a_2a_3)+1/(a_3a_4)+...+1/(a_(n-1)a_n)=(n-1)/(a_1a_n)`
View SolutionSolve :`(|x|-2)/(|x|-3)ge0`,where `x in R` and `x!=+-3`
View SolutionIf the extremitied of a focal chord of the parabola `y^2=4ax`be `(at_1^2,2at_1)`and `(at_2^2,2at_2)`,show that `t_1t_2=-1`
View SolutionThe number of tangents that can be drawn from the point (6, 2) on the hyperbola `(x^(2))/(9)-(y^(2))/(4)=1` is
View Solution
EXERCISE questions and complete solutions for chapter ANNUAL EXAMINATION QUESTION PAPERS 2016 of UNITED BOOK HOUSE of Class 11