Complete Solutions to EXERCISE of chapter ANNUAL EXAMINATION QUESTION PAPERS 2017 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | ANNUAL EXAMINATION QUESTION PAPERS 2017 |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
If `BsubeA`,then the set B -A will be ____
View SolutionIf `omega`be the imaginary cube root of 1 ,then the value of `(3+omega+3omega^2)^4`will be ___
View SolutionIf the differnce between the roots of the quadratic equation `x^2+px+8=0`be 2,thn the value of p will be __
View SolutionIf 16C_r = 16C_(2r+1) then the vaue of r is
View SolutionFind the coordinates of the focus , axis, the question of the directrix and latus rectum of the parabola `y^(2)=8x`.
View SolutionThe co-ordinattes of B and C of the trianglle ABC are (5,2,8) and (2,-3,4) respectively.If the centroid of the triangle ABC are (3,-1,3) then the coordinates of A are ___
View SolutionThe value of `Lt_(xrarr0)(e^x-e^-x)/x`is ___
View Solutionif `y=cos^2(x/2)`the value of dy/dx is ___
View SolutionIf `P(AcapB)=5/13`,then the value of `P(A^c cup B^c)`is___
View SolutionIf the variance of a distribution is 4 coefficient of variation is 5%,then mean of the distribution is ___
View SolutionA relation R is defined from A={1,2,3,4,5} to B={1,2,3}in such a way that,`(x,y)in Rimpliesx>y`.Express R a set of ordered pairs.
View SolutionIf (x+y) `prop` (x-y) , then show that `(x^(3) +y^(3)) prop (x^(3)-y^(3))`.
View SolutionShow that `1/(sin10^@)-sqrt3/(cos10^@)=4`
View SolutionFind the value of `2ac sin ((A-B+C)/2)`for `/_\ABC`
View SolutionIf z be a complex number and `|z+5|le6`,then find the maximum and minimum values on |z+2|.
View SolutionIf ``^nP_r=504`and ``^nC_r=84`,then find value of n and r.
View SolutionFind the coefficinet of `1/x^2`in the expansion of `(2x^3-1/x^2)^6`.
View SolutionIf P-th term of an arithmetic progression be Q and Q-th term be P.then show that (P+Q)thterm is O.
View SolutionIf the perimeter of the triangle formed by the straight line 4x+3y+k=0with the coordinate axes be 24 unit,then the value of k.
View SolutionFind the co-ordinates of the point lies on the plane YOZ which is equidistant from the points A(1,-1,0),B(2,1,2) and C(3,2,-1).
View SolutionEvaluate :`lim_(xrarr0)(cos5x-cos7x)/(cosx-cos5x)`
View SolutionIf (x+4)y=x.then show that x dy/dx+y(y-1)=0.
View SolutionIf a coin is tossed 3 times in succession,then find the probability of obtaining tail at least once.
View SolutionThe standard deviation of 32 numbers is 5.If the sum of the numbers is 80,determine the sum of the squares of the numbers.
View SolutionFor any three sets A,B and C ,prove that `A-(BcupC)=(A-B)cap(A-C)`.
View SolutionIf `sin^4alpha/a+cos^4alpha/b=1/(a+b)`,then show that `sin^8alpha/a^3+cos^8alpha/b^3=1/((a+b)^3)`.
View SolutionIf for a triangle ABC , cot A+cotB+cotC=`sqrt3`,then show that the triangle is equilateral.
View SolutionIf n in N,then prove by mathematical induction that 7^(2n)+2^3(n-1).3^(n-1) is always a multipe of 25.
View SolutionIf z=x+iy and `(z-i)/(z+1)`is purely imaginary,then show that the point z always lies on a circle.
View SolutionHow many odd numbers of fve digits can be formed with the digits 3,6,7,2,0 when no digits is repeated?
View SolutionProve that the middle term in the expansion of `(1+x)^(2n)`is`(1.3.5.......(2n-1))/|___n.2^n.x^n`
View SolutionIf the ratio the sum of 1st n terms of two arithemtic series is (4n-13):(3n+10),then find the ration of their ninth terms.
View SolutionFind the equations of the lines passing thorugh the point (4,5)making equal angles with the lines 3x=4y+7 and 5y=12x+6.
View SolutionIf the equation of the side BC of an equilateral triangle ABC is `x+y=2` and the coordinate of the vertex A is (2,3) then find the equation of the other two sides.
View SolutionA circle in the first quadrant touches both the axes its centre lies on the straight line lx+my+n=0.show that the equation of that circle is `(1+m)^2(x^2+y^2)+2n(l+m)(x+y)+n^2=0`
View SolutionEvaluate:`underset(x rarr 0)(lim)((e^(x)-1)log(1+x))/(sin^(2)x)`
View SolutionFind from the first principle,the derivative of f(x)=sec2xat `x=pi/8`.
View SolutionProve by method of contradiction that `sqrt5`is an irrational number
View SolutionProve that truth table ~(pvq)=~p~q.
View SolutionIf 30 dates are named at random,find the probability that 5 of them will be sundays.
View Solutioncalculate the mean deviation about median for the following data:
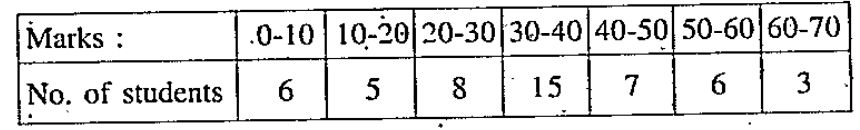
View SolutionSolve: 4 sin x sin 2x sin 4x =sin3x.
View Solutionif `tan (theta/2)=sqrt((1-e)/(1+e))``tan (varphi/2)`,then prove that `cos varphi=(costheta-e)/(I-ecostheta)`
View SolutionSolve the following inequation `(|x+2|+2x)/(x+2)>2`.
View SolutionSolve applying formula: `3x^2-(2-i)x+10-4i=0`
View SolutionOut of 14 marbles 10 are red in colour and remaining 4 are of different colours.How many ways can you select 10 marbles out of these 14 marbles?
View SolutionFind the sum to n terms :`1/2+3/2^2+5/2^3+...+(2n-1)/2^n`
View SolutionProve that the locus of the mid-points of chords of length 2d units of the hyperbola `xy=c^2`is `(x^2+y^2)(xy-c^2)=d^2xy`
View SolutionThe co-ordinates of end points of a focal chord of an ellipse are `(x_1,y_1)`and `(x_2,y_2)`.Prove that `y_1y_2+4x_1x_2=0`
View SolutionThe ellipse `x^2/a^2+y^2/b^2=1`passes through the point of intersection of the lines 7x+13y=87and 5x-8y+7=0and the length of its latus rectum is `(32sqrt2)/5`units.Find the values of a and b.
View Solution
EXERCISE questions and complete solutions for chapter ANNUAL EXAMINATION QUESTION PAPERS 2017 of UNITED BOOK HOUSE of Class 11